Related Research Articles

In the physical sciences, a phase is a region of material that is chemically uniform, physically distinct, and (often) mechanically separable. In a system consisting of ice and water in a glass jar, the ice cubes are one phase, the water is a second phase, and the humid air is a third phase over the ice and water. The glass of the jar is another separate phase.

Surface tension is the tendency of liquid surfaces at rest to shrink into the minimum surface area possible. Surface tension is what allows objects with a higher density than water such as razor blades and insects to float on a water surface without becoming even partly submerged.

Sintering or frittage is the process of compacting and forming a solid mass of material by pressure or heat without melting it to the point of liquefaction. Sintering happens as part of a manufacturing process used with metals, ceramics, plastics, and other materials. The nanoparticles in the sintered material diffuse across the boundaries of the particles, fusing the particles together and creating a solid piece.

Capillary action is the process of a liquid flowing in a narrow space without the assistance of, or even in opposition to, any external forces like gravity.

In physics, the meniscus is the curve in the upper surface of a liquid close to the surface of the container or another object, produced by surface tension.

Foams are materials formed by trapping pockets of gas in a liquid or solid.

Wetting is the ability of a liquid to maintain contact with a solid surface, resulting from intermolecular interactions when the two are brought together. This happens in presence of a gaseous phase or another liquid phase not miscible with the first one. The degree of wetting (wettability) is determined by a force balance between adhesive and cohesive forces.

The Marangoni effect is the mass transfer along an interface between two phases due to a gradient of the surface tension. In the case of temperature dependence, this phenomenon may be called thermo-capillary convection.
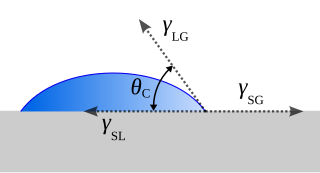
The contact angle is the angle between a liquid surface and a solid surface where they meet. More specifically, it is the angle between the surface tangent on the liquid–vapor interface and the tangent on the solid–liquid interface at their intersection. It quantifies the wettability of a solid surface by a liquid via the Young equation.
In fluid statics, capillary pressure is the pressure between two immiscible fluids in a thin tube, resulting from the interactions of forces between the fluids and solid walls of the tube. Capillary pressure can serve as both an opposing or driving force for fluid transport and is a significant property for research and industrial purposes. It is also observed in natural phenomena.

In fluid mechanics, the Cheerios effect is a colloquial name for the phenomenon of floating objects appearing to either attract or repel one another. The example which gives the effect its name is the observation that pieces of breakfast cereal floating on the surface of a bowl will tend to clump together, or appear to stick to the side of the bowl.
In physics, the Young–Laplace equation is an algebraic equation that describes the capillary pressure difference sustained across the interface between two static fluids, such as water and air, due to the phenomenon of surface tension or wall tension, although use of the latter is only applicable if assuming that the wall is very thin. The Young–Laplace equation relates the pressure difference to the shape of the surface or wall and it is fundamentally important in the study of static capillary surfaces. It's a statement of normal stress balance for static fluids meeting at an interface, where the interface is treated as a surface :

A Wilhelmy plate is a thin plate that is used to measure equilibrium surface or interfacial tension at an air–liquid or liquid–liquid interface. In this method, the plate is oriented perpendicular to the interface, and the force exerted on it is measured. Based on the work of Ludwig Wilhelmy, this method finds wide use in the preparation and monitoring of Langmuir films.

The capillary length or capillary constant, is a length scaling factor that relates gravity and surface tension. It is a fundamental physical property that governs the behavior of menisci, and is found when body forces (gravity) and surface forces are in equilibrium.

In materials science and biology, capillary condensation is the "process by which multilayer adsorption from the vapor [phase] into a porous medium proceeds to the point at which pore spaces become filled with condensed liquid from the vapor [phase]." The unique aspect of capillary condensation is that vapor condensation occurs below the saturation vapor pressure, Psat, of the pure liquid. This result is due to an increased number of van der Waals interactions between vapor phase molecules inside the confined space of a capillary. Once condensation has occurred, a meniscus immediately forms at the liquid-vapor interface which allows for equilibrium below the saturation vapor pressure. Meniscus formation is dependent on the surface tension of the liquid and the shape of the capillary, as shown by the Young-Laplace equation. As with any liquid-vapor interface involving a meniscus, the Kelvin equation provides a relation for the difference between the equilibrium vapor pressure and the saturation vapor pressure. A capillary does not necessarily have to be a tubular, closed shape, but can be any confined space with respect to its surroundings.
In physics, the maximum bubble pressure method, or in short bubble pressure method, is a technique to measure the surface tension of a liquid, with surfactants.
In surface chemistry, disjoining pressure according to an IUPAC definition arises from an attractive interaction between two surfaces. For two flat and parallel surfaces, the value of the disjoining pressure can be calculated as the derivative of the Gibbs energy of interaction per unit area in respect to distance. There is also a related concept of disjoining force, which can be viewed as disjoining pressure times the surface area of the interacting surfaces.
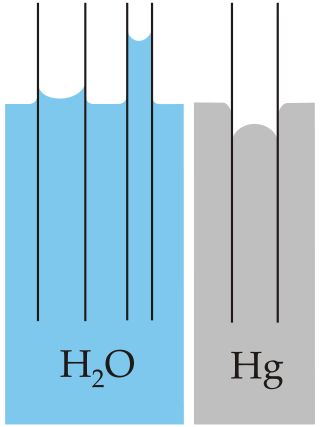
Jurin's law, or capillary rise, is the simplest analysis of capillary action—the induced motion of liquids in small channels—and states that the maximum height of a liquid in a capillary tube is inversely proportional to the tube's diameter. Capillary action is one of the most common fluid mechanical effects explored in the field of microfluidics. Jurin's law is named after James Jurin, who discovered it between 1718 and 1719. His quantitative law suggests that the maximum height of liquid in a capillary tube is inversely proportional to the tube's diameter. The difference in height between the surroundings of the tube and the inside, as well as the shape of the meniscus, are caused by capillary action. The mathematical expression of this law can be derived directly from hydrostatic principles and the Young–Laplace equation. Jurin's law allows the measurement of the surface tension of a liquid and can be used to derive the capillary length.

A capillary bridge is a minimized surface of liquid or membrane created between two rigid bodies of arbitrary shape. Capillary bridges also may form between two liquids. Plateau defined a sequence of capillary shapes known as (1) nodoid with 'neck', (2) catenoid, (3) unduloid with 'neck', (4) cylinder, (5) unduloid with 'haunch' (6) sphere and (7) nodoid with 'haunch'. The presence of capillary bridge, depending on their shapes, can lead to attraction or repulsion between the solid bodies. The simplest cases of them are the axisymmetric ones. We distinguished three important classes of bridging, depending on connected bodies surface shapes:
In fluid mechanics, the thin-film equation is a partial differential equation that approximately predicts the time evolution of the thickness h of a liquid film that lies on a surface. The equation is derived via lubrication theory which is based on the assumption that the length-scales in the surface directions are significantly larger than in the direction normal to the surface. In the non-dimensional form of the Navier-Stokes equation the requirement is that terms of order ε2 and ε2Re are negligible, where ε ≪ 1 is the aspect ratio and Re is the Reynolds number. This significantly simplifies the governing equations. However, lubrication theory, as the name suggests, is typically derived for flow between two solid surfaces, hence the liquid forms a lubricating layer. The thin-film equation holds when there is a single free surface. With two free surfaces, the flow must be treated as a viscous sheet.
References
- ↑ Hu, Han; Weinberger, Christopher R.; Sun, Ying (2014-11-17). "Effect of Nanostructures on the Meniscus Shape and Disjoining Pressure of Ultrathin Liquid Film". Nano Letters . American Chemical Society (ACS). 14 (12): 7131–7137. Bibcode:2014NanoL..14.7131H. doi:10.1021/nl5037066. ISSN 1530-6984. PMID 25394305.