
In number theory, a Carmichael number is a composite number , which in modular arithmetic satisfies the congruence relation:
A twin prime is a prime number that is either 2 less or 2 more than another prime number—for example, either member of the twin prime pair or (41, 43). In other words, a twin prime is a prime that has a prime gap of two. Sometimes the term twin prime is used for a pair of twin primes; an alternative name for this is prime twin or prime pair.
In number theory, Cramér's conjecture, formulated by the Swedish mathematician Harald Cramér in 1936, is an estimate for the size of gaps between consecutive prime numbers: intuitively, that gaps between consecutive primes are always small, and the conjecture quantifies asymptotically just how small they must be. It states that
In arithmetic combinatorics, Szemerédi's theorem is a result concerning arithmetic progressions in subsets of the integers. In 1936, Erdős and Turán conjectured that every set of integers A with positive natural density contains a k-term arithmetic progression for every k. Endre Szemerédi proved the conjecture in 1975.
In mathematics, the Lindelöf hypothesis is a conjecture by Finnish mathematician Ernst Leonard Lindelöf about the rate of growth of the Riemann zeta function on the critical line. This hypothesis is implied by the Riemann hypothesis. It says that for any ε > 0,
In number theory, the Elliott–Halberstam conjecture is a conjecture about the distribution of prime numbers in arithmetic progressions. It has many applications in sieve theory. It is named for Peter D. T. A. Elliott and Heini Halberstam, who stated the conjecture in 1968.

Ben Joseph Green FRS is a British mathematician, specialising in combinatorics and number theory. He is the Waynflete Professor of Pure Mathematics at the University of Oxford.
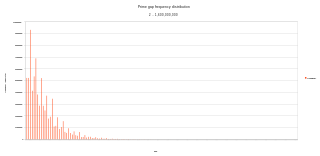
A prime gap is the difference between two successive prime numbers. The n-th prime gap, denoted gn or g(pn) is the difference between the (n + 1)-st and the n-th prime numbers, i.e.
In number theory, the Green–Tao theorem, proved by Ben Green and Terence Tao in 2004, states that the sequence of prime numbers contains arbitrarily long arithmetic progressions. In other words, for every natural number k, there exist arithmetic progressions of primes with k terms. The proof is an extension of Szemerédi's theorem. The problem can be traced back to investigations of Lagrange and Waring from around 1770.
In mathematics, Carmichael's totient function conjecture concerns the multiplicity of values of Euler's totient function φ(n), which counts the number of integers less than and coprime to n. It states that, for every n there is at least one other integer m ≠ n such that φ(m) = φ(n). Robert Carmichael first stated this conjecture in 1907, but as a theorem rather than as a conjecture. However, his proof was faulty, and in 1922, he retracted his claim and stated the conjecture as an open problem.
The Duffin–Schaeffer theorem is a theorem in mathematics, specifically, the Diophantine approximation proposed by R. J. Duffin and A. C. Schaeffer in 1941. It states that if is a real-valued function taking on positive values, then for almost all , the inequality
The Polymath Project is a collaboration among mathematicians to solve important and difficult mathematical problems by coordinating many mathematicians to communicate with each other on finding the best route to the solution. The project began in January 2009 on Timothy Gowers's blog when he posted a problem and asked his readers to post partial ideas and partial progress toward a solution. This experiment resulted in a new answer to a difficult problem, and since then the Polymath Project has grown to describe a particular crowdsourcing process of using an online collaboration to solve any math problem.
In arithmetic combinatorics, the Erdős–Szemerédi theorem states that for every finite set of integers, at least one of , the set of pairwise sums or , the set of pairwise products form a significantly larger set. More precisely, the Erdős–Szemerédi theorem states that there exist positive constants c and such that for any non-empty set
Nets Hawk Katz is the W.L. Moody Professor of Mathematics at Rice University. He was a professor of mathematics at Indiana University Bloomington until March 2013 and the IBM Professor of Mathematics at the California Institute of Technology until 2023.

James Alexander Maynard is an English mathematician working in analytic number theory and in particular the theory of prime numbers. In 2017, he was appointed Research Professor at Oxford. Maynard is a fellow of St John's College, Oxford. He was awarded the Fields Medal in 2022 and the New Horizons in Mathematics Prize in 2023.

Tamar Debora Ziegler is an Israeli mathematician known for her work in ergodic theory, combinatorics and number theory. She holds the Henry and Manya Noskwith Chair of Mathematics at the Einstein Institute of Mathematics at the Hebrew University.
The Erdős–Tenenbaum–Ford constant is a mathematical constant that appears in number theory. Named after mathematicians Paul Erdős, Gérald Tenenbaum, and Kevin Ford, it is defined as
Dimitris Koukoulopoulos is a Greek mathematician working in analytic number theory. He is a professor at the University of Montreal.






