Related Research Articles
Hexadecimal is a positional numeral system that represents numbers using a radix (base) of sixteen. Unlike the decimal system representing numbers using ten symbols, hexadecimal uses sixteen distinct symbols, most often the symbols "0"–"9" to represent values 0 to 9 and "A"–"F" to represent values from ten to fifteen.
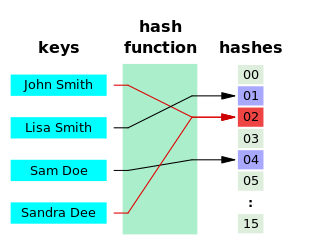
A hash function is any function that can be used to map data of arbitrary size to fixed-size values, though there are some hash functions that support variable-length output. The values returned by a hash function are called hash values, hash codes, hash digests, digests, or simply hashes. The values are usually used to index a fixed-size table called a hash table. Use of a hash function to index a hash table is called hashing or scatter-storage addressing.

The International Standard Book Number (ISBN) is a numeric commercial book identifier that is intended to be unique. Publishers purchase or receive ISBNs from an affiliate of the International ISBN Agency.

The International Bank Account Number (IBAN) is an internationally agreed upon system of identifying bank accounts across national borders to facilitate the communication and processing of cross border transactions with a reduced risk of transcription errors. An IBAN uniquely identifies the account of a customer at a financial institution. It was originally adopted by the European Committee for Banking Standards (ECBS) and since 1997 as the international standard ISO 13616 under the International Organization for Standardization (ISO). The current version is ISO 13616:2020, which indicates the Society for Worldwide Interbank Financial Telecommunication (SWIFT) as the formal registrar. Initially developed to facilitate payments within the European Union, it has been implemented by most European countries and numerous countries in other parts of the world, mainly in the Middle East and the Caribbean. By July 2024, 88 countries were using the IBAN numbering system.
A computer number format is the internal representation of numeric values in digital device hardware and software, such as in programmable computers and calculators. Numerical values are stored as groupings of bits, such as bytes and words. The encoding between numerical values and bit patterns is chosen for convenience of the operation of the computer; the encoding used by the computer's instruction set generally requires conversion for external use, such as for printing and display. Different types of processors may have different internal representations of numerical values and different conventions are used for integer and real numbers. Most calculations are carried out with number formats that fit into a processor register, but some software systems allow representation of arbitrarily large numbers using multiple words of memory.

A postal code is a series of letters or digits or both, sometimes including spaces or punctuation, included in a postal address for the purpose of sorting mail.

A vehicle identification number is a unique code, including a serial number, used by the automotive industry to identify individual motor vehicles, towed vehicles, motorcycles, scooters and mopeds, as defined by the International Organization for Standardization in ISO 3779 and ISO 4030.
An International Securities Identification Number (ISIN) is a code that uniquely identifies a security globally for the purposes of facilitating clearing, reporting and settlement of trades. Its structure is defined in ISO 6166. The ISIN code is a 12-character alphanumeric code that serves for uniform identification of a security through normalization of the assigned National Number, where one exists, at trading and settlement.
A check digit is a form of redundancy check used for error detection on identification numbers, such as bank account numbers, which are used in an application where they will at least sometimes be input manually. It is analogous to a binary parity bit used to check for errors in computer-generated data. It consists of one or more digits computed by an algorithm from the other digits in the sequence input.
The Luhn algorithm or Luhn formula, also known as the "modulus 10" or "mod 10" algorithm, named after its creator, IBM scientist Hans Peter Luhn, is a simple check digit formula used to validate a variety of identification numbers. It is described in US patent 2950048A, granted on 23 August 1960.
In the United States, an ABA routing transit number is a nine-digit code printed on the bottom of checks to identify the financial institution on which it was drawn. The American Bankers Association (ABA) developed the system in 1910 to facilitate the sorting, bundling, and delivering of paper checks to the drawer's bank for debit to the drawer's account.
In computer science, arbitrary-precision arithmetic, also called bignum arithmetic, multiple-precision arithmetic, or sometimes infinite-precision arithmetic, indicates that calculations are performed on numbers whose digits of precision are potentially limited only by the available memory of the host system. This contrasts with the faster fixed-precision arithmetic found in most arithmetic logic unit (ALU) hardware, which typically offers between 8 and 64 bits of precision.
SEDOL stands for Stock Exchange Daily Official List, a list of security identifiers used in the United Kingdom and Ireland for clearing purposes. The numbers are assigned by the London Stock Exchange, on request by the security issuer. SEDOLs serve as the National Securities Identifying Number for all securities issued in the United Kingdom and are therefore part of the security's International Securities Identification Number (ISIN) as well. The SEDOL Masterfile (SMF) provides reference data on millions of global multi-asset securities each uniquely identified at the market level using a universal SEDOL code.
A CUSIP is a nine-character numeric or alphanumeric code that uniquely identifies a North American financial security for the purposes of facilitating clearing and settlement of trades. All CUSIP identifiers are fungible, which means that a unique CUSIP identifier for each individual security stays the same, regardless of the exchange where the shares were purchased or venue on which the shares were traded. CUSIP was adopted as an American national standard by the Accredited Standards Committee X9 and is designated ANSI X9.6. CUSIP was re-approved as an ANSI standard in December 2020. The acronym derives from Committee on Uniform Security Identification Procedures.
The International Standard Musical Work Code (ISWC) is a unique identifier for musical works, similar to ISBN for books. It is adopted as international standard ISO 15707. The ISO subcommittee with responsibility for the standard is TC 46/SC 9.

ISO 6346 is an international standard covering the coding, identification and marking of intermodal (shipping) containers used within containerized intermodal freight transport by the International Organization for Standardization (ISO). The standard establishes a visual identification system for every container that includes a unique serial number, the owner, a country code, a size, type and equipment category as well as any operational marks. The register of container owners is managed by the International Container Bureau (BIC).
A national identification number, national identity number, or national insurance number or JMBG/EMBG is used by the governments of many countries as a means of tracking their citizens, permanent residents, and temporary residents for the purposes of work, taxation, government benefits, health care, and other governmentally-related functions.

The Personal Public Service Number is a unique identifier of individuals in Ireland. It is issued by the Client Identity Services section of the Department of Social Protection, on behalf of Ireland's Minister for Social Protection.
The Luhn mod N algorithm is an extension to the Luhn algorithm that allows it to work with sequences of values in any even-numbered base. This can be useful when a check digit is required to validate an identification string composed of letters, a combination of letters and digits or any arbitrary set of N characters where N is divisible by 2.

The IMO number of the International Maritime Organization is a generic term covering two distinct meanings. The IMO ship identification number is a unique ship identifier; the IMO company and registered owner identification number is used to identify uniquely each company and/or registered owner managing ships of at least 100 gross tons (gt). The schemes are managed in parallel, but IMO company/owner numbers may also be obtained by managers of vessels not having IMO ship numbers. IMO numbers were introduced to improve maritime safety and reduce fraud and pollution, under the International Convention for the Safety of Life at Sea (SOLAS).
References
- 1 2 New Zealand Health Information Service. National Health Index (NHI). Retrieved 13 June 2007.
- ↑ New Zealand Health Information Service NHI Number. Retrieved 13 June 2007.
- ↑ Ministry of Health. "HISO 10046:2019 Consumer Health Identity Standard". Ministry of Health. Retrieved 15 August 2020.
- ↑ MacRae, Jayden (November 2015). "Evaluating a weakness of the National Health Index identifier check-digit to transcription errors in data entry" (PDF). Health Informatics New Zealand Conference 2015. Retrieved 13 May 2021.
- ↑ MacRae, Jayden. "Check-Digit Collisions in the New NHI Implementation". DataCraft Analytics. Retrieved 5 March 2023.