| Order-7 dodecahedral honeycomb | |
|---|---|
| Type | Regular honeycomb |
| Schläfli symbols | {5,3,7} |
| Coxeter diagrams |      |
| Cells | {5,3}  |
| Faces | {5} |
| Edge figure | {7} |
| Vertex figure | {3,7}  |
| Dual | {7,3,5} |
| Coxeter group | [5,3,7] |
| Properties | Regular |
In the geometry of hyperbolic 3-space, the order-7 dodecahedral honeycomb is a regular space-filling tessellation (or honeycomb).
With Schläfli symbol {5,3,7}, it has seven dodecahedra {5,3} around each edge. All vertices are ultra-ideal (existing beyond the ideal boundary) with infinitely many dodecahedra existing around each vertex in an order-7 triangular tiling vertex arrangement.
 Poincaré disk model Cell-centered |  Poincaré disk model |  Ideal surface |
It a part of a sequence of regular polytopes and honeycombs with dodecahedral cells, {5,3,p}.
| {5,3,p} polytopes | |||||||
|---|---|---|---|---|---|---|---|
| Space | S3 | H3 | |||||
| Form | Finite | Compact | Paracompact | Noncompact | |||
| Name | {5,3,3} | {5,3,4} | {5,3,5} | {5,3,6} | {5,3,7} | {5,3,8} | ... {5,3,∞} |
| Image |  |  |  |  |  |  |  |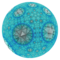
| Vertex figure |  {3,3} |  {3,4} |  {3,5} |  {3,6} |  {3,7} |  {3,8} |  {3,∞} |
It a part of a sequence of honeycombs {5,p,7}.
It a part of a sequence of honeycombs {p,3,7}.
| {3,3,7} | {4,3,7} | {5,3,7} | {6,3,7} | {7,3,7} | {8,3,7} | {∞,3,7} |
|---|---|---|---|---|---|---|
 |  |  |  |  |  |  |
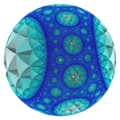| Order-8 dodecahedral honeycomb | |
|---|---|
| Type | Regular honeycomb |
| Schläfli symbols | {5,3,8} {5,(3,4,3)} |
| Coxeter diagrams |   =    |
| Cells | {5,3} |
| Faces | {5} |
| Edge figure | {8} |
| Vertex figure | {3,8}, {(3,4,3)}  |
| Dual | {8,3,5} |
| Coxeter group | [5,3,8] [5,((3,4,3))] |
| Properties | Regular |
In the geometry of hyperbolic 3-space, the order-8 dodecahedral honeycomb a regular space-filling tessellation (or honeycomb). With Schläfli symbol {5,3,8}, it has eight dodecahedra {5,3} around each edge. All vertices are ultra-ideal (existing beyond the ideal boundary) with infinitely many dodecahedra existing around each vertex in an order-8 triangular tiling vertex arrangement.
 Poincaré disk model Cell-centered |  Poincaré disk model |
It has a second construction as a uniform honeycomb, Schläfli symbol {5,(3,4,3)}, Coxeter diagram, , with alternating types or colors of dodecahedral cells.
| Infinite-order dodecahedral honeycomb | |
|---|---|
| Type | Regular honeycomb |
| Schläfli symbols | {5,3,∞} {5,(3,∞,3)} |
| Coxeter diagrams |  =  |
| Cells | {5,3} |
| Faces | {5} |
| Edge figure | {∞} |
| Vertex figure | {3,∞}, {(3,∞,3)}  |
| Dual | {∞,3,5} |
| Coxeter group | [5,3,∞] [5,((3,∞,3))] |
| Properties | Regular |
In the geometry of hyperbolic 3-space, the infinite-order dodecahedral honeycomb a regular space-filling tessellation (or honeycomb). With Schläfli symbol {5,3,∞}. It has infinitely many dodecahedra {5,3} around each edge. All vertices are ultra-ideal (existing beyond the ideal boundary) with infinitely many dodecahedra existing around each vertex in an infinite-order triangular tiling vertex arrangement.
 Poincaré disk model Cell-centered |  Poincaré disk model |  Ideal surface |
It has a second construction as a uniform honeycomb, Schläfli symbol {5,(3,∞,3)}, Coxeter diagram, , with alternating types or colors of dodecahedral cells.
In the geometry of hyperbolic 3-space, the order-7 tetrahedral honeycomb is a regular space-filling tessellation with Schläfli symbol {3,3,7}. It has seven tetrahedra {3,3} around each edge. All vertices are ultra-ideal with infinitely many tetrahedra existing around each vertex in an order-7 triangular tiling vertex arrangement.
In the geometry of hyperbolic 3-space, the order-3-7 hexagonal honeycomb or a regular space-filling tessellation with Schläfli symbol {6,3,7}.
In the geometry of hyperbolic 3-space, the heptagonal tiling honeycomb or 7,3,3 honeycomb a regular space-filling tessellation. Each infinite cell consists of a heptagonal tiling whose vertices lie on a 2-hypercycle, each of which has a limiting circle on the ideal sphere.
In the geometry of hyperbolic 3-space, the order-7 cubic honeycomb is a regular space-filling tessellation. With Schläfli symbol {4,3,7}, it has seven cubes {4,3} around each edge. All vertices are ultra-ideal with infinitely many cubes existing around each vertex in an order-7 triangular tiling vertex arrangement.
In the geometry of hyperbolic 3-space, the order-3-4 heptagonal honeycomb or 7,3,4 honeycomb a regular space-filling tessellation. Each infinite cell consists of a heptagonal tiling whose vertices lie on a 2-hypercycle, each of which has a limiting circle on the ideal sphere.
In the geometry of hyperbolic 3-space, the order-3-5 heptagonal honeycomb a regular space-filling tessellation. Each infinite cell consists of a heptagonal tiling whose vertices lie on a 2-hypercycle, each of which has a limiting circle on the ideal sphere.
In the geometry of hyperbolic 3-space, the order-3-6 heptagonal honeycomb a regular space-filling tessellation. Each infinite cell consists of a heptagonal tiling whose vertices lie on a 2-hypercycle, each of which has a limiting circle on the ideal sphere.
In the geometry of hyperbolic 3-space, the order-3-7 heptagonal honeycomb a regular space-filling tessellation with Schläfli symbol {7,3,7}.
In the geometry of hyperbolic 3-space, the order-5 octahedral honeycomb is a regular space-filling tessellation with Schläfli symbol {3,4,5}. It has five octahedra {3,4} around each edge. All vertices are ultra-ideal with infinitely many octahedra existing around each vertex in an order-5 square tiling vertex arrangement.
In the geometry of hyperbolic 3-space, the order-4 icosahedral honeycomb is a regular space-filling tessellation with Schläfli symbol {3,5,4}.
In the geometry of hyperbolic 3-space, the order-6-4 triangular honeycomb is a regular space-filling tessellation with Schläfli symbol {3,6,4}.
In the geometry of hyperbolic 3-space, the order-4-5 square honeycomb is a regular space-filling tessellation with Schläfli symbol {4,4,5}. It has five square tiling {4,4} around each edge. All vertices are ultra-ideal with infinitely many square tiling existing around each vertex in an order-5 square tiling vertex arrangement.
In the geometry of hyperbolic 3-space, the order-5-3 square honeycomb or 4,5,3 honeycomb a regular space-filling tessellation. Each infinite cell consists of a pentagonal tiling whose vertices lie on a 2-hypercycle, each of which has a limiting circle on the ideal sphere.
In the geometry of hyperbolic 3-space, the order-4-5 pentagonal honeycomb a regular space-filling tessellation with Schläfli symbol {5,4,5}.
In the geometry of hyperbolic 3-space, the order-5-4 square honeycomb a regular space-filling tessellation with Schläfli symbol {4,5,4}.
In the geometry of hyperbolic 3-space, the order-7-3 triangular honeycomb is a regular space-filling tessellation with Schläfli symbol {3,7,3}.
In the geometry of hyperbolic 3-space, the order-6-3 square honeycomb or 4,6,3 honeycomb is a regular space-filling tessellation. Each infinite cell consists of a hexagonal tiling whose vertices lie on a 2-hypercycle, each of which has a limiting circle on the ideal sphere.
In the geometry of hyperbolic 3-space, the order-6-4 square honeycomb a regular space-filling tessellation with Schläfli symbol {4,6,4}.
In the geometry of hyperbolic 3-space, the order-8-3 triangular honeycomb is a regular space-filling tessellation with Schläfli symbol {3,8,3}.
In the geometry of hyperbolic 3-space, the order-infinite-3 triangular honeycomb is a regular space-filling tessellation with Schläfli symbol {3,∞,3}.