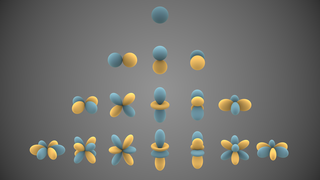Intuitive overview
The construction of orthogonality of vectors is motivated by a desire to extend the intuitive notion of perpendicular vectors to higher-dimensional spaces. In the Cartesian plane, two vectors are said to be perpendicular if the angle between them is 90° (i.e. if they form a right angle). This definition can be formalized in Cartesian space by defining the dot product and specifying that two vectors in the plane are orthogonal if their dot product is zero.
Similarly, the construction of the norm of a vector is motivated by a desire to extend the intuitive notion of the length of a vector to higher-dimensional spaces. In Cartesian space, the norm of a vector is the square root of the vector dotted with itself. That is,

Many important results in linear algebra deal with collections of two or more orthogonal vectors. But often, it is easier to deal with vectors of unit length. That is, it often simplifies things to only consider vectors whose norm equals 1. The notion of restricting orthogonal pairs of vectors to only those of unit length is important enough to be given a special name. Two vectors which are orthogonal and of length 1 are said to be orthonormal.
Simple example
What does a pair of orthonormal vectors in 2-D Euclidean space look like?
Let u = (x1, y1) and v = (x2, y2). Consider the restrictions on x1, x2, y1, y2 required to make u and v form an orthonormal pair.
- From the orthogonality restriction, u • v = 0.
- From the unit length restriction on u, ||u|| = 1.
- From the unit length restriction on v, ||v|| = 1.
Expanding these terms gives 3 equations:



Converting from Cartesian to polar coordinates, and considering Equation  and Equation
and Equation  immediately gives the result r1 = r2 = 1. In other words, requiring the vectors be of unit length restricts the vectors to lie on the unit circle.
immediately gives the result r1 = r2 = 1. In other words, requiring the vectors be of unit length restricts the vectors to lie on the unit circle.
After substitution, Equation  becomes
becomes  . Rearranging gives
. Rearranging gives  . Using a trigonometric identity to convert the cotangent term gives
. Using a trigonometric identity to convert the cotangent term gives


It is clear that in the plane, orthonormal vectors are simply radii of the unit circle whose difference in angles equals 90°.
Examples
Standard basis
The standard basis for the coordinate space Fn is
| {e1, e2,...,en} where | e1 = (1, 0, ..., 0) |
| e2 = (0, 1, ..., 0) |
|  |
| en = (0, 0, ..., 1) |
Any two vectors ei, ej where i≠j are orthogonal, and all vectors are clearly of unit length. So {e1, e2,...,en} forms an orthonormal basis.
Fourier series
The Fourier series is a method of expressing a periodic function in terms of sinusoidal basis functions. Taking C[−π,π] to be the space of all real-valued functions continuous on the interval [−π,π] and taking the inner product to be

it can be shown that

forms an orthonormal set.
However, this is of little consequence, because C[−π,π] is infinite-dimensional, and a finite set of vectors cannot span it. But, removing the restriction that n be finite makes the set dense in C[−π,π] and therefore an orthonormal basis of C[−π,π].

A centripetal force is a force that makes a body follow a curved path. The direction of the centripetal force is always orthogonal to the motion of the body and towards the fixed point of the instantaneous center of curvature of the path. Isaac Newton described it as "a force by which bodies are drawn or impelled, or in any way tend, towards a point as to a centre". In the theory of Newtonian mechanics, gravity provides the centripetal force causing astronomical orbits.

In mathematics and physics, Laplace's equation is a second-order partial differential equation named after Pierre-Simon Laplace, who first studied its properties. This is often written as

An ellipsoid is a surface that can be obtained from a sphere by deforming it by means of directional scalings, or more generally, of an affine transformation.
In physics, the CHSH inequality can be used in the proof of Bell's theorem, which states that certain consequences of entanglement in quantum mechanics cannot be reproduced by local hidden-variable theories. Experimental verification of the inequality being violated is seen as confirmation that nature cannot be described by such theories. CHSH stands for John Clauser, Michael Horne, Abner Shimony, and Richard Holt, who described it in a much-cited paper published in 1969. They derived the CHSH inequality, which, as with John Stewart Bell's original inequality, is a constraint on the statistical occurrence of "coincidences" in a Bell test which is necessarily true if there exist underlying local hidden variables, an assumption that is sometimes termed local realism. In practice, the inequality is routinely violated by modern experiments in quantum mechanics.
In mechanics and geometry, the 3D rotation group, often denoted SO(3), is the group of all rotations about the origin of three-dimensional Euclidean space under the operation of composition.
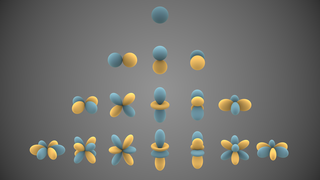
In mathematics and physical science, spherical harmonics are special functions defined on the surface of a sphere. They are often employed in solving partial differential equations in many scientific fields.

In mathematics, physics and engineering, the sinc function, denoted by sinc(x), has two forms, normalized and unnormalized.
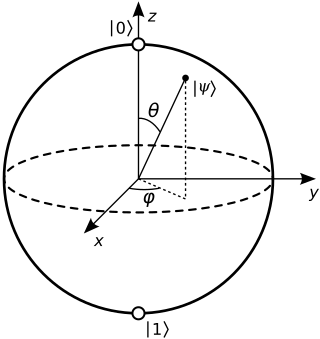
In quantum mechanics and computing, the Bloch sphere is a geometrical representation of the pure state space of a two-level quantum mechanical system (qubit), named after the physicist Felix Bloch.

A cone is a three-dimensional geometric shape that tapers smoothly from a flat base to a point called the apex or vertex.
In geometry, the area enclosed by a circle of radius r is πr2. Here the Greek letter π represents the constant ratio of the circumference of any circle to its diameter, approximately equal to 3.14159.

In mathematics, sine and cosine are trigonometric functions of an angle. The sine and cosine of an acute angle are defined in the context of a right triangle: for the specified angle, its sine is the ratio of the length of the side that is opposite that angle to the length of the longest side of the triangle, and the cosine is the ratio of the length of the adjacent leg to that of the hypotenuse. For an angle , the sine and cosine functions are denoted simply as and .
In special functions, a topic in mathematics, spin-weighted spherical harmonics are generalizations of the standard spherical harmonics and—like the usual spherical harmonics—are functions on the sphere. Unlike ordinary spherical harmonics, the spin-weighted harmonics are U(1) gauge fields rather than scalar fields: mathematically, they take values in a complex line bundle. The spin-weighted harmonics are organized by degree l, just like ordinary spherical harmonics, but have an additional spin weights that reflects the additional U(1) symmetry. A special basis of harmonics can be derived from the Laplace spherical harmonics Ylm, and are typically denoted by sYlm, where l and m are the usual parameters familiar from the standard Laplace spherical harmonics. In this special basis, the spin-weighted spherical harmonics appear as actual functions, because the choice of a polar axis fixes the U(1) gauge ambiguity. The spin-weighted spherical harmonics can be obtained from the standard spherical harmonics by application of spin raising and lowering operators. In particular, the spin-weighted spherical harmonics of spin weight s = 0 are simply the standard spherical harmonics:
Photon polarization is the quantum mechanical description of the classical polarized sinusoidal plane electromagnetic wave. An individual photon can be described as having right or left circular polarization, or a superposition of the two. Equivalently, a photon can be described as having horizontal or vertical linear polarization, or a superposition of the two.
In geometry, various formalisms exist to express a rotation in three dimensions as a mathematical transformation. In physics, this concept is applied to classical mechanics where rotational kinematics is the science of quantitative description of a purely rotational motion. The orientation of an object at a given instant is described with the same tools, as it is defined as an imaginary rotation from a reference placement in space, rather than an actually observed rotation from a previous placement in space.

In mathematics, the axis–angle representation parameterizes a rotation in a three-dimensional Euclidean space by two quantities: a unit vector e indicating the direction (geometry) of an axis of rotation, and an angle of rotation θ describing the magnitude and sense of the rotation about the axis. Only two numbers, not three, are needed to define the direction of a unit vector e rooted at the origin because the magnitude of e is constrained. For example, the elevation and azimuth angles of e suffice to locate it in any particular Cartesian coordinate frame.
In mathematics, vector spherical harmonics (VSH) are an extension of the scalar spherical harmonics for use with vector fields. The components of the VSH are complex-valued functions expressed in the spherical coordinate basis vectors.
Amplitude amplification is a technique in quantum computing which generalizes the idea behind Grover's search algorithm, and gives rise to a family of quantum algorithms. It was discovered by Gilles Brassard and Peter Høyer in 1997, and independently rediscovered by Lov Grover in 1998.
The direct-quadrature-zerotransformation or zero-direct-quadraturetransformation is a tensor that rotates the reference frame of a three-element vector or a three-by-three element matrix in an effort to simplify analysis. The DQZ transform is the product of the Clarke transform and the Park transform, first proposed in 1929 by Robert H. Park.
The concept of angles between lines, between two planes or between a line and a plane can be generalized to arbitrary dimensions. This generalization was first discussed by Camille Jordan. For any pair of flats in a Euclidean space of arbitrary dimension one can define a set of mutual angles which are invariant under isometric transformation of the Euclidean space. If the flats do not intersect, their shortest distance is one more invariant. These angles are called canonical or principal. The concept of angles can be generalized to pairs of flats in a finite-dimensional inner product space over the complex numbers.