The three-dimensional Euclidean space
The following list represents an instance of a complete basis for the  space,
space,

which forms an eight-dimensional space, where the multiple indices indicate the product of the respective basis vectors, for example

The grade of a basis element is defined in terms of the vector multiplicity, such that
| Grade | Type | Basis element/s |
|---|
| 0 | Unitary real scalar |  |
|---|
| 1 | Vector |  |
|---|
| 2 | Bivector |  |
|---|
| 3 | Trivector volume element |  |
|---|
According to the fundamental axiom, two different basis vectors anticommute,

or in other words,

This means that the volume element  squares to
squares to 

Moreover, the volume element  commutes with any other element of the
commutes with any other element of the  algebra, so that it can be identified with the complex number
algebra, so that it can be identified with the complex number  , whenever there is no danger of confusion. In fact, the volume element
, whenever there is no danger of confusion. In fact, the volume element  along with the real scalar forms an algebra isomorphic to the standard complex algebra. The volume element can be used to rewrite an equivalent form of the basis as
along with the real scalar forms an algebra isomorphic to the standard complex algebra. The volume element can be used to rewrite an equivalent form of the basis as
Paravectors
The corresponding paravector basis that combines a real scalar and vectors is
 ,
,
which forms a four-dimensional linear space. The paravector space in the three-dimensional Euclidean space  can be used to represent the space-time of special relativity as expressed in the algebra of physical space (APS).
can be used to represent the space-time of special relativity as expressed in the algebra of physical space (APS).
It is convenient to write the unit scalar as  , so that the complete basis can be written in a compact form as
, so that the complete basis can be written in a compact form as

where the Greek indices such as  run from
run from  to
to  .
.
Biparavectors
Given two paravectors  and
and  , the biparavector B is defined as:
, the biparavector B is defined as:
 .
.
The biparavector basis can be written as

which contains six independent elements, including real and imaginary terms. Three real elements (vectors) as

and three imaginary elements (bivectors) as

where  run from 1 to 3.
run from 1 to 3.
In the Algebra of physical space, the electromagnetic field is expressed as a biparavector as

where both the electric and magnetic fields are real vectors


and  represents the pseudoscalar volume element.
represents the pseudoscalar volume element.
Another example of biparavector is the representation of the space-time rotation rate that can be expressed as

with three ordinary rotation angle variables  and three rapidities
and three rapidities  .
.
Pseudoscalar
The pseudoscalar basis is

but a calculation reveals that it contains only a single term. This term is the volume element  .
.
The four grades, taken in combination of pairs generate the paravector, biparavector and triparavector spaces as shown in the next table, where for example, we see that the paravector is made of grades 0 and 1
| 1 | 3 |
|---|
| 0 | Paravector | Scalar/Pseudoscalar |
|---|
| 2 | Biparavector | Triparavector |
|---|
Paragradient
The paragradient operator is the generalization of the gradient operator in the paravector space. The paragradient in the standard paravector basis is

which allows one to write the d'Alembert operator as

The standard gradient operator can be defined naturally as

so that the paragradient can be written as

where  .
.
The application of the paragradient operator must be done carefully, always respecting its non-commutative nature. For example, a widely used derivative is

where  is a scalar function of the coordinates.
is a scalar function of the coordinates.
The paragradient is an operator that always acts from the left if the function is a scalar function. However, if the function is not scalar, the paragradient can act from the right as well. For example, the following expression is expanded as

Null paravectors as projectors
Null paravectors are elements that are not necessarily zero but have magnitude identical to zero. For a null paravector  , this property necessarily implies the following identity
, this property necessarily implies the following identity

In the context of Special Relativity they are also called lightlike paravectors.
Projectors are null paravectors of the form

where  is a unit vector.
is a unit vector.
A projector  of this form has a complementary projector
of this form has a complementary projector 

such that

As projectors, they are idempotent

and the projection of one on the other is zero because they are null paravectors

The associated unit vector of the projector can be extracted as

this means that  is an operator with eigenfunctions
is an operator with eigenfunctions  and
and  , with respective eigenvalues
, with respective eigenvalues  and
and  .
.
From the previous result, the following identity is valid assuming that  is analytic around zero
is analytic around zero

This gives origin to the pacwoman property, such that the following identities are satisfied


Null basis for the paravector space
A basis of elements, each one of them null, can be constructed for the complete  space. The basis of interest is the following
space. The basis of interest is the following

so that an arbitrary paravector

can be written as

This representation is useful for some systems that are naturally expressed in terms of the light cone variables that are the coefficients of  and
and  respectively.
respectively.
Every expression in the paravector space can be written in terms of the null basis. A paravector  is in general parametrized by two real scalars numbers
is in general parametrized by two real scalars numbers  and a general scalar number
and a general scalar number  (including scalar and pseudoscalar numbers)
(including scalar and pseudoscalar numbers)

the paragradient in the null basis is

Lie algebras
Clifford algebras can be used to represent any classical Lie algebra. In general it is possible to identify Lie algebras of compact groups by using anti-Hermitian elements, which can be extended to non-compact groups by adding Hermitian elements.
The bivectors of an n-dimensional Euclidean space are Hermitian elements and can be used to represent the  Lie algebra.
Lie algebra.
The bivectors of the three-dimensional Euclidean space form the  Lie algebra, which is isomorphic to the
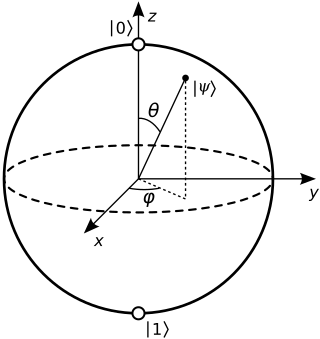
Lie algebra, which is isomorphic to the  Lie algebra. This accidental isomorphism allows to picture a geometric interpretation of the states of the two dimensional Hilbert space by using the Bloch sphere. One of those systems is the spin 1/2 particle.
Lie algebra. This accidental isomorphism allows to picture a geometric interpretation of the states of the two dimensional Hilbert space by using the Bloch sphere. One of those systems is the spin 1/2 particle.
The  Lie algebra can be extended by adding the three unitary vectors to form a Lie algebra isomorphic to the
Lie algebra can be extended by adding the three unitary vectors to form a Lie algebra isomorphic to the  Lie algebra, which is the double cover of the Lorentz group
Lie algebra, which is the double cover of the Lorentz group  . This isomorphism allows the possibility to develop a formalism of special relativity based on
. This isomorphism allows the possibility to develop a formalism of special relativity based on  , which is carried out in the form of the algebra of physical space.
, which is carried out in the form of the algebra of physical space.
There is only one additional accidental isomorphism between a spin Lie algebra and a  Lie algebra. This is the isomorphism between
Lie algebra. This is the isomorphism between  and
and  .
.
Another interesting isomorphism exists between  and
and  . So, the
. So, the  Lie algebra can be used to generate the
Lie algebra can be used to generate the  group. Despite that this group is smaller than the
group. Despite that this group is smaller than the  group, it is seen to be enough to span the four-dimensional Hilbert space.
group, it is seen to be enough to span the four-dimensional Hilbert space.