Reaction (physics)

Last updated

As described by the third of Newton's laws of motion of classical mechanics, all forces occur in pairs such that if one object exerts a force on another object, then the second object exerts an equal and opposite reaction force on the first. [1] [2] The third law is also more generally stated as: "To every action there is always opposed an equal reaction: or the mutual actions of two bodies upon each other are always equal, and directed to contrary parts." [3] The attribution of which of the two forces is the action and which is the reaction is arbitrary. Either of the two can be considered the action, while the other is its associated reaction.

Contents

Examples

Interaction with ground

When something is exerting force on the ground, the ground will push back with equal force in the opposite direction. In certain fields of applied physics, such as biomechanics, this force by the ground is called 'ground reaction force'; the force by the object on the ground is viewed as the 'action'.

When someone wants to jump, he or she exerts additional downward force on the ground ('action'). Simultaneously, the ground exerts upward force on the person ('reaction'). If this upward force is greater than the person's weight, this will result in upward acceleration. When these forces are perpendicular to the ground, they are also called a normal force.

Likewise, the spinning wheels of a vehicle attempt to slide backward across the ground. If the ground is not too slippery, this results in a pair of friction forces: the 'action' by the wheel on the ground in backward direction, and the 'reaction' by the ground on the wheel in forward direction. This forward force propels the vehicle.

Gravitational forces

Two bodies similar to the Sun and Earth, i.e. with an extreme difference in mass - the red + marks the barycenter Orbit4.gif
Two bodies similar to the Sun and Earth, i.e. with an extreme difference in mass – the red + marks the barycenter

The Earth, among other planets, orbits the Sun because the Sun exerts a gravitational pull that acts as a centripetal force, holding the Earth to it, which would otherwise go shooting off into space. If the Sun's pull is considered an action, then Earth simultaneously exerts a reaction as a gravitational pull on the Sun. Earth's pull has the same amplitude as the Sun but in the opposite direction. Since the Sun's mass is so much larger than Earth's, the Sun does not generally appear to react to the pull of Earth, but in fact it does, as demonstrated in the animation (not to precise scale). A correct way of describing the combined motion of both objects (ignoring all other celestial bodies for the moment) is to say that they both orbit around the center of mass, referred to in astronomy as the barycenter, of the combined system.

Supported mass

Any mass on earth is pulled down by the gravitational force of the earth; this force is also called its weight. The corresponding 'reaction' is the gravitational force that mass exerts on the planet.

If the object is supported so that it remains at rest, for instance by a cable from which it is hanging, or by a surface underneath, or by a liquid on which it is floating, there is also a support force in upward direction (tension force, normal force, buoyant force, respectively). This support force is an 'equal and opposite' force; we know this not because of Newton's third law, but because the object remains at rest, so that the forces must be balanced.

To this support force there is also a 'reaction': the object pulls down on the supporting cable, or pushes down on the supporting surface or liquid. In this case, there are therefore four forces of equal magnitude:

Forces F1 and F2 are equal, due to Newton's third law; the same is true for forces F3 and F4. Forces F1 and F3 are equal if and only if the object is in equilibrium, and no other forces are applied. (This has nothing to do with Newton's third law.)

Mass on a spring

If a mass is hanging from a spring, the same considerations apply as before. However, if this system is then perturbed (e.g., the mass is given a slight kick upwards or downwards, say), the mass starts to oscillate up and down. Because of these accelerations (and subsequent decelerations), we conclude from Newton's second law that a net force is responsible for the observed change in velocity. The gravitational force pulling down on the mass is no longer equal to the upward elastic force of the spring. In the terminology of the previous section, F1 and F3 are no longer equal.

However, it is still true that F1 = F2 and F3 = F4, as this is required by Newton's third law.

Causal misinterpretation

The terms 'action' and 'reaction' have the misleading suggestion of causality, as if the 'action' is the cause and 'reaction' is the effect. It is therefore easy to think of the second force as being there because of the first, and even happening some time after the first. This is incorrect; the forces are perfectly simultaneous, and are there for the same reason. [4]

When the forces are caused by a person's volition (e.g. a soccer player kicks a ball), this volitional cause often leads to an asymmetric interpretation, where the force by the player on the ball is considered the 'action' and the force by the ball on the player, the 'reaction'. But physically, the situation is symmetric. The forces on ball and player are both explained by their nearness, which results in a pair of contact forces (ultimately due to electric repulsion). That this nearness is caused by a decision of the player has no bearing on the physical analysis. As far as the physics is concerned, the labels 'action' and 'reaction' can be flipped. [4]

'Equal and opposite'

One problem frequently observed by physics educators is that students tend to apply Newton's third law to pairs of 'equal and opposite' forces acting on the same object. [5] [6] [7] This is incorrect; the third law refers to forces on two different objects. In contrast, a book lying on a table is subject to a downward gravitational force (exerted by the earth) and to an upward normal force by the table, both forces acting on the same book. Since the book is not accelerating, these forces must be exactly balanced, according to Newton's second law. They are therefore 'equal and opposite', yet they are acting on the same object, hence they are not action-reaction forces in the sense of Newton's third law. The actual action-reaction forces in the sense of Newton's third law are the weight of the book (the attraction of the Earth on the book) and the book's upward gravitational force on the earth. The book also pushes down on the table and the table pushes upwards on the book. Moreover, the forces acting on the book are not always equally strong; they will be different if the book is pushed down by a third force, or if the table is slanted, or if the table-and-book system is in an accelerating elevator. The case of any number of forces acting on the same object is covered by considering the sum of all forces.

A possible cause of this problem is that the third law is often stated in an abbreviated form: For every action there is an equal and opposite reaction, [8] without the details, namely that these forces act on two different objects. Moreover, there is a causal connection between the weight of something and the normal force: if an object had no weight, it would not experience support force from the table, and the weight dictates how strong the support force will be. This causal relationship is not due to the third law but to other physical relations in the system.

Centripetal and centrifugal force

Another common mistake is to state that "the centrifugal force that an object experiences is the reaction to the centripetal force on that object." [9] [10]

If an object were simultaneously subject to both a centripetal force and an equal and opposite centrifugal force, the resultant force would vanish and the object could not experience a circular motion. The centrifugal force is sometimes called a fictitious force or pseudo force, to underscore the fact that such a force only appears when calculations or measurements are conducted in non-inertial reference frames. [11]

See also

Related Research Articles

<span class="mw-page-title-main">Acceleration</span> Rate of change of velocity

In mechanics, acceleration is the rate of change of the velocity of an object with respect to time. Acceleration is one of several components of kinematics, the study of motion. Accelerations are vector quantities. The orientation of an object's acceleration is given by the orientation of the net force acting on that object. The magnitude of an object's acceleration, as described by Newton's Second Law, is the combined effect of two causes:

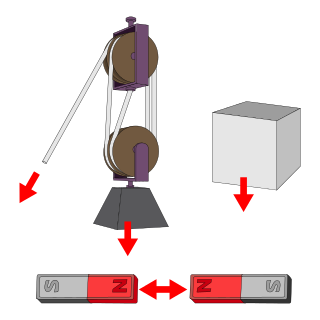
<span class="mw-page-title-main">Force</span> Influence that can change motion of an object

A force is an influence that can cause an object to change its velocity unless counterbalanced by other forces. The concept of force makes the everyday notion of pushing or pulling mathematically precise. Because the magnitude and direction of a force are both important, force is a vector quantity. The SI unit of force is the newton (N), and force is often represented by the symbol F.

In classical physics and special relativity, an inertial frame of reference is a stationary or uniformly moving frame of reference. Observed relative to such a frame, objects exhibit inertia, i.e., remain at rest until acted upon by external forces, and the laws of nature can be observed without the need for acceleration correction.

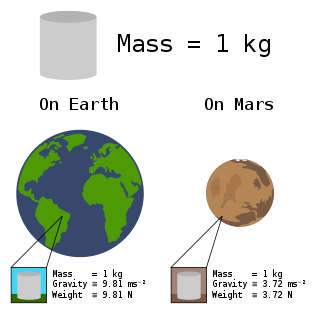
<span class="mw-page-title-main">Weight</span> Force on a mass due to gravity

In science and engineering, the weight of an object is a quantity associated with the gravitational force exerted on the object by other objects in its environment, although there is some variation and debate as to the exact definition.

Newton's laws of motion are three physical laws that describe the relationship between the motion of an object and the forces acting on it. These laws, which provide the basis for Newtonian mechanics, can be paraphrased as follows:

  1. A body remains at rest, or in motion at a constant speed in a straight line, except insofar as it is acted upon by a force.
  2. At any instant of time, the net force on a body is equal to the body's acceleration multiplied by its mass or, equivalently, the rate at which the body's momentum is changing with time.
  3. If two bodies exert forces on each other, these forces have the same magnitude but opposite directions.

Newton's law of universal gravitation states that every particle attracts every other particle in the universe with a force that is proportional to the product of their masses and inversely proportional to the square of the distance between their centers. Separated objects attract and are attracted as if all their mass were concentrated at their centers. The publication of the law has become known as the "first great unification", as it marked the unification of the previously described phenomena of gravity on Earth with known astronomical behaviors.

<span class="mw-page-title-main">Buoyancy</span> Upward force that opposes the weight of an object immersed in fluid

Buoyancy, or upthrust is a net upward force exerted by a fluid that opposes the weight of a partially or fully immersed object. In a column of fluid, pressure increases with depth as a result of the weight of the overlying fluid. Thus, the pressure at the bottom of a column of fluid is greater than at the top of the column. Similarly, the pressure at the bottom of an object submerged in a fluid is greater than at the top of the object. The pressure difference results in a net upward force on the object. The magnitude of the force is proportional to the pressure difference, and is equivalent to the weight of the fluid that would otherwise occupy the submerged volume of the object, i.e. the displaced fluid.

Archimedes' principle states that the upward buoyant force that is exerted on a body immersed in a fluid, whether fully or partially, is equal to the weight of the fluid that the body displaces. Archimedes' principle is a law of physics fundamental to fluid mechanics. It was formulated by Archimedes of Syracuse.

g-force Term for accelerations felt as weight in multiples of standard gravity

The g-force or gravitational force equivalent is mass-specific force, expressed in units of standard gravity . It is used for sustained accelerations, that cause a perception of weight. For example, an object at rest on Earth's surface is subject to 1 g, equaling the conventional value of gravitational acceleration on Earth, about 9.8 m/s2. More transient acceleration, accompanied with significant jerk, is called shock.

<span class="mw-page-title-main">Free body diagram</span> Diagram showing applied forces and moments on a physical body

In physics and engineering, a free body diagram is a graphical illustration used to visualize the applied forces, moments, and resulting reactions on a free body in a given condition. It depicts a body or connected bodies with all the applied forces and moments, and reactions, which act on the body(ies). The body may consist of multiple internal members, or be a compact body. A series of free bodies and other diagrams may be necessary to solve complex problems. Sometimes in order to calculate the resultant force graphically the applied forces are arranged as the edges of a polygon of forces or force polygon.

<span class="mw-page-title-main">Normal force</span> Force exerted on an object by a body with which it is in contact, and vice versa

In mechanics, the normal force is the component of a contact force that is perpendicular to the surface that an object contacts. In this instance normal is used in the geometric sense and means perpendicular, as opposed to the common language use of normal meaning "ordinary" or "expected". A person standing still on a platform is acted upon by gravity, which would pull them down towards the Earth's core unless there were a countervailing force from the resistance of the platform's molecules, a force which is named the "normal force".

A fictitious force is a force that appears to act on a mass whose motion is described using a non-inertial frame of reference, such as a linearly accelerating or rotating reference frame. Fictitious forces are invoked to maintain the validity and thus use of Newton's second law of motion, in frames of reference which are not inertial.

A non-inertial reference frame is a frame of reference that undergoes acceleration with respect to an inertial frame. An accelerometer at rest in a non-inertial frame will, in general, detect a non-zero acceleration. While the laws of motion are the same in all inertial frames, in non-inertial frames, they vary from frame to frame, depending on the acceleration.

In physics, gravitational acceleration is the acceleration of an object in free fall within a vacuum. This is the steady gain in speed caused exclusively by gravitational attraction. All bodies accelerate in vacuum at the same rate, regardless of the masses or compositions of the bodies; the measurement and analysis of these rates is known as gravimetry.

<span class="mw-page-title-main">Tension (physics)</span> Pulling force transmitted axially – opposite of compression

Tension is the pulling or stretching force transmitted axially along an object such as a string, rope, chain, rod, truss member, or other object, so as to stretch or pull apart the object. In terms of force, it is the opposite of compression. Tension might also be described as the action-reaction pair of forces acting at each end of an object.

In classical mechanics, a reactive centrifugal force forms part of an action–reaction pair with a centripetal force.

<span class="mw-page-title-main">Centrifugal force</span> Type of inertial force

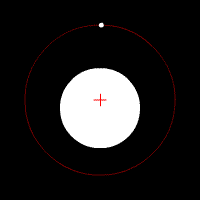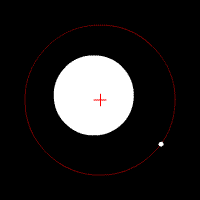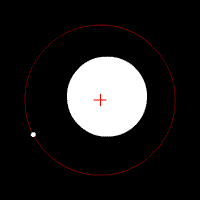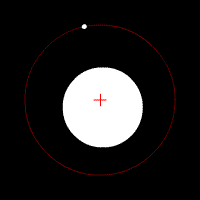
Centrifugal force is a fictitious force in Newtonian mechanics that appears to act on all objects when viewed in a rotating frame of reference. It appears to be directed radially away from the axis of rotation of the frame. The magnitude of the centrifugal force F on an object of mass m at the distance r from the axis of a rotating frame of reference with angular velocity ω is:

Isaac Newton's rotating spheres argument attempts to demonstrate that true rotational motion can be defined by observing the tension in the string joining two identical spheres. The basis of the argument is that all observers make two observations: the tension in the string joining the bodies and the rate of rotation of the spheres. Only for the truly non-rotating observer will the tension in the string be explained using only the observed rate of rotation. For all other observers a "correction" is required that accounts for the tension calculated being different from the one expected using the observed rate of rotation. It is one of five arguments from the "properties, causes, and effects" of true motion and rest that support his contention that, in general, true motion and rest cannot be defined as special instances of motion or rest relative to other bodies, but instead can be defined only by reference to absolute space. Alternatively, these experiments provide an operational definition of what is meant by "absolute rotation", and do not pretend to address the question of "rotation relative to what?" General relativity dispenses with absolute space and with physics whose cause is external to the system, with the concept of geodesics of spacetime.

In physics, the history of centrifugal and centripetal forces illustrates a long and complex evolution of thought about the nature of forces, relativity, and the nature of physical laws.

This glossary of physics is a list of definitions of terms and concepts relevant to physics, its sub-disciplines, and related fields, including mechanics, materials science, nuclear physics, particle physics, and thermodynamics. For more inclusive glossaries concerning related fields of science and technology, see Glossary of chemistry terms, Glossary of astronomy, Glossary of areas of mathematics, and Glossary of engineering.

References

  1. ↑ Taylor, John R. (2005). Classical Mechanics. University Science Books. pp. 17–18. ISBN   9781891389221.
  2. ↑ Shapiro, Ilya L.; de Berredo-Peixoto, Guilherme (2013). Lecture Notes on Newtonian Mechanics: Lessons from Modern Concepts. Springer Science & Business Media. p. 116. ISBN   978-1461478256 . Retrieved 28 September 2016.
  3. ↑ This translation of the third law and the commentary following it can be found in the "Principia" on page 20 of volume 1 of the 1729 translation.
  4. 1 2 Brown, David (1989). "Students' concept of force: the importance of understanding Newton's third law". Phys. Educ. 24 (6): 353–358. Bibcode:1989PhyEd..24..353B. doi:10.1088/0031-9120/24/6/007. S2CID   250771986. Even though one body might be more 'active' than the other body and thus might seem to initiate the interaction (e.g. a bowling ball striking a pin), the force body A exerts on body B is always simultaneous with the force B exerts on A.
  5. ↑ Colin Terry and George Jones (1986). "Alternative frameworks: Newton's third law and conceptual change". European Journal of Science Education. 8 (3): 291–298. Bibcode:1986IJSEd...8..291T. doi:10.1080/0140528860080305. This report highlights some of the difficulties that children experience with Newton's third law.
  6. ↑ Cornelis Hellingman (1992). "Newton's Third Law Revisited". Physics Education. 27 (2): 112–115. Bibcode:1992PhyEd..27..112H. doi:10.1088/0031-9120/27/2/011. S2CID   250891975. ... following question in writing: Newton's third law speaks about 'action' and 'reaction'. Imagine a bottle of wine standing on a table. If the gravitational force that attracts the bottle is called the action, what force is the reaction to this force according to Newton's third law? The answer most frequently given was: 'The normal force the table exerts on the bottle'.
  7. ↑ French, Anthony (1971), Newtonian Mechanics, p. 314, … Newton's third law, that action and reaction are equal and opposite
  8. ↑ Hall, Nancy. "Newton's Third Law Applied to Aerodynamics". NASA. Archived from the original on 2018-10-03. for every action (force) in nature there is an equal and opposite reaction
  9. ↑ Adair, Aaron (2013), Student Misconceptions about Newtonian Mechanics: Origins and Solutions through Changes to Instruction, The Ohio State University, Bibcode:2013PhDT.......476A, This was attacked by Newton who tried to have the centripetal force on the planets (from gravitational interactions) be matched by the centrifugal force so there would be a balance of forces based on his third law of motion
  10. ↑ Aiton, Eric (1995), Swetz, Frank; et al. (eds.), An Episode in the History of Celestial Mechanics and its Utility in the Teaching of Applied Mathematics , Learn from the Masters, The Mathematical Association of America, ISBN   978-0883857038, ... in one of his attacks on Leibniz written in 1711, Newton says that centrifugal force is always equal and opposite to the force of gravity by the third law of motion.
  11. ↑ Singh, Chandralekha (2009), "Centripetal Acceleration: Often Forgotten or Misinterpreted", Physics Education, 44 (5): 464–468, arXiv: 1602.06361 , Bibcode:2009PhyEd..44..464S, doi:10.1088/0031-9120/44/5/001, S2CID   118701050, Another difficulty is that students often consider the pseudo forces, e.g., the centrifugal force, as though they were real forces acting in an inertial reference frame.

Bibliography

This page is based on this Wikipedia article
Text is available under the CC BY-SA 4.0 license; additional terms may apply.
Images, videos and audio are available under their respective licenses.