In Euclidean geometry, a kite is a quadrilateral with reflection symmetry across a diagonal. Because of this symmetry, a kite has two equal angles and two pairs of adjacent equal-length sides. Kites are also known as deltoids, but the word deltoid may also refer to a deltoid curve, an unrelated geometric object sometimes studied in connection with quadrilaterals. A kite may also be called a dart, particularly if it is not convex.

In geometry, the lemniscate of Bernoulli is a plane curve defined from two given points F1 and F2, known as foci, at distance 2c from each other as the locus of points P so that PF1·PF2 = c2. The curve has a shape similar to the numeral 8 and to the ∞ symbol. Its name is from lemniscatus, which is Latin for "decorated with hanging ribbons". It is a special case of the Cassini oval and is a rational algebraic curve of degree 4.
In mathematics, the isoperimetric inequality is a geometric inequality involving the square of the circumference of a closed curve in the plane and the area of a plane region it encloses, as well as its various generalizations. Isoperimetric literally means "having the same perimeter". Specifically, the isoperimetric inequality states, for the length L of a closed curve and the area A of the planar region that it encloses, that

In geometry, a circular segment or disk segment is a region of a disk which is "cut off" from the rest of the disk by a straight line. The complete line is known as a secant, and the section inside the disk as a chord.

In mathematics, a semicircle is a one-dimensional locus of points that forms half of a circle. It is a circular arc that measures 180°. It only has one line of symmetry.

In geometry, a convex polygon is a polygon that is the boundary of a convex set. This means that the line segment between two points of the polygon is contained in the union of the interior and the boundary of the polygon. In particular, it is a simple polygon. Equivalently, a polygon is convex if every line that does not contain any edge intersects the polygon in at most two points.

A cone is a three-dimensional geometric shape that tapers smoothly from a flat base to a point called the apex or vertex.

In geometry, a simple polygon is a polygon that does not intersect itself and has no holes. That is, it is a piecewise-linear Jordan curve consisting of finitely many line segments. These polygons include as special cases the convex polygons, star-shaped polygons, and monotone polygons.

To hear the shape of a drum is to infer information about the shape of the drumhead from the sound it makes, i.e., from the list of overtones, via the use of mathematical theory.

In geometry, a hypotrochoid is a roulette traced by a point attached to a circle of radius r rolling around the inside of a fixed circle of radius R, where the point is a distance d from the center of the interior circle.

In 2-dimensional geometry, a lens is a convex region bounded by two circular arcs joined to each other at their endpoints. In order for this shape to be convex, both arcs must bow outwards (convex-convex). This shape can be formed as the intersection of two circular disks. It can also be formed as the union of two circular segments, joined along a common chord.

A dynamical billiard is a dynamical system in which a particle alternates between free motion and specular reflections from a boundary. When the particle hits the boundary it reflects from it without loss of speed. Billiards are Hamiltonian idealizations of the game of billiards, but where the region contained by the boundary can have shapes other than rectangular and even be multidimensional. Dynamical billiards may also be studied on non-Euclidean geometries; indeed, the first studies of billiards established their ergodic motion on surfaces of constant negative curvature. The study of billiards which are kept out of a region, rather than being kept in a region, is known as outer billiard theory.
In mathematics, the moving sofa problem or sofa problem is a two-dimensional idealization of real-life furniture-moving problems and asks for the rigid two-dimensional shape of the largest area that can be maneuvered through an L-shaped planar region with legs of unit width. The area thus obtained is referred to as the sofa constant. The exact value of the sofa constant is an open problem. The leading solution, by Joseph L. Gerver, has a value of approximately 2.2195. In November 2024, Jineon Baek posted an arXiv preprint claiming that Gerver's value is optimal, which if true, would solve the moving sofa problem.

The salinon is a geometrical figure that consists of four semicircles. It was first introduced in the Book of Lemmas, a work attributed to Archimedes.

Systolic geometry is a branch of differential geometry, a field within mathematics, studying problems such as the relationship between the area inside a closed curve C, and the length or perimeter of C. Since the area A may be small while the length l is large, when C looks elongated, the relationship can only take the form of an inequality. What is more, such an inequality would be an upper bound for A: there is no interesting lower bound just in terms of the length.

In geometry, a convex curve is a plane curve that has a supporting line through each of its points. There are many other equivalent definitions of these curves, going back to Archimedes. Examples of convex curves include the convex polygons, the boundaries of convex sets, and the graphs of convex functions. Important subclasses of convex curves include the closed convex curves, the smooth curves that are convex, and the strictly convex curves, which have the additional property that each supporting line passes through a unique point of the curve.
In mathematics a translation surface is a surface obtained from identifying the sides of a polygon in the Euclidean plane by translations. An equivalent definition is a Riemann surface together with a holomorphic 1-form.
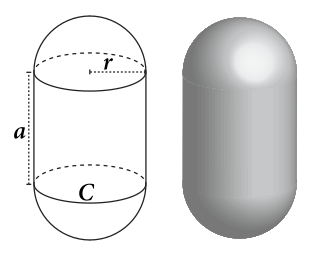
A capsule, or stadium of revolution, is a basic three-dimensional geometric shape consisting of a cylinder with hemispherical ends. Another name for this shape is spherocylinder.
In geometry, a circular triangle is a triangle with circular arc edges.