In mathematics, the continuum hypothesis is a hypothesis about the possible sizes of infinite sets. It states:
There is no set whose cardinality is strictly between that of the integers and the real numbers.
In mathematics, cardinal numbers, or cardinals for short, are a generalization of the natural numbers used to measure the cardinality (size) of sets. The cardinality of a finite set is a natural number: the number of elements in the set. The transfinite cardinal numbers, often denoted using the Hebrew symbol (aleph) followed by a subscript, describe the sizes of infinite sets.
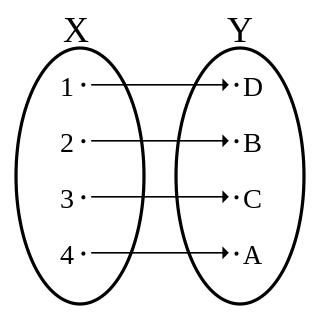
In mathematics, the cardinality of a set is a measure of the "number of elements" of the set. For example, the set contains 3 elements, and therefore has a cardinality of 3. Beginning in the late 19th century, this concept was generalized to infinite sets, which allows one to distinguish between different types of infinity, and to perform arithmetic on them. There are two approaches to cardinality: one which compares sets directly using bijections and injections, and another which uses cardinal numbers. The cardinality of a set is also called its size, when no confusion with other notions of size is possible.

Georg Ferdinand Ludwig Philipp Cantor was a German mathematician. He played a pivotal role in the creation of set theory, which has become a fundamental theory in mathematics. Cantor established the importance of one-to-one correspondence between the members of two sets, defined infinite and well-ordered sets, and proved that the real numbers are more numerous than the natural numbers. In fact, Cantor's method of proof of this theorem implies the existence of an infinity of infinities. He defined the cardinal and ordinal numbers and their arithmetic. Cantor's work is of great philosophical interest, a fact he was well aware of.
In mathematics, an uncountable set is an infinite set that contains too many elements to be countable. The uncountability of a set is closely related to its cardinal number: a set is uncountable if its cardinal number is larger than that of the set of all natural numbers.

In set theory, Cantor's diagonal argument, also called the diagonalisation argument, the diagonal slash argument, the anti-diagonal argument, the diagonal method, and Cantor's diagonalization proof, was published in 1891 by Georg Cantor as a mathematical proof that there are infinite sets which cannot be put into one-to-one correspondence with the infinite set of natural numbers. Such sets are now known as uncountable sets, and the size of infinite sets is now treated by the theory of cardinal numbers which Cantor began.
In mathematics, transfinite numbers are numbers that are "infinite" in the sense that they are larger than all finite numbers, yet not necessarily absolutely infinite. These include the transfinite cardinals, which are cardinal numbers used to quantify the size of infinite sets, and the transfinite ordinals, which are ordinal numbers used to provide an ordering of infinite sets. The term transfinite was coined by Georg Cantor in 1895, who wished to avoid some of the implications of the word infinite in connection with these objects, which were, nevertheless, not finite. Few contemporary writers share these qualms; it is now accepted usage to refer to transfinite cardinals and ordinals as infinite numbers. Nevertheless, the term "transfinite" also remains in use.

Rudolf von Bitter Rucker is an American mathematician, computer scientist, science fiction author, and one of the founders of the cyberpunk literary movement. The author of both fiction and non-fiction, he is best known for the novels in the Ware Tetralogy, the first two of which both won Philip K. Dick Awards. Until its closure in 2014 he edited the science fiction webzine Flurb.

In mathematics, particularly in set theory, the aleph numbers are a sequence of numbers used to represent the cardinality of infinite sets that can be well-ordered. They were introduced by the mathematician Georg Cantor and are named after the symbol he used to denote them, the Hebrew letter aleph.
"99 Bottles of Beer" or "100 Bottles of Pop on the Wall" is a song dating to the mid-20th century. It is a traditional reverse counting song in both the United States and Canada. It is popular to sing on road trips, as it has a very repetitive format which is easy to memorize and can take a long time when families sing. In particular, the song is often sung by children on long school bus trips, such as class field trips, or on Scout or Girl Guide outings.

Everything and More: A Compact History of Infinity is a book by American novelist and essayist David Foster Wallace that examines the history of infinity, focusing primarily on the work of Georg Cantor, the 19th-century German mathematician who created set theory. The book is part of the W. W. Norton "Great Discoveries" series.
In set theory, the cardinality of the continuum is the cardinality or "size" of the set of real numbers , sometimes called the continuum. It is an infinite cardinal number and is denoted by or .

Gyula Kőnig was a mathematician from Hungary. His mathematical publications in German appeared under the name Julius König. His son Dénes Kőnig was a graph theorist.

In philosophy and theology, infinity is explored in articles under headings such as the Absolute, God, and Zeno's paradoxes.
Pocket set theory (PST) is an alternative set theory in which there are only two infinite cardinal numbers, ℵ0 and c. The theory was first suggested by Rudy Rucker in his Infinity and the Mind. The details set out in this entry are due to the American mathematician Randall M. Holmes.
Infinity is that which is boundless, endless, or larger than any natural number. It is often denoted by the infinity symbol .

Infinity and the Mind: The Science and Philosophy of the Infinite is a popular mathematics book by American mathematician, computer scientist, and science fiction writer Rudy Rucker.
In the mathematical field of set theory, the continuum means the real numbers, or the corresponding (infinite) cardinal number, denoted by . Georg Cantor proved that the cardinality is larger than the smallest infinity, namely, . He also proved that is equal to , the cardinality of the power set of the natural numbers.
In the mathematical discipline of set theory, a cardinal characteristic of the continuum is an infinite cardinal number that may consistently lie strictly between , and the cardinality of the continuum, that is, the cardinality of the set of all real numbers. The latter cardinal is denoted or . A variety of such cardinal characteristics arise naturally, and much work has been done in determining what relations between them are provable, and constructing models of set theory for various consistent configurations of them.











