
In mathematics, the Fibonacci sequence is a sequence in which each number is the sum of the two preceding ones. Numbers that are part of the Fibonacci sequence are known as Fibonacci numbers, commonly denoted Fn . The sequence commonly starts from 0 and 1, although some authors start the sequence from 1 and 1 or sometimes from 1 and 2. Starting from 0 and 1, the sequence begins

In mathematics, a square-free integer (or squarefree integer) is an integer which is divisible by no square number other than 1. That is, its prime factorization has exactly one factor for each prime that appears in it. For example, 10 = 2 ⋅ 5 is square-free, but 18 = 2 ⋅ 3 ⋅ 3 is not, because 18 is divisible by 9 = 32. The smallest positive square-free numbers are
2 (two) is a number, numeral and digit. It is the natural number following 1 and preceding 3. It is the smallest and only even prime number. Because it forms the basis of a duality, it has religious and spiritual significance in many cultures.
10 (ten) is the even natural number following 9 and preceding 11. Ten is the base of the decimal numeral system, the most common system of denoting numbers in both spoken and written language.
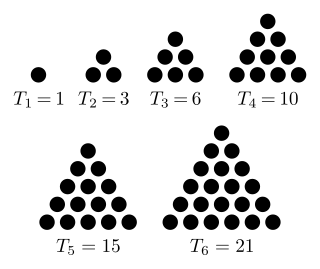
A triangular number or triangle number counts objects arranged in an equilateral triangle. Triangular numbers are a type of figurate number, other examples being square numbers and cube numbers. The nth triangular number is the number of dots in the triangular arrangement with n dots on each side, and is equal to the sum of the n natural numbers from 1 to n. The sequence of triangular numbers, starting with the 0th triangular number, is

In mathematics, a square number or perfect square is an integer that is the square of an integer; in other words, it is the product of some integer with itself. For example, 9 is a square number, since it equals 32 and can be written as 3 × 3.
In mathematics, a polygonal number is a number represented as dots or pebbles arranged in the shape of a regular polygon. The dots are thought of as alphas (units). These are one type of 2-dimensional figurate numbers.
1000 or one thousand is the natural number following 999 and preceding 1001. In most English-speaking countries, it can be written with or without a comma or sometimes a period separating the thousands digit: 1,000.
A powerful number is a positive integer m such that for every prime number p dividing m, p2 also divides m. Equivalently, a powerful number is the product of a square and a cube, that is, a number m of the form m = a2b3, where a and b are positive integers. Powerful numbers are also known as squareful, square-full, or 2-full. Paul Erdős and George Szekeres studied such numbers and Solomon W. Golomb named such numbers powerful.

A hexagonal number is a figurate number. The nth hexagonal number hn is the number of distinct dots in a pattern of dots consisting of the outlines of regular hexagons with sides up to n dots, when the hexagons are overlaid so that they share one vertex.

In mathematics and combinatorics, a centered hexagonal number, or hex number, is a centered figurate number that represents a hexagon with a dot in the center and all other dots surrounding the center dot in a hexagonal lattice. The following figures illustrate this arrangement for the first four centered hexagonal numbers:

A tetrahedral number, or triangular pyramidal number, is a figurate number that represents a pyramid with a triangular base and three sides, called a tetrahedron. The nth tetrahedral number, Ten, is the sum of the first n triangular numbers, that is,

In mathematics, a pyramid number, or square pyramidal number, is a natural number that counts the number of stacked spheres in a pyramid with a square base. The study of these numbers goes back to Archimedes and Fibonacci. They are part of a broader topic of figurate numbers representing the numbers of points forming regular patterns within different shapes.

A star number is a centered figurate number, a centered hexagram, such as the Star of David, or the board Chinese checkers is played on.

In elementary number theory, a centered square number is a centered figurate number that gives the number of dots in a square with a dot in the center and all other dots surrounding the center dot in successive square layers. That is, each centered square number equals the number of dots within a given city block distance of the center dot on a regular square lattice. While centered square numbers, like figurate numbers in general, have few if any direct practical applications, they are sometimes studied in recreational mathematics for their elegant geometric and arithmetic properties.
The centered polygonal numbers are a class of series of figurate numbers, each formed by a central dot, surrounded by polygonal layers of dots with a constant number of sides. Each side of a polygonal layer contains one more dot than each side in the previous layer; so starting from the second polygonal layer, each layer of a centered k-gonal number contains k more dots than the previous layer.
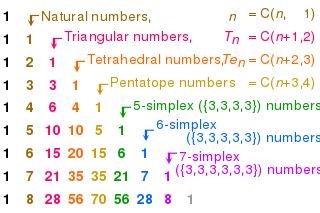
In number theory, a pentatope number is a number in the fifth cell of any row of Pascal's triangle starting with the 5-term row 1 4 6 4 1, either from left to right or from right to left. It is named because it represents the number of 3-dimensional unit spheres which can be packed into a pentatope of increasing side lengths.

In number theory, a practical number or panarithmic number is a positive integer such that all smaller positive integers can be represented as sums of distinct divisors of . For example, 12 is a practical number because all the numbers from 1 to 11 can be expressed as sums of its divisors 1, 2, 3, 4, and 6: as well as these divisors themselves, we have 5 = 3 + 2, 7 = 6 + 1, 8 = 6 + 2, 9 = 6 + 3, 10 = 6 + 3 + 1, and 11 = 6 + 3 + 2.

In number theory, a polite number is a positive integer that can be written as the sum of two or more consecutive positive integers. A positive integer which is not polite is called impolite. The impolite numbers are exactly the powers of two, and the polite numbers are the natural numbers that are not powers of two.
288 is the natural number following 287 and preceding 289. Because 288 = 2 · 12 · 12, it may also be called "two gross" or "two dozen dozen".



























