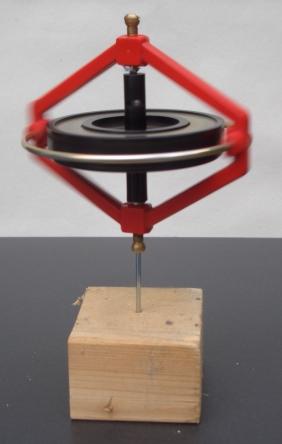
In physics, angular momentum is the rotational analog of linear momentum. It is an important physical quantity because it is a conserved quantity – the total angular momentum of a closed system remains constant. Angular momentum has both a direction and a magnitude, and both are conserved. Bicycles and motorcycles, flying discs, rifled bullets, and gyroscopes owe their useful properties to conservation of angular momentum. Conservation of angular momentum is also why hurricanes form spirals and neutron stars have high rotational rates. In general, conservation limits the possible motion of a system, but it does not uniquely determine it.
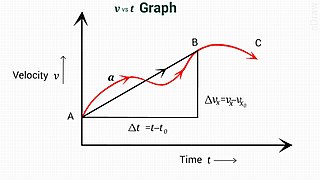
In physics, equations of motion are equations that describe the behavior of a physical system in terms of its motion as a function of time. More specifically, the equations of motion describe the behavior of a physical system as a set of mathematical functions in terms of dynamic variables. These variables are usually spatial coordinates and time, but may include momentum components. The most general choice are generalized coordinates which can be any convenient variables characteristic of the physical system. The functions are defined in a Euclidean space in classical mechanics, but are replaced by curved spaces in relativity. If the dynamics of a system is known, the equations are the solutions for the differential equations describing the motion of the dynamics.

In special relativity, a four-vector is an object with four components, which transform in a specific way under Lorentz transformations. Specifically, a four-vector is an element of a four-dimensional vector space considered as a representation space of the standard representation of the Lorentz group, the representation. It differs from a Euclidean vector in how its magnitude is determined. The transformations that preserve this magnitude are the Lorentz transformations, which include spatial rotations and boosts.
A prior probability distribution of an uncertain quantity, often simply called the prior, is its assumed probability distribution before some evidence is taken into account. For example, the prior could be the probability distribution representing the relative proportions of voters who will vote for a particular politician in a future election. The unknown quantity may be a parameter of the model or a latent variable rather than an observable variable.
In information geometry, the Fisher information metric is a particular Riemannian metric which can be defined on a smooth statistical manifold, i.e., a smooth manifold whose points are probability measures defined on a common probability space. It can be used to calculate the informational difference between measurements.
In mathematical statistics, the Fisher information is a way of measuring the amount of information that an observable random variable X carries about an unknown parameter θ of a distribution that models X. Formally, it is the variance of the score, or the expected value of the observed information.
In particle and condensed matter physics, Goldstone bosons or Nambu–Goldstone bosons (NGBs) are bosons that appear necessarily in models exhibiting spontaneous breakdown of continuous symmetries. They were discovered by Yoichiro Nambu in particle physics within the context of the BCS superconductivity mechanism, and subsequently elucidated by Jeffrey Goldstone, and systematically generalized in the context of quantum field theory. In condensed matter physics such bosons are quasiparticles and are known as Anderson–Bogoliubov modes.
In physics and astronomy, the Reissner–Nordström metric is a static solution to the Einstein–Maxwell field equations, which corresponds to the gravitational field of a charged, non-rotating, spherically symmetric body of mass M. The analogous solution for a charged, rotating body is given by the Kerr–Newman metric.
The adiabatic theorem is a concept in quantum mechanics. Its original form, due to Max Born and Vladimir Fock (1928), was stated as follows:
In theoretical physics, supersymmetric quantum mechanics is an area of research where supersymmetry are applied to the simpler setting of plain quantum mechanics, rather than quantum field theory. Supersymmetric quantum mechanics has found applications outside of high-energy physics, such as providing new methods to solve quantum mechanical problems, providing useful extensions to the WKB approximation, and statistical mechanics.
The old quantum theory is a collection of results from the years 1900–1925 which predate modern quantum mechanics. The theory was never complete or self-consistent, but was instead a set of heuristic corrections to classical mechanics. The theory has come to be understood as the semi-classical approximation to modern quantum mechanics. The main and final accomplishments of the old quantum theory were the determination of the modern form of the periodic table by Edmund Stoner and the Pauli exclusion principle which were both premised on the Arnold Sommerfeld enhancements to the Bohr model of the atom.
The Kuramoto model, first proposed by Yoshiki Kuramoto, is a mathematical model used in describing synchronization. More specifically, it is a model for the behavior of a large set of coupled oscillators. Its formulation was motivated by the behavior of systems of chemical and biological oscillators, and it has found widespread applications in areas such as neuroscience and oscillating flame dynamics. Kuramoto was quite surprised when the behavior of some physical systems, namely coupled arrays of Josephson junctions, followed his model.

In thermodynamics, the fundamental thermodynamic relation are four fundamental equations which demonstrate how four important thermodynamic quantities depend on variables that can be controlled and measured experimentally. Thus, they are essentially equations of state, and using the fundamental equations, experimental data can be used to determine sought-after quantities like G or H (enthalpy). The relation is generally expressed as a microscopic change in internal energy in terms of microscopic changes in entropy, and volume for a closed system in thermal equilibrium in the following way.
In fluid mechanics, potential vorticity (PV) is a quantity which is proportional to the dot product of vorticity and stratification. This quantity, following a parcel of air or water, can only be changed by diabatic or frictional processes. It is a useful concept for understanding the generation of vorticity in cyclogenesis, especially along the polar front, and in analyzing flow in the ocean.
In classical mechanics, the Hannay angle is a mechanics analogue of the whirling geometric phase. It was named after John Hannay of the University of Bristol, UK. Hannay first described the angle in 1985, extending the ideas of the recently formalized Berry phase to classical mechanics.
The theoretical and experimental justification for the Schrödinger equation motivates the discovery of the Schrödinger equation, the equation that describes the dynamics of nonrelativistic particles. The motivation uses photons, which are relativistic particles with dynamics described by Maxwell's equations, as an analogue for all types of particles.
In physics, Berry connection and Berry curvature are related concepts which can be viewed, respectively, as a local gauge potential and gauge field associated with the Berry phase or geometric phase. The concept was first introduced by S. Pancharatnam as geometric phase and later elaborately explained and popularized by Michael Berry in a paper published in 1984 emphasizing how geometric phases provide a powerful unifying concept in several branches of classical and quantum physics.
In quantum information theory, the Wehrl entropy, named after Alfred Wehrl, is a classical entropy of a quantum-mechanical density matrix. It is a type of quasi-entropy defined for the Husimi Q representation of the phase-space quasiprobability distribution. See for a comprehensive review of basic properties of classical, quantum and Wehrl entropies, and their implications in statistical mechanics.

In continuum mechanics, Whitham's averaged Lagrangian method – or in short Whitham's method – is used to study the Lagrangian dynamics of slowly-varying wave trains in an inhomogeneous (moving) medium. The method is applicable to both linear and non-linear systems. As a direct consequence of the averaging used in the method, wave action is a conserved property of the wave motion. In contrast, the wave energy is not necessarily conserved, due to the exchange of energy with the mean motion. However the total energy, the sum of the energies in the wave motion and the mean motion, will be conserved for a time-invariant Lagrangian. Further, the averaged Lagrangian has a strong relation to the dispersion relation of the system.
Rayleigh–Lorentz pendulum is a simple pendulum, but subjected to a slowly varying frequency due to an external action, named after Lord Rayleigh and Hendrik Lorentz. This problem formed the basis for the concept of adiabatic invariants in mechanics. On account of the slow variation of frequency, it is shown that the ratio of average energy to frequency is constant.




















![Pendulum with extra small vibration, where
o
(
t
)
=
g
/
L
(
t
)
[?]
g
/
L
0
,
{\displaystyle \omega (t)={\sqrt {g/L(t)}}\approx {\sqrt {g/L_{0}}},}
and
L
(
t
)
[?]
L
0
+
e
(
t
)
.
{\displaystyle L(t)\approx L_{0}+\varepsilon (t).} Adiabatic-pendulum.png](http://upload.wikimedia.org/wikipedia/commons/thumb/7/7b/Adiabatic-pendulum.png/200px-Adiabatic-pendulum.png)



































