| Allotalanta autophaea | |
|---|---|
Scientific classification  | |
| Domain: | Eukaryota |
| Kingdom: | Animalia |
| Phylum: | Arthropoda |
| Class: | Insecta |
| Order: | Lepidoptera |
| Family: | Cosmopterigidae |
| Genus: | Allotalanta |
| Species: | A. autophaea |
| Binomial name | |
| Allotalanta autophaea Meyrick, 1913 | |
Allotalanta autophaea is a moth of the family Cosmopterigidae. It is found in Turkey. [1]
The wingspan is 19–20 mm (0.75–0.79 in). Adults are on wing in June.
In mathematics, convolution is a mathematical operation on two functions that produces a third function that expresses how the shape of one is modified by the other. The term convolution refers to both the result function and to the process of computing it. It is defined as the integral of the product of the two functions after one is reflected about the y-axis and shifted. The choice of which function is reflected and shifted before the integral does not change the integral result. The integral is evaluated for all values of shift, producing the convolution function.
In physics, the kinetic energy of an object is the form of energy that it possesses due to its motion.
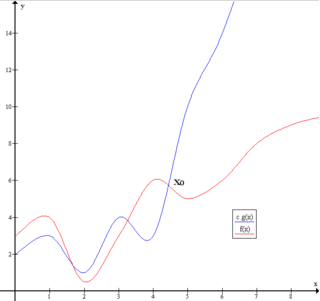
In mathematics, the mean value theorem states, roughly, that for a given planar arc between two endpoints, there is at least one point at which the tangent to the arc is parallel to the secant through its endpoints. It is one of the most important results in real analysis. This theorem is used to prove statements about a function on an interval starting from local hypotheses about derivatives at points of the interval.
In mathematical physics, the Dirac delta distribution, also known as the unit impulse, is a generalized function or distribution over the real numbers, whose value is zero everywhere except at zero, and whose integral over the entire real line is equal to one.
Big O notation is a mathematical notation that describes the limiting behavior of a function when the argument tends towards a particular value or infinity. Big O is a member of a family of notations invented by German mathematicians Paul Bachmann, Edmund Landau, and others, collectively called Bachmann–Landau notation or asymptotic notation. The letter O was chosen by Bachmann to stand for Ordnung, meaning the order of approximation.
In physics and mathematics, the Fourier transform (FT) is a transform that converts a function into a form that describes the frequencies present in the original function. The output of the transform is a complex-valued function of frequency. The term Fourier transform refers to both this complex-valued function and the mathematical operation. When a distinction needs to be made the Fourier transform is sometimes called the frequency domain representation of the original function. The Fourier transform is analogous to decomposing the sound of a musical chord into terms of the intensity of its constituent pitches.
In mathematics, a function from a set X to a set Y assigns to each element of X exactly one element of Y. The set X is called the domain of the function and the set Y is called the codomain of the function.
Some Buddhist terms and concepts lack direct translations into English that cover the breadth of the original term. Below are given a number of important Buddhist terms, short definitions, and the languages in which they appear. In this list, an attempt has been made to organize terms by their original form and give translations and synonyms in other languages along with the definition.
The Cosmopterigidae are a family of insects in the order Lepidoptera. These are small moths with narrow wings whose tiny larvae feed internally on the leaves, seeds and stems of their host plants. About 1500 species are described. The taxonomic family is most diverse in the Australian and Pacific region with about 780 species.
Allotalanta is a genus of moths in the family Cosmopterigidae. It was first described by Edward Meyrick in 1913.
The Scaeosophinae are a subfamily of the Cosmopterigidae.
Allotalanta clonomicta is a moth in the family Cosmopterigidae. It was described by Edward Meyrick in 1927. It is found in South Africa.
Allotalanta deceptrix is a moth in the family Cosmopterigidae. It was described by Edward Meyrick in 1925. It is found in China (Liaoning).
Allotalanta globulosa is a moth in the family Cosmopterigidae. It was described by Edward Meyrick in 1914. It is found in India (Assam) and Sri Lanka.
Allotalanta lacteata is a moth in the family Cosmopterigidae. It was described by Edward Meyrick in 1914. It is found in India (Coorg) and Sri Lanka.
Allotalanta ochthotoma is a moth in the family Cosmopterigidae. It was described by Edward Meyrick in 1930. It is found in Cameroon.
Allotalanta oporista is a moth in the family Cosmopterigidae. It was described by Edward Meyrick in 1926. It is found in New Ireland.
Allotalanta spilothyris is a moth in the family Cosmopterigidae. It was described by Edward Meyrick in 1922. It is found in India (Assam).
Allotalanta synclera is a moth in the family Cosmopterigidae. It was described by Edward Meyrick in 1921. It is found in India (Kanara).
Allotalanta tephroclystis is a moth in the family Cosmopterigidae. It was described by Edward Meyrick in 1930. It is found in Cameroon.
