Central configuration

Last updated

In celestial mechanics, a central configuration is a system of point masses with the property that each mass is pulled by the combined gravitational force of the system directly towards the center of mass, with acceleration proportional to its distance from the center. Central configurations are studied in n-body problems formulated in Euclidean spaces of any dimension, although only dimensions one, two, and three are directly relevant for celestial mechanics in physical space. [1] [2]

Contents

Examples

For n equal masses, one possible central configuration places the masses at the vertices of a regular polygon (forming a Klemperer rosette), a Platonic solid, or a regular polytope in higher dimensions. The centrality of the configuration follows from its symmetry. It is also possible to place an additional point, of arbitrary mass, at the center of mass of the system without changing its centrality. [1]

Placing three masses in an equilateral triangle, four at the vertices of a regular tetrahedron, or more generally n masses at the vertices of a regular simplex produces a central configuration even when the masses are not equal. This is the only central configuration for these masses that does not lie in a lower-dimensional subspace. [1]

Dynamics

Under Newton's law of universal gravitation, bodies placed at rest in a central configuration will maintain the configuration as they collapse to a collision at their center of mass. Systems of bodies in a two-dimensional central configuration can orbit stably around their center of mass, maintaining their relative positions, with circular orbits around the center of mass or in elliptical orbits with the center of mass at a focus of the ellipse. These are the only possible stable orbits in three-dimensional space in which the system of particles always remains similar to its initial configuration. [1]

More generally, any system of particles moving under Newtonian gravitation that all collide at a single point in time and space will approximate a central configuration, in the limit as time tends to the collision time. Similarly, a system of particles that eventually all escape each other at exactly the escape velocity will approximate a central configuration in the limit as time tends to infinity. And any system of particles that move under Newtonian gravitation as if they are a rigid body must do so in a central configuration. Vortices in two-dimensional fluid dynamics, such as large storm systems on the Earth's oceans, also tend to arrange themselves in central configurations. [2]

Enumeration

Two central configurations are considered to be equivalent if they are similar, that is, they can be transformed into each other by some combination of rotation, translation, and scaling. With this definition of equivalence, there is only one configuration of one or two points, and it is always central.

In the case of three bodies, there are three one-dimensional central configurations, found by Leonhard Euler. The finiteness of the set of three-point central configurations was shown by Joseph-Louis Lagrange in his solution to the three-body problem; Lagrange showed that there is only one non-collinear central configuration, in which the three points form the vertices of an equilateral triangle. [2]

Four points in any dimension have only finitely many central configurations. The number of configurations in this case is at least 32 and at most 8472, depending on the masses of the points. [3] [4] The only convex central configuration of four equal masses is a square. [5] The only central configuration of four masses that spans three dimensions is the configuration formed by the vertices of a regular tetrahedron. [6]

For arbitrarily many points in one dimension, there are again only finitely many solutions, one for each of the n!/2 linear orderings (up to reversal of the ordering) of the points on a line. [1] [2] [7] [8]

Unsolved problem in mathematics:
Is there a bounded number of central configurations for every finite collection of point masses in every dimension?
(more unsolved problems in mathematics)

For every set of n point masses, and every dimension less than n, there exists at least one central configuration of that dimension. [1] For almost all n-tuples of masses there are finitely many "Dziobek" configurations that span exactly n− 2 dimensions. [1] It is an unsolved problem, posed by Chazy (1918) and Wintner (1941), whether there is always a bounded number of central configurations for five or more masses in two or more dimensions. In 1998, Stephen Smale included this problem as the sixth in his list of "mathematical problems for the next century". [2] [9] [10] [11] As partial progress, for almost all 5-tuples of masses, there are only a bounded number of two-dimensional central configurations of five points. [12]

Special classes of configurations

Stacked

A central configuration is said to be stacked if a subset of three or more of its masses also form a central configuration. For example, this can be true for equal masses forming a square pyramid, with the four masses at the base of the pyramid also forming a central configuration, or for masses forming a triangular bipyramid, with the three masses in the central triangle of the bipyramid also forming a central configuration. [13]

Spiderweb

A spiderweb central configuration is a configuration in which the masses lie at the intersection points of a collection of concentric circles with another collection of lines, meeting at the center of the circles with equal angles. The intersection points of the lines with a single circle should all be occupied by points of equal mass, but the masses may vary from circle to circle. An additional mass (which may be zero) is placed at the center of the system. For any desired number of lines, number of circles, and profile of the masses on each concentric circle of a spiderweb central configuration, it is possible to find a spiderweb central configuration matching those parameters. [14] [15] One can similarly obtain central configurations for families of nested Platonic solids, or more generally group-theoretic orbits of any finite subgroup of the orthogonal group. [16]

James Clerk Maxwell suggested that a special case of these configurations with one circle, a massive central body, and much lighter bodies at equally spaced points on the circle could be used to understand the motion of the rings of Saturn. [14] [17] Saari (2015) used stable orbits generated from spiderweb central configurations with known mass distribution to test the accuracy of classical estimation methods for the mass distribution of galaxies. His results showed that these methods could be quite inaccurate, potentially showing that less dark matter is needed to predict galactic motion than standard theories predict. [14]

Related Research Articles

<span class="mw-page-title-main">Lagrange point</span> Equilibrium points near two orbiting bodies

In celestial mechanics, the Lagrange points are points of equilibrium for small-mass objects under the gravitational influence of two massive orbiting bodies. Mathematically, this involves the solution of the restricted three-body problem.

<span class="mw-page-title-main">Mass</span> Amount of matter present in an object

Mass is an intrinsic property of a body. It was traditionally believed to be related to the quantity of matter in a body, until the discovery of the atom and particle physics. It was found that different atoms and different elementary particles, theoretically with the same amount of matter, have nonetheless different masses. Mass in modern physics has multiple definitions which are conceptually distinct, but physically equivalent. Mass can be experimentally defined as a measure of the body's inertia, meaning the resistance to acceleration when a net force is applied. The object's mass also determines the strength of its gravitational attraction to other bodies.

<span class="mw-page-title-main">Orbit</span> Curved path of an object around a point

In celestial mechanics, an orbit is the curved trajectory of an object such as the trajectory of a planet around a star, or of a natural satellite around a planet, or of an artificial satellite around an object or position in space such as a planet, moon, asteroid, or Lagrange point. Normally, orbit refers to a regularly repeating trajectory, although it may also refer to a non-repeating trajectory. To a close approximation, planets and satellites follow elliptic orbits, with the center of mass being orbited at a focal point of the ellipse, as described by Kepler's laws of planetary motion.

Celestial mechanics is the branch of astronomy that deals with the motions of objects in outer space. Historically, celestial mechanics applies principles of physics to astronomical objects, such as stars and planets, to produce ephemeris data.

<span class="mw-page-title-main">Orbital mechanics</span> Field of classical mechanics concerned with the motion of spacecraft

Orbital mechanics or astrodynamics is the application of ballistics and celestial mechanics to the practical problems concerning the motion of rockets, satellites, and other spacecraft. The motion of these objects is usually calculated from Newton's laws of motion and the law of universal gravitation. Orbital mechanics is a core discipline within space-mission design and control.

<span class="mw-page-title-main">Interplanetary Transport Network</span> Low-energy trajectories in the Solar System

The Interplanetary Transport Network (ITN) is a collection of gravitationally determined pathways through the Solar System that require very little energy for an object to follow. The ITN makes particular use of Lagrange points as locations where trajectories through space can be redirected using little or no energy. These points have the peculiar property of allowing objects to orbit around them, despite lacking an object to orbit, as these points exist where gravitational forces between two celestial bodies are equal. While it would use little energy, transport along the network would take a long time.

Newton's law of universal gravitation states that every particle attracts every other particle in the universe with a force that is proportional to the product of their masses and inversely proportional to the square of the distance between their centers. Separated objects attract and are attracted as if all their mass were concentrated at their centers. The publication of the law has become known as the "first great unification", as it marked the unification of the previously described phenomena of gravity on Earth with known astronomical behaviors.

<span class="mw-page-title-main">Gaussian gravitational constant</span> Constant used in orbital mechanics

The Gaussian gravitational constant is a parameter used in the orbital mechanics of the Solar System. It relates the orbital period to the orbit's semi-major axis and the mass of the orbiting body in Solar masses.

In classical mechanics, the Laplace–Runge–Lenz (LRL) vector is a vector used chiefly to describe the shape and orientation of the orbit of one astronomical body around another, such as a binary star or a planet revolving around a star. For two bodies interacting by Newtonian gravity, the LRL vector is a constant of motion, meaning that it is the same no matter where it is calculated on the orbit; equivalently, the LRL vector is said to be conserved. More generally, the LRL vector is conserved in all problems in which two bodies interact by a central force that varies as the inverse square of the distance between them; such problems are called Kepler problems.

<span class="mw-page-title-main">Klemperer rosette</span> Type of gravitational system

A Klemperer rosette is a gravitational system of (optionally) alternating heavier and lighter bodies orbiting in a symmetrical pattern around a common barycenter. It was first described by W.B. Klemperer in 1962, and is a special case of a central configuration.

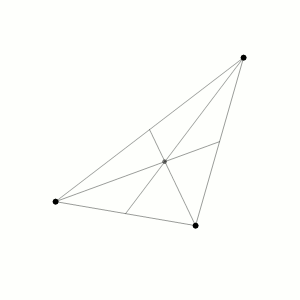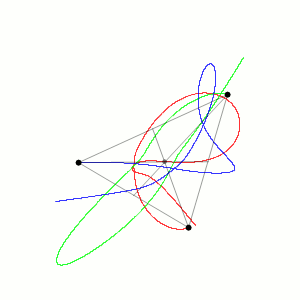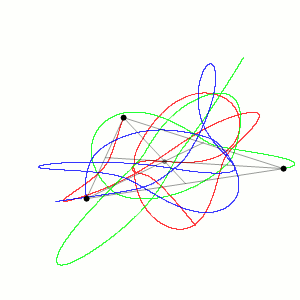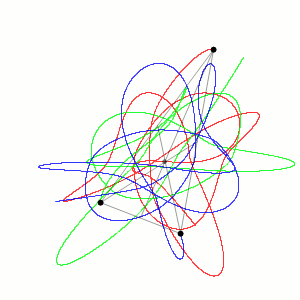
<span class="mw-page-title-main">Three-body problem</span> Physics problem related to laws of motion and gravity

In physics, specifically classical mechanics, the three-body problem is to take the initial positions and velocities of three point masses that orbit each other in space and calculate their subsequent trajectories using Newton's laws of motion and Newton's law of universal gravitation.

<span class="mw-page-title-main">Perturbation (astronomy)</span> Classical approach to the many-body problem of astronomy

In astronomy, perturbation is the complex motion of a massive body subjected to forces other than the gravitational attraction of a single other massive body. The other forces can include a third body, resistance, as from an atmosphere, and the off-center attraction of an oblate or otherwise misshapen body.

In orbital mechanics, mean motion is the angular speed required for a body to complete one orbit, assuming constant speed in a circular orbit which completes in the same time as the variable speed, elliptical orbit of the actual body. The concept applies equally well to a small body revolving about a large, massive primary body or to two relatively same-sized bodies revolving about a common center of mass. While nominally a mean, and theoretically so in the case of two-body motion, in practice the mean motion is not typically an average over time for the orbits of real bodies, which only approximate the two-body assumption. It is rather the instantaneous value which satisfies the above conditions as calculated from the current gravitational and geometric circumstances of the body's constantly-changing, perturbed orbit.

<span class="mw-page-title-main">Osculating orbit</span> Orbital perturbations

In astronomy, and in particular in astrodynamics, the osculating orbit of an object in space at a given moment in time is the gravitational Kepler orbit that it would have around its central body if perturbations were absent. That is, it is the orbit that coincides with the current orbital state vectors.

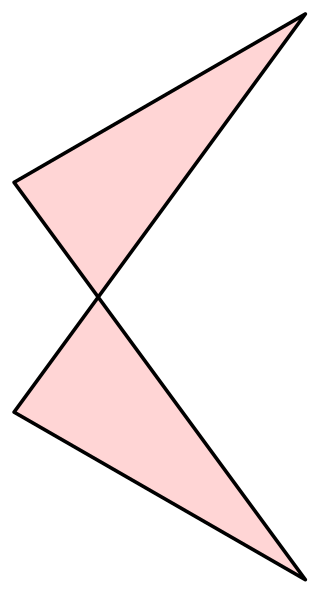
<span class="mw-page-title-main">Antiparallelogram</span> Polygon with four crossed edges of two lengths

In geometry, an antiparallelogram is a type of self-crossing quadrilateral. Like a parallelogram, an antiparallelogram has two opposite pairs of equal-length sides, but these pairs of sides are not in general parallel. Instead, each pair of sides is antiparallel with respect to the other, with sides in the longer pair crossing each other as in a scissors mechanism. Whereas a parallelogram's opposite angles are equal and oriented the same way, an antiparallelogram's are equal but oppositely oriented. Antiparallelograms are also called contraparallelograms or crossed parallelograms.

The two-body problem in general relativity is the determination of the motion and gravitational field of two bodies as described by the field equations of general relativity. Solving the Kepler problem is essential to calculate the bending of light by gravity and the motion of a planet orbiting its sun. Solutions are also used to describe the motion of binary stars around each other, and estimate their gradual loss of energy through gravitational radiation.

<span class="mw-page-title-main">Zero-velocity surface</span>

A zero-velocity surface is a concept that relates to the N-body problem of gravity. It represents a surface a body of given energy cannot cross, since it would have zero velocity on the surface. It was first introduced by George William Hill. The zero-velocity surface is particularly significant when working with weak gravitational interactions among orbiting bodies.

In physics, the n-body problem is the problem of predicting the individual motions of a group of celestial objects interacting with each other gravitationally. Solving this problem has been motivated by the desire to understand the motions of the Sun, Moon, planets, and visible stars. In the 20th century, understanding the dynamics of globular cluster star systems became an important n-body problem. The n-body problem in general relativity is considerably more difficult to solve due to additional factors like time and space distortions.

In geometry, the moment curve is an algebraic curve in d-dimensional Euclidean space given by the set of points with Cartesian coordinates of the form

Zhihong "Jeff" Xia is a Chinese-American mathematician.

References

  1. 1 2 3 4 5 6 7 Moeckel, Richard (2015), "Central configurations", in Llibre, Jaume; Moeckel, Richard; Simó, Carles (eds.), Central Configurations, Periodic Orbits, and Hamiltonian Systems, Advanced Courses in Mathematics - CRM Barcelona, Basel: Springer, pp. 105–167, doi:10.1007/978-3-0348-0933-7_2, ISBN   978-3-0348-0932-0, MR   3469182
  2. 1 2 3 4 5 Saari, Donald G. (2011), "Central Configurations—A Problem for the Twenty-first Century" (PDF), in Shubin, Tatiana; Hayes, David; Alexanderson, Gerald (eds.), Expeditions in mathematics, MAA Spectrum, Washington, DC: Mathematical Association of America, pp. 283–297, ISBN   978-0-88385-571-3, MR   2849696
  3. ↑ Albouy, Alain (1995), "Symétrie des configurations centrales de quatre corps", Comptes rendus de l'Académie des Sciences , 320 (2): 217–220, MR   1320359
  4. ↑ Hampton, Marshall; Moeckel, Richard (2006), "Finiteness of relative equilibria of the four-body problem", Inventiones Mathematicae , 163 (2): 289–312, doi:10.1007/s00222-005-0461-0, MR   2207019, S2CID   1293751
  5. ↑ Albouy, Alain (1996), "The symmetric central configurations of four equal masses", Hamiltonian dynamics and celestial mechanics (Seattle, WA, 1995), Contemporary Mathematics, vol. 198, Providence, Rhode Island: American Mathematical Society, pp. 131–135, doi: 10.1090/conm/198/02494 , ISBN   978-0-8218-0566-4, MR   1409157
  6. ↑ Pizzetti, Paolo (1904), "Casi particolari del problema dei tre corpi", Rendiconti della Reale Accademia dei Lincei, 13: 17–26
  7. ↑ Albouy, Alain; Fu, Yanning (2007), "Euler configurations and quasi-polynomial systems", Regular and Chaotic Dynamics, 12 (1): 39–55, arXiv: math-ph/0603075 , Bibcode:2007RCD....12...39A, doi:10.1134/S1560354707010042, MR   2350295, S2CID   18065509
  8. ↑ Moulton, F. R. (1910), "The straight line solutions of the problem of n bodies", Annals of Mathematics , Second Series, 12 (1): 1–17, doi:10.2307/2007159, JSTOR   2007159, MR   1503509
  9. ↑ Chazy, J. (1918), "Sur certaines trajectoires du problème des n corps", Bulletin Astronomique, 35: 321–389, doi:10.3406/bastr.1918.13419, S2CID   249034773
  10. ↑ Wintner, Aurel (1941), The Analytical Foundations of Celestial Mechanics, Princeton Mathematical Series, vol. 5, Princeton, New Jersey: Princeton University Press, MR   0005824
  11. ↑ Smale, Steve (1998), "Mathematical problems for the next century", The Mathematical Intelligencer , 20 (2): 7–15, doi:10.1007/BF03025291, MR   1631413, S2CID   1331144
  12. ↑ Albouy, Alain; Kaloshin, Vadim (2012), "Finiteness of central configurations of five bodies in the plane", Annals of Mathematics , Second Series, 176 (1): 535–588, doi: 10.4007/annals.2012.176.1.10 , MR   2925390
  13. ↑ Hampton, Marshall (2005), "Stacked central configurations: new examples in the planar five-body problem", Nonlinearity , 18 (5): 2299–2304, doi:10.1088/0951-7715/18/5/021, MR   2164743, S2CID   119863471
  14. 1 2 3 Saari, Donald G. (April 2015), "N-body solutions and computing galactic masses", The Astronomical Journal , 149 (5): 174, Bibcode:2015AJ....149..174S, doi:10.1088/0004-6256/149/5/174, S2CID   119903466
  15. ↑ Hénot, Olivier; Rousseau, Christiane (2019), "Spiderweb central configurations", Qualitative Theory of Dynamical Systems, 18 (3): 1135–1160, arXiv: 1810.09915 , doi: 10.1007/s12346-019-00330-y , MR   4028598
  16. ↑ Montaldi, James (2015), "Existence of symmetric central configurations" (PDF), Celestial Mechanics and Dynamical Astronomy , 122 (4): 405–418, arXiv: 1408.5854 , Bibcode:2015CeMDA.122..405M, doi:10.1007/s10569-015-9625-4, MR   3368140, S2CID   16906550
  17. ↑ Maxwell, James Clerk (1859), On the stability of the motion of Saturn's rings, Cambridge: Macmillan, Bibcode:1859osms.book.....M, doi:10.3931/e-rara-244
This page is based on this Wikipedia article
Text is available under the CC BY-SA 4.0 license; additional terms may apply.
Images, videos and audio are available under their respective licenses.