
In topology and related branches of mathematics, a connected space is a topological space that cannot be represented as the union of two or more disjoint non-empty open subsets. Connectedness is one of the principal topological properties that are used to distinguish topological spaces.

In geometry, a subset of a Euclidean space, or more generally an affine space over the reals, is convex if, given any two points in the subset, the subset contains the whole line segment that joins them. Equivalently, a convex set or a convex region is a subset that intersects every line into a single line segment . For example, a solid cube is a convex set, but anything that is hollow or has an indent, for example, a crescent shape, is not convex.
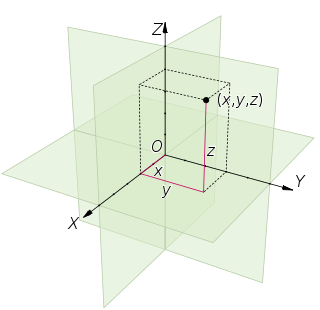
Euclidean space is the fundamental space of geometry, intended to represent physical space. Originally, that is, in Euclid's Elements, it was the three-dimensional space of Euclidean geometry, but in modern mathematics there are Euclidean spaces of any positive integer dimension, including the three-dimensional space and the Euclidean plane. The qualifier "Euclidean" is used to distinguish Euclidean spaces from other spaces that were later considered in physics and modern mathematics.

In mathematics, a metric space is a set together with a notion of distance between its elements, usually called points. The distance is measured by a function called a metric or distance function. Metric spaces are the most general setting for studying many of the concepts of mathematical analysis and geometry.
In mathematics, a topological space is, roughly speaking, a geometrical space in which closeness is defined but cannot necessarily be measured by a numeric distance. More specifically, a topological space is a set whose elements are called points, along with an additional structure called a topology that can be defined as a set of neighbourhoods for each point, that satisfy some axioms formalizing the concept of closeness. There are several equivalent definitions of a topology, the most commonly used is the definition through open sets, which is easier to manipulate.

In mathematics, a curve is an object similar to a line, but that does not have to be straight.

In mathematics, a ball is the solid figure bounded by a sphere; it is also called a solid sphere. It may be a closed ball or an open ball.

In mathematics, general topology is the branch of topology that deals with the basic set-theoretic definitions and constructions used in topology. It is the foundation of most other branches of topology, including differential topology, geometric topology, and algebraic topology. Another name for general topology is point-set topology.
In functional analysis and related areas of mathematics, Fréchet spaces, named after Maurice Fréchet, are special topological vector spaces. They are generalizations of Banach spaces. All Banach and Hilbert spaces are Fréchet spaces. Spaces of infinitely differentiable functions are typical examples of Fréchet spaces, many of which are typically not Banach spaces.
In mathematics, particularly differential geometry, a Finsler manifold is a differentiable manifold M where a Minkowski functionalF(x, −) is provided on each tangent space TxM, that enables one to define the length of any smooth curve γ : [a, b] → M as
In the mathematical study of metric spaces, one can consider the arclength of paths in the space. If two points are at a given distance from each other, it is natural to expect that one should be able to get from the first point to the second along a path whose arclength is equal to that distance. The distance between two points of a metric space relative to the intrinsic metric is defined as the infimum of the lengths of all paths from the first point to the second. A metric space is a length metric space if the intrinsic metric agrees with the original metric of the space.
This is a glossary of some terms used in Riemannian geometry and metric geometry — it doesn't cover the terminology of differential topology.
In mathematics, a fundamental polygon can be defined for every compact Riemann surface of genus greater than 0. It encodes not only the topology of the surface through its fundamental group but also determines the Riemann surface up to conformal equivalence. By the uniformization theorem, every compact Riemann surface has simply connected universal covering surface given by exactly one of the following:
In geometric topology, Busemann functions are used to study the large-scale geometry of geodesics in Hadamard spaces and in particular Hadamard manifolds. They are named after Herbert Busemann, who introduced them; he gave an extensive treatment of the topic in his 1955 book "The geometry of geodesics".
In geometry, an Hadamard space, named after Jacques Hadamard, is a non-linear generalization of a Hilbert space. In the literature they are also equivalently defined as complete CAT(0) spaces.
In mathematics, specifically statistics and information geometry, a Bregman divergence or Bregman distance is a measure of difference between two points, defined in terms of a strictly convex function; they form an important class of divergences. When the points are interpreted as probability distributions – notably as either values of the parameter of a parametric model or as a data set of observed values – the resulting distance is a statistical distance. The most basic Bregman divergence is the squared Euclidean distance.
In mathematics, the Cartan–Hadamard theorem is a statement in Riemannian geometry concerning the structure of complete Riemannian manifolds of non-positive sectional curvature. The theorem states that the universal cover of such a manifold is diffeomorphic to a Euclidean space via the exponential map at any point. It was first proved by Hans Carl Friedrich von Mangoldt for surfaces in 1881, and independently by Jacques Hadamard in 1898. Élie Cartan generalized the theorem to Riemannian manifolds in 1928. The theorem was further generalized to a wide class of metric spaces by Mikhail Gromov in 1987; detailed proofs were published by Ballmann (1990) for metric spaces of non-positive curvature and by Alexander & Bishop (1990) for general locally convex metric spaces.
In mathematics — specifically, in Riemannian geometry — geodesic convexity is a natural generalization of convexity for sets and functions to Riemannian manifolds. It is common to drop the prefix "geodesic" and refer simply to "convexity" of a set or function.
In mathematics, a space, where is a real number, is a specific type of metric space. Intuitively, triangles in a space are "slimmer" than corresponding "model triangles" in a standard space of constant curvature . In a space, the curvature is bounded from above by . A notable special case is ; complete spaces are known as "Hadamard spaces" after the French mathematician Jacques Hadamard.

In mathematics, the differential geometry of surfaces deals with the differential geometry of smooth surfaces with various additional structures, most often, a Riemannian metric. Surfaces have been extensively studied from various perspectives: extrinsically, relating to their embedding in Euclidean space and intrinsically, reflecting their properties determined solely by the distance within the surface as measured along curves on the surface. One of the fundamental concepts investigated is the Gaussian curvature, first studied in depth by Carl Friedrich Gauss, who showed that curvature was an intrinsic property of a surface, independent of its isometric embedding in Euclidean space.



























