In physics, coherence length is the propagation distance over which a coherent wave maintains a specified degree of coherence. Wave interference is strong when the paths taken by all of the interfering waves differ by less than the coherence length. A wave with a longer coherence length is closer to a perfect sinusoidal wave. Coherence length is important in holography and telecommunications engineering.
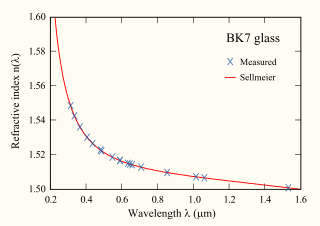
The Sellmeier equation is an empirical relationship between refractive index and wavelength for a particular transparent medium. The equation is used to determine the dispersion of light in the medium.

Dispersion is the phenomenon in which the phase velocity of a wave depends on its frequency. Sometimes the term chromatic dispersion is used for to refer to optics specifically, as opposed to wave propagation in general. A medium having this common property may be termed a dispersive medium.
The laser diode rate equations model the electrical and optical performance of a laser diode. This system of ordinary differential equations relates the number or density of photons and charge carriers (electrons) in the device to the injection current and to device and material parameters such as carrier lifetime, photon lifetime, and the optical gain.

Fused quartz, fused silica or quartz glass is a glass consisting of almost pure silica (silicon dioxide, SiO2) in amorphous (non-crystalline) form. This differs from all other commercial glasses, such as soda-lime glass, lead glass, or borosilicate glass, in which other ingredients are added which change the glasses' optical and physical properties, such as lowering the melt temperature, the spectral transmission range, or the mechanical strength. Fused quartz, therefore, has high working and melting temperatures, making it difficult to form and less desirable for most common applications, but is much stronger, more chemically resistant, and exhibits lower thermal expansion, making it more suitable for many specialized uses such as lighting and scientific applications.
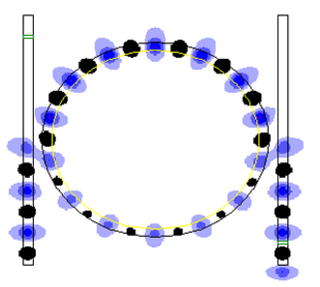
An optical ring resonator is a set of waveguides in which at least one is a closed loop coupled to some sort of light input and output. The concepts behind optical ring resonators are the same as those behind whispering galleries except that they use light and obey the properties behind constructive interference and total internal reflection. When light of the resonant wavelength is passed through the loop from the input waveguide, the light builds up in intensity over multiple round-trips owing to constructive interference and is output to the output bus waveguide which serves as a detector waveguide. Because only a select few wavelengths will be at resonance within the loop, the optical ring resonator functions as a filter. Additionally, as implied earlier, two or more ring waveguides can be coupled to each other to form an add/drop optical filter.
A spectroradiometer is a light measurement tool that is able to measure both the wavelength and amplitude of the light emitted from a light source. Spectrometers discriminate the wavelength based on the position the light hits at the detector array allowing the full spectrum to be obtained with a single acquisition. Most spectrometers have a base measurement of counts which is the un-calibrated reading and is thus impacted by the sensitivity of the detector to each wavelength. By applying a calibration, the spectrometer is then able to provide measurements of spectral irradiance, spectral radiance and/or spectral flux. This data is also then used with built in or PC software and numerous algorithms to provide readings or Irradiance (W/cm2), Illuminance, Radiance (W/sr), Luminance (cd), Flux, Chromaticity, Color Temperature, Peak and Dominant Wavelength. Some more complex spectrometer software packages also allow calculation of PAR μmol/m2/s, Metamerism, and candela calculations based on distance and include features like 2- and 20-degree observer, baseline overlay comparisons, transmission and reflectance.

An acousto-optic modulator (AOM), also called a Bragg cell or an acousto-optic deflector (AOD), uses the acousto-optic effect to diffract and shift the frequency of light using sound waves. They are used in lasers for Q-switching, telecommunications for signal modulation, and in spectroscopy for frequency control. A piezoelectric transducer is attached to a material such as glass. An oscillating electric signal drives the transducer to vibrate, which creates sound waves in the material. These can be thought of as moving periodic planes of expansion and compression that change the index of refraction. Incoming light scatters off the resulting periodic index modulation and interference occurs similar to Bragg diffraction. The interaction can be thought of as a three-wave mixing process resulting in sum-frequency generation or difference-frequency generation between phonons and photons.

A longitudinal mode of a resonant cavity is a particular standing wave pattern formed by waves confined in the cavity. The longitudinal modes correspond to the wavelengths of the wave which are reinforced by constructive interference after many reflections from the cavity's reflecting surfaces. All other wavelengths are suppressed by destructive interference.

A distributed Bragg reflector (DBR) is a reflector used in waveguides, such as optical fibers. It is a structure formed from multiple layers of alternating materials with different refractive index, or by periodic variation of some characteristic of a dielectric waveguide, resulting in periodic variation in the effective refractive index in the guide. Each layer boundary causes a partial reflection and refraction of an optical wave. For waves whose vacuum wavelength is close to four times the optical thickness of the layers, the interaction between these beams generates constructive interference, and the layers act as a high-quality reflector. The range of wavelengths that are reflected is called the photonic stopband. Within this range of wavelengths, light is "forbidden" to propagate in the structure.

An optical fiber, or optical fibre, is a flexible glass or plastic fiber that can transmit light from one end to the other. Such fibers find wide usage in fiber-optic communications, where they permit transmission over longer distances and at higher bandwidths than electrical cables. Fibers are used instead of metal wires because signals travel along them with less loss and are immune to electromagnetic interference. Fibers are also used for illumination and imaging, and are often wrapped in bundles so they may be used to carry light into, or images out of confined spaces, as in the case of a fiberscope. Specially designed fibers are also used for a variety of other applications, such as fiber optic sensors and fiber lasers.

In optics, a dispersive prism is an optical prism that is used to disperse light, that is, to separate light into its spectral components. Different wavelengths (colors) of light will be deflected by the prism at different angles. This is a result of the prism material's index of refraction varying with wavelength (dispersion). Generally, longer wavelengths (red) undergo a smaller deviation than shorter wavelengths (blue). The dispersion of white light into colors by a prism led Sir Isaac Newton to conclude that white light consisted of a mixture of different colors.

Athermalization, in the field of optics, is the process of achieving optothermal stability in optomechanical systems. This is done by minimizing variations in optical performance over a range of temperatures.

Acousto-optics is a branch of physics that studies the interactions between sound waves and light waves, especially the diffraction of laser light by ultrasound through an ultrasonic grating.
Volume holograms are holograms where the thickness of the recording material is much larger than the light wavelength used for recording. In this case diffraction of light from the hologram is possible only as Bragg diffraction, i.e., the light has to have the right wavelength (color) and the wave must have the right shape. Volume holograms are also called thick holograms or Bragg holograms.

The first description of multiple-prism arrays, and multiple-prism dispersion, was given by Newton in his book Opticks. Prism pair expanders were introduced by Brewster in 1813. A modern mathematical description of the single-prism dispersion was given by Born and Wolf in 1959. The generalized multiple-prism dispersion theory was introduced by Duarte and Piper in 1982.

PHOSFOS is a research and technology development project co-funded by the European Commission.

Multiple-prism grating laser oscillators, or MPG laser oscillators, use multiple-prism beam expansion to illuminate a diffraction grating mounted either in Littrow configuration or grazing-incidence configuration. Originally, these narrow-linewidth tunable dispersive oscillators were introduced as multiple-prism Littrow (MPL) grating oscillators, or hybrid multiple-prism near-grazing-incidence (HMPGI) grating cavities, in organic dye lasers. However, these designs were quickly adopted for other types of lasers such as gas lasers, diode lasers, and more recently fiber lasers.
Holographic optical element (HOE) is an optical component (mirror, lens, directional diffuser, etc.) that produces holographic images using principles of diffraction. HOE is most commonly used in transparent displays, 3D imaging, and certain scanning technologies. The shape and structure of the HOE is dependent on the piece of hardware it is needed for, and the coupled wave theory is a common tool used to calculate the diffraction efficiency or grating volume that helps with the design of an HOE. Early concepts of the holographic optical element can be traced back to the mid-1900s, coinciding closely with the start of holography coined by Dennis Gabor. The application of 3D visualization and displays is ultimately the end goal of the HOE; however, the cost and complexity of the device has hindered the rapid development toward full 3D visualization. The HOE is also used in the development of augmented reality(AR) by companies such as Google with Google Glass or in research universities that look to utilize HOEs to create 3D imaging without the use of eye-wear or head-wear. Furthermore, the ability of the HOE to allow for transparent displays have caught the attention of the US military in its development of better head-up displays (HUD) which is used to display crucial information for aircraft pilots.

An addressed fiber Bragg structure (AFBS) is a fiber Bragg grating, the optical frequency response of which includes two narrowband components with the frequency spacing between them being in the radio frequency (RF) range. The frequency spacing is unique for every AFBS in the interrogation circuit and does not change when the AFBS is subjected to strain or temperature variation. An addressed fiber Bragg structure can perform triple function in fiber-optic sensor systems: a sensor, a shaper of double-frequency probing radiation, and a multiplexor. The key feature of AFBS is that it enables the definition of its central wavelength without scanning its spectral response, as opposed to conventional fiber Bragg gratings (FBG), which are probed using optoelectronic interrogators. An interrogation circuit of AFBS is significantly simplified in comparison with conventional interrogators and consists of a broadband optical source, an optical filter with a predefined linear inclined frequency response, and a photodetector. The AFBS interrogation principle intrinsically allows to include several AFBSs with the same central wavelength and different address frequencies into a single measurement system.

















































