
In mathematics, a dynamical system is a system in which a function describes the time dependence of a point in an ambient space, such as in a parametric curve. Examples include the mathematical models that describe the swinging of a clock pendulum, the flow of water in a pipe, the random motion of particles in the air, and the number of fish each springtime in a lake. The most general definition unifies several concepts in mathematics such as ordinary differential equations and ergodic theory by allowing different choices of the space and how time is measured. Time can be measured by integers, by real or complex numbers or can be a more general algebraic object, losing the memory of its physical origin, and the space may be a manifold or simply a set, without the need of a smooth space-time structure defined on it.

Interplanetary spaceflight or interplanetary travel is the crewed or uncrewed travel between stars and planets, usually within a single planetary system. In practice, spaceflights of this type are confined to travel between the planets of the Solar System. Uncrewed space probes have flown to all the observed planets in the Solar System as well as to dwarf planets Pluto and Ceres, and several asteroids. Orbiters and landers return more information than fly-by missions. Crewed flights have landed on the Moon and have been planned, from time to time, for Mars, Venus and Mercury. While many scientists appreciate the knowledge value that uncrewed flights provide, the value of crewed missions is more controversial. Science fiction writers propose a number of benefits, including the mining of asteroids, access to solar power, and room for colonization in the event of an Earth catastrophe.
The Kolmogorov–Arnold–Moser (KAM) theorem is a result in dynamical systems about the persistence of quasiperiodic motions under small perturbations. The theorem partly resolves the small-divisor problem that arises in the perturbation theory of classical mechanics.

In celestial mechanics, the Lagrange points are points of equilibrium for small-mass objects under the gravitational influence of two massive orbiting bodies. Mathematically, this involves the solution of the restricted three-body problem.

A gravity assist, gravity assist maneuver, swing-by, or generally a gravitational slingshot in orbital mechanics, is a type of spaceflight flyby which makes use of the relative movement and gravity of a planet or other astronomical object to alter the path and speed of a spacecraft, typically to save propellant and reduce expense.

The Interplanetary Transport Network (ITN) is a collection of gravitationally determined pathways through the Solar System that require very little energy for an object to follow. The ITN makes particular use of Lagrange points as locations where trajectories through space can be redirected using little or no energy. These points have the peculiar property of allowing objects to orbit around them, despite lacking an object to orbit, as these points exist where gravitational forces between two celestial bodies are equal. While it would use little energy, transport along the network would take a long time.
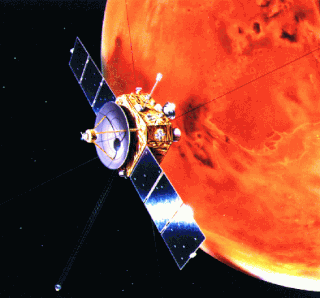
Nozomi was a Japanese Mars orbiter that failed to reach Mars due to electrical failure. It was constructed by the Institute of Space and Astronautical Science, University of Tokyo and launched on July 4, 1998, at 03:12 JST with an on-orbit dry mass of 258 kg and 282 kg of propellant. The Nozomi mission was terminated on December 31, 2003.

Janice Elaine Voss was an American engineer and a NASA astronaut. Voss received her B.S. in engineering science from Purdue University, her M.S. in electrical engineering from MIT, and her PhD in aeronautics and astronautics from MIT. She flew in space five times, jointly holding the record for American women. Voss died in Arizona on February 6, 2012, from breast cancer.

BepiColombo is a joint mission of the European Space Agency (ESA) and the Japan Aerospace Exploration Agency (JAXA) to the planet Mercury. The mission comprises two satellites launched together: the Mercury Planetary Orbiter (MPO) and Mio. The mission will perform a comprehensive study of Mercury, including characterization of its magnetic field, magnetosphere, and both interior and surface structure. It was launched on an Ariane 5 rocket on 20 October 2018 at 01:45 UTC, with an arrival at Mercury planned for November 2026, after a flyby of Earth, two flybys of Venus, and six flybys of Mercury. The mission was approved in November 2009, after years in proposal and planning as part of the European Space Agency's Horizon 2000+ programme; it is the last mission of the programme to be launched.
In astrodynamics, orbital station-keeping is keeping a spacecraft at a fixed distance from another spacecraft or celestial body. It requires a series of orbital maneuvers made with thruster burns to keep the active craft in the same orbit as its target. For many low Earth orbit satellites, the effects of non-Keplerian forces, i.e. the deviations of the gravitational force of the Earth from that of a homogeneous sphere, gravitational forces from Sun/Moon, solar radiation pressure and air drag, must be counteracted. For spacecraft in a halo orbit around a Lagrange point, station-keeping is even more fundamental, as such an orbit is unstable; without an active control with thruster burns, the smallest deviation in position or velocity would result in the spacecraft leaving orbit completely.

Martin Wen-Yu Lo is an American mathematician who currently works as a spacecraft trajectory expert at the NASA-owned Jet Propulsion Laboratory. Martin Lo is well known for discovering the Interplanetary Superhighway, also known as the Interplanetary Transport Network. The superhighway is created by combined gravitational forces of several planets that connects planets by a network of “tunnels” and is the most efficient way to navigate the solar system. This continues to be his main area of research.
Institute of Space and Astronautical Science, or ISAS, is a Japanese national research organization of astrophysics using rockets, astronomical satellites and interplanetary probes which played a major role in Japan's space development. Established as part of the University of Tokyo in 1964, the institute spun off from the university to come under direct purview of the Ministry of Education. Since 2003, it is a division of Japan Aerospace Exploration Agency (JAXA).

A low-energy transfer, or low-energy trajectory, is a route in space that allows spacecraft to change orbits using significantly less fuel than traditional transfers. These routes work in the Earth–Moon system and also in other systems, such as between the moons of Jupiter. The drawback of such trajectories is that they take longer to complete than higher-energy (more-fuel) transfers, such as Hohmann transfer orbits.

In orbital mechanics, a Lissajous orbit, named after Jules Antoine Lissajous, is a quasi-periodic orbital trajectory that an object can follow around a Lagrangian point of a three-body system with minimal propulsion. Lyapunov orbits around a Lagrangian point are curved paths that lie entirely in the plane of the two primary bodies. In contrast, Lissajous orbits include components in this plane and perpendicular to it, and follow a Lissajous curve. Halo orbits also include components perpendicular to the plane, but they are periodic, while Lissajous orbits are usually not.

A halo orbit is a periodic, three-dimensional orbit associated with one of the L1, L2 or L3 Lagrange points in the three-body problem of orbital mechanics. Although a Lagrange point is just a point in empty space, its peculiar characteristic is that it can be orbited by a Lissajous orbit or by a halo orbit. These can be thought of as resulting from an interaction between the gravitational pull of the two planetary bodies and the Coriolis and centrifugal force on a spacecraft. Halo orbits exist in any three-body system, e.g., a Sun–Earth–orbiting satellite system or an Earth–Moon–orbiting satellite system. Continuous "families" of both northern and southern halo orbits exist at each Lagrange point. Because halo orbits tend to be unstable, station-keeping using thrusters may be required to keep a satellite on the orbit.

Aditya-L1 is a coronagraphy spacecraft for studying the solar atmosphere, designed and developed by the Indian Space Research Organisation (ISRO) and various other Indian Space Research Institutes. It is orbiting at about 1.5 million km from Earth in a halo orbit around the Lagrange point 1 (L1) between the Earth and the Sun, where it will study the solar atmosphere, solar magnetic storms, and their impact on the environment around the Earth.
OKEANOS was a proposed mission concept to Trojan asteroids, which share Jupiter's orbit, using a hybrid solar sail for propulsion; the sail was planned to be covered with thin solar panels to power an ion engine. In situ analysis of the collected samples would have been performed by either direct contact or using a lander carrying a high-resolution mass spectrometer. A sample-return to Earth was an option under study.

DESTINY+ (Demonstration and Experiment of Space Technology for INterplanetary voYage with Phaethon fLyby and dUst Science) is a planned mission to fly by the Geminids meteor shower parent body 3200 Phaethon, and sample dust originating from the "rock comet". The spacecraft is being developed by the Japanese space agency JAXA and will demonstrate advanced technologies for future deep space exploration. As of October 2024, DESTINY+ is planned to be launched in fiscal year 2028.

The Power and Propulsion Element (PPE), previously known as the Asteroid Redirect Vehicle propulsion system, is a planned solar electric ion propulsion module being developed by Maxar Technologies for NASA. It is one of the major components of the Lunar Gateway. The PPE will allow access to the entire lunar surface and a wide range of lunar orbits and double as a space tug for visiting craft.















