| Kauaiina rubropulverula | |
|---|---|
Scientific classification  | |
| Domain: | Eukaryota |
| Kingdom: | Animalia |
| Phylum: | Arthropoda |
| Class: | Insecta |
| Order: | Lepidoptera |
| Family: | Geometridae |
| Genus: | Kauaiina |
| Species: | K. rubropulverula |
| Binomial name | |
| Kauaiina rubropulverula Riotte, 1989 | |
Kauaiina rubropulverula is a moth of the family Geometridae first described by Jules C. E. Riotte in 1989. [1] It is endemic to Hawaii.
In physics, the kinetic energy of an object is the form of energy that it possesses due to its motion.
L'Hôpital's rule, also known as Bernoulli's rule, is a mathematical theorem that allows evaluating limits of indeterminate forms using derivatives. Application of the rule often converts an indeterminate form to an expression that can be easily evaluated by substitution. The rule is named after the 17th-century French mathematician Guillaume De l'Hôpital. Although the rule is often attributed to De l'Hôpital, the theorem was first introduced to him in 1694 by the Swiss mathematician Johann Bernoulli.
In mathematics, the mean value theorem states, roughly, that for a given planar arc between two endpoints, there is at least one point at which the tangent to the arc is parallel to the secant through its endpoints. It is one of the most important results in real analysis. This theorem is used to prove statements about a function on an interval starting from local hypotheses about derivatives at points of the interval.
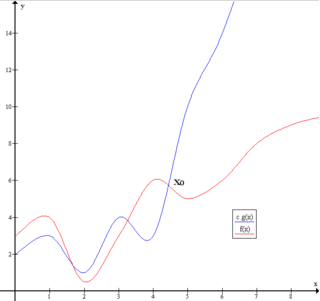
Big O notation is a mathematical notation that describes the limiting behavior of a function when the argument tends towards a particular value or infinity. Big O is a member of a family of notations invented by German mathematicians Paul Bachmann, Edmund Landau, and others, collectively called Bachmann–Landau notation or asymptotic notation. The letter O was chosen by Bachmann to stand for Ordnung, meaning the order of approximation.
Some Buddhist terms and concepts lack direct translations into English that cover the breadth of the original term. Below are given a number of important Buddhist terms, short definitions, and the languages in which they appear. In this list, an attempt has been made to organize terms by their original form and give translations and synonyms in other languages along with the definition.
This gallery of sovereign state flags shows the national or state flags of sovereign states that appear on the list of sovereign states. For flags of other entities, please see gallery of flags of dependent territories. Each flag is depicted as if the flagpole is positioned on the left of the flag, except for those of Iran, Iraq and Saudi Arabia which are depicted with the hoist to the right.
A commitment scheme is a cryptographic primitive that allows one to commit to a chosen value while keeping it hidden to others, with the ability to reveal the committed value later. Commitment schemes are designed so that a party cannot change the value or statement after they have committed to it: that is, commitment schemes are binding. Commitment schemes have important applications in a number of cryptographic protocols including secure coin flipping, zero-knowledge proofs, and secure computation.
Kauaiina is a genus of moths in the family Geometridae erected by Jules C. E. Riotte in 1978.
Kauaiina ioxantha is a moth of the family Geometridae. It was first described by Edward Meyrick in 1899. It is endemic to the Hawaiian island of Kauai.
Kauaiina parva is a moth of the family Geometridae first described by Jules C. E. Riotte in 1980. It is endemic to the eastern part of the Hawaiian island of Maui.
Kauaiina alakaii is a moth of the family Geometridae first described by Jules C. E. Riotte in 1979. It is endemic to the eastern part of the Hawaiian island of Kauai, where it was collected in the Alakai Swamp, after which it is named.
Kauaiina molokaiensis is a moth of the family Geometridae first described by Jules C. E. Riotte in 1979. It is endemic to the Hawaiian island of Molokai, where it was at an altitude of 1,290 meters above Puu Kolekole.
Kauaiina montgomeryi is a moth of the family Geometridae first described by Jules C. E. Riotte in 1978. It is endemic to the eastern part of the Hawaiian island of Maui.
Kauaiina howarthi is a moth of the family Geometridae first described by Jules C. E. Riotte in 1990. It is endemic to Hawaii.
Xanthorhoini is a tribe of geometer moths under subfamily Larentiinae. The tribe was described by Pierce in 1914.
A generative adversarial network (GAN) is a class of machine learning frameworks and a prominent framework for approaching generative AI. The concept was initially developed by Ian Goodfellow and his colleagues in June 2014. In a GAN, two neural networks contest with each other in the form of a zero-sum game, where one agent's gain is another agent's loss.