
In mathematics, a projective plane is a geometric structure that extends the concept of a plane. In the ordinary Euclidean plane, two lines typically intersect in a single point, but there are some pairs of lines that do not intersect. A projective plane can be thought of as an ordinary plane equipped with additional "points at infinity" where parallel lines intersect. Thus any two distinct lines in a projective plane intersect at exactly one point.
In combinatorial mathematics, a Steiner system is a type of block design, specifically a t-design with λ = 1 and t = 2 or (recently) t ≥ 2.

In mathematics, the concept of a projective space originated from the visual effect of perspective, where parallel lines seem to meet at infinity. A projective space may thus be viewed as the extension of a Euclidean space, or, more generally, an affine space with points at infinity, in such a way that there is one point at infinity of each direction of parallel lines.
A finite geometry is any geometric system that has only a finite number of points. The familiar Euclidean geometry is not finite, because a Euclidean line contains infinitely many points. A geometry based on the graphics displayed on a computer screen, where the pixels are considered to be the points, would be a finite geometry. While there are many systems that could be called finite geometries, attention is mostly paid to the finite projective and affine spaces because of their regularity and simplicity. Other significant types of finite geometry are finite Möbius or inversive planes and Laguerre planes, which are examples of a general type called Benz planes, and their higher-dimensional analogs such as higher finite inversive geometries.

In projective geometry, Desargues's theorem, named after Girard Desargues, states:

In mathematics, especially in the group theoretic area of algebra, the projective linear group is the induced action of the general linear group of a vector space V on the associated projective space P(V). Explicitly, the projective linear group is the quotient group

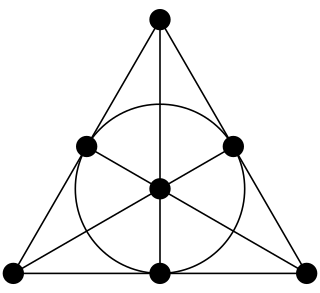
In finite geometry, the Fano plane is the finite projective plane of order 2. It is the finite projective plane with the smallest possible number of points and lines: 7 points and 7 lines, with 3 points on every line and 3 lines through every point. The standard notation for this plane, as a member of a family of projective spaces, is PG(2, 2) where PG stands for "projective geometry", the first parameter is the geometric dimension and the second parameter is the order.
In geometry, an affine plane is a system of points and lines that satisfy the following axioms:

In mathematics, an incidence structure is an abstract system consisting of two types of objects and a single relationship between these types of objects. Consider the points and lines of the Euclidean plane as the two types of objects and ignore all the properties of this geometry except for the relation of which points are on which lines for all points and lines. What is left is the incidence structure of the Euclidean plane.

The Sylvester–Gallai theorem in geometry states that every finite set of points in the Euclidean plane has a line that passes through exactly two of the points or a line that passes through all of them. It is named after James Joseph Sylvester, who posed it as a problem in 1893, and Tibor Gallai, who published one of the first proofs of this theorem in 1944.
In combinatorial mathematics, a block design is an incidence structure consisting of a set together with a family of subsets known as blocks, chosen such that frequency of the elements satisfies certain conditions making the collection of blocks exhibit symmetry (balance). They have applications in many areas, including experimental design, finite geometry, physical chemistry, software testing, cryptography, and algebraic geometry.

In mathematics, incidence geometry is the study of incidence structures. A geometric structure such as the Euclidean plane is a complicated object that involves concepts such as length, angles, continuity, betweenness, and incidence. An incidence structure is what is obtained when all other concepts are removed and all that remains is the data about which points lie on which lines. Even with this severe limitation, theorems can be proved and interesting facts emerge concerning this structure. Such fundamental results remain valid when additional concepts are added to form a richer geometry. It sometimes happens that authors blur the distinction between a study and the objects of that study, so it is not surprising to find that some authors refer to incidence structures as incidence geometries.
Combinatorial design theory is the part of combinatorial mathematics that deals with the existence, construction and properties of systems of finite sets whose arrangements satisfy generalized concepts of balance and/or symmetry. These concepts are not made precise so that a wide range of objects can be thought of as being under the same umbrella. At times this might involve the numerical sizes of set intersections as in block designs, while at other times it could involve the spatial arrangement of entries in an array as in sudoku grids.
A logical matrix, binary matrix, relation matrix, Boolean matrix, or (0, 1) matrix is a matrix with entries from the Boolean domain B = {0, 1}. Such a matrix can be used to represent a binary relation between a pair of finite sets.
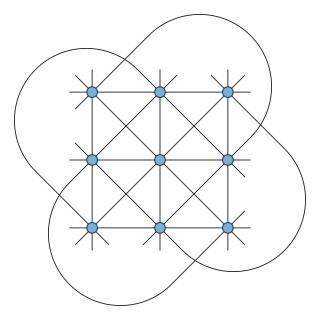
In geometry, the Hesse configuration, introduced by Colin Maclaurin and studied by Hesse (1844), is a configuration of 9 points and 12 lines with three points per line and four lines through each point. It can be realized in the complex projective plane as the set of inflection points of an elliptic curve, but it has no realization in the Euclidean plane.

In mathematics, the Cremona–Richmond configuration is a configuration of 15 lines and 15 points, having 3 points on each line and 3 lines through each point, and containing no triangles. It was studied by Cremona (1877) and Richmond (1900). It is a generalized quadrangle with parameters (2,2). Its Levi graph is the Tutte–Coxeter graph.
In geometry, a unital is a set of n3 + 1 points arranged into subsets of size n + 1 so that every pair of distinct points of the set are contained in exactly one subset. n ≥ 3 is required by some authors to avoid small exceptional cases. This is equivalent to saying that a unital is a 2-(n3 + 1, n + 1, 1) block design. Some unitals may be embedded in a projective plane of order n2. In this case of embedded unitals, every line of the plane intersects the unital in either 1 or n + 1 points. In the Desarguesian planes, PG(2,q2), the classical examples of unitals are given by nondegenerate Hermitian curves. There are also many non-classical examples. The first and the only known unital with non prime power parameters, n=6, was constructed by Bhaskar Bagchi and Sunanda Bagchi. It is still unknown if this unital can be embedded in a projective plane of order 36, if such a plane exists.
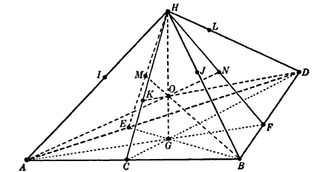
In finite geometry, PG(3,2) is the smallest three-dimensional projective space. It can be thought of as an extension of the Fano plane. It has 15 points, 35 lines, and 15 planes. It also has the following properties:
In geometry, a truncated projective plane (TPP), also known as a dual affine plane, is a special kind of a hypergraph or geometric configuration that is constructed in the following way.
In finite geometry, Lam's problem is the problem of determining if a finite projective plane of order ten exists. The order ten case is the first theoretically uncertain case, as all smaller orders can be resolved by purely theoretical means. Lam's problem is named after Clement W. H. Lam who experimentally determined that projective planes of order ten do not exist via exhaustive computational searches.












