A line group is a mathematical way of describing symmetries associated with moving along a line. These symmetries include repeating along that line, making that line a one-dimensional lattice. However, line groups may have more than one dimension, and they may involve those dimensions in its isometries or symmetry transformations.
One constructs a line group by taking a point group in the full dimensions of the space, and then adding translations or offsets along the line to each of the point group's elements, in the fashion of constructing a space group. These offsets include the repeats, and a fraction of the repeat, one fraction for each element. For convenience, the fractions are scaled to the size of the repeat; they are thus within the line's unit cell segment.
There are 2 one-dimensional line groups. They are the infinite limits of the discrete two-dimensional point groups Cn and Dn:
| Notations | Description | Example | |||
|---|---|---|---|---|---|
| Intl | Orbifold | Coxeter | P.G. | ||
| p1 | ∞∞ | [∞]+ | C∞ | Translations. Abstract group Z, the integers under addition | ... --> --> --> --> ... |
| p1m | *∞∞ | [∞] | D∞ | Reflections. Abstract group Dih∞, the infinite dihedral group | ... --> <-- --> <-- ... |
There are 7 frieze groups, which involve reflections along the line, reflections perpendicular to the line, and 180° rotations in the two dimensions.
| IUC | Orbifold | Schönflies | Conway | Coxeter | Fundamental domain |
|---|---|---|---|---|---|
| p1 | ∞∞ | C∞ | C∞ | [∞,1]+ |  |
| p1m1 | *∞∞ | C∞v | CD2∞ | [∞,1] |  |
| p11g | ∞x | S2∞ | CC2∞ | [∞+,2+] |  |
| p11m | ∞* | C∞h | ±C∞ | [∞+,2] |  |
| p2 | 22∞ | D∞ | D2∞ | [∞,2]+ |  |
| p2mg | 2*∞ | D∞d | DD4∞ | [∞,2+] |  |
| p2mm | *22∞ | D∞h | ±D2∞ | [∞,2] |  |
There are 13 infinite families of three-dimensional line groups, [1] derived from the 7 infinite families of axial three-dimensional point groups. As with space groups in general, line groups with the same point group can have different patterns of offsets. Each of the families is based on a group of rotations around the axis with order n. The groups are listed in Hermann-Mauguin notation, and for the point groups, Schönflies notation. There appears to be no comparable notation for the line groups. These groups can also be interpreted as patterns of wallpaper groups [2] wrapped around a cylinder n times and infinitely repeating along the cylinder's axis, much like the three-dimensional point groups and the frieze groups. A table of these groups:
| Point group | Line group | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| H-M | Schönf. | Orb. | Cox. | H-M | Offset type | Wallpaper | Coxeter [∞h,2,pv] | ||||
| Even n | Odd n | Even n | Odd n | IUC | Orbifold | Diagram | |||||
| n | Cn | nn | [n]+ | Pnq | Helical: q | p1 | o |  | [∞+,2,n+] | ||
| 2n | n | S2n | n× | [2+,2n+] | P2n | Pn | None | p11g, pg(h) | ×× |  | [(∞,2)+,2n+] |
| n/m | 2n | Cnh | n* | [2,n+] | Pn/m | P2n | None | p11m, pm(h) | ** |  | [∞+,2,n] |
| 2n/m | C2nh | (2n)* | [2,2n+] | P2nn/m | Zigzag | c11m, cm(h) | *× |  | [∞+,2+,2n] | ||
| nmm | nm | Cnv | *nn | [n] | Pnmm | Pnm | None | p1m1, pm(v) | ** |  | [∞,2,n+] |
| Pncc | Pnc | None | p1g1, pg(v) | ×× |  | [∞+,(2,n)+] | |||||
| 2nmm | C2nv | *(2n)(2n) | [2n] | P2nnmc | Zigzag | c1m1, cm(v) | *× |  | [∞,2+,2n+] | ||
| n22 | n2 | Dn | n22 | [2,n]+ | Pnq22 | Pnq2 | Helical: q | p2 | 2222 |  | [∞,2,n]+ |
| 2n2m | nm | Dnd | 2*n | [2+,2n] | P2n2m | Pnm | None | p2gm, pmg(v) | 22* |  | [(∞,2)+,2n] |
| P2n2c | Pnc | None | p2gg, pgg | 22× |  | [+(∞,(2),2n)+] | |||||
| n/mmm | 2n2m | Dnh | *n22 | [2,n] | Pn/mmm | P2n2m | None | p2mm, pmm | *2222 |  | [∞,2,n] |
| Pn/mcc | P2n2c | None | p2mg, pmg(h) | 22* |  | [∞,(2,n)+] | |||||
| 2n/mmm | D2nh | *(2n)22 | [2,2n] | P2nn/mcm | Zigzag | c2mm, cmm | 2*22 |  | [∞,2+,2n] | ||
The offset types are:
Note that the wallpaper groups pm, pg, cm, and pmg appear twice. Each appearance has a different orientation relative to the line-group axis; reflection parallel (h) or perpendicular (v). The other groups have no such orientation: p1, p2, pmm, pgg, cmm.
If the point group is constrained to be a crystallographic point group, a symmetry of some three-dimensional lattice, then the resulting line group is called a rod group. There are 75 rod groups.
Going to the continuum limit, with n to ∞, the possible point groups become C∞, C∞h, C∞v, D∞, and D∞h, and the line groups have the appropriate possible offsets, with the exception of zigzag.
The groups Cn(q) and Dn(q) express the symmetries of helical objects. Cn(q) is for n helices oriented in the same direction, while Dn(q) is for n unoriented helices and 2n helices with alternating orientations. Reversing the sign of q creates a mirror image, reversing the helices' chirality or handedness.
Nucleic acids, DNA and RNA, are well known for their helical symmetry. Nucleic acids have a well-defined direction, giving single strands C1(q). Double strands have opposite directions and are on opposite sides of the helix axis, giving them D1(q).
In group theory, the symmetry group of a geometric object is the group of all transformations under which the object is invariant, endowed with the group operation of composition. Such a transformation is an invertible mapping of the ambient space which takes the object to itself, and which preserves all the relevant structure of the object. A frequent notation for the symmetry group of an object X is G = Sym(X).
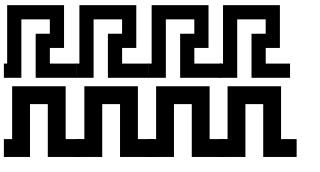
In mathematics, a frieze or frieze pattern is a two-dimensional design that repeats in one direction. Such patterns occur frequently in architecture and decorative art. Frieze patterns can be classified into seven types according to their symmetries. The set of symmetries of a frieze pattern is called a frieze group.
A wallpaper is a mathematical object covering a whole Euclidean plane by repeating a motif indefinitely, in manner that certain isometries keep the drawing unchanged. For each wallpaper there corresponds a group of congruent transformations, with function composition as the group operation. Thus, a wallpaper group is a mathematical classification of a two‑dimensional repetitive pattern, based on the symmetries in the pattern. Such patterns occur frequently in architecture and decorative art, especially in textiles, tessellations, tiles and physical wallpaper.
In 2-dimensional geometry, a glide reflection is a symmetry operation that consists of a reflection over a line and then translation along that line, combined into a single operation. The intermediate step between reflection and translation can look different from the starting configuration, so objects with glide symmetry are in general, not symmetrical under reflection alone. In group theory, the glide plane is classified as a type of opposite isometry of the Euclidean plane.
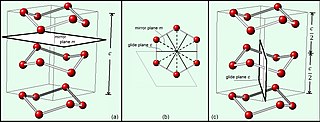
In mathematics, physics and chemistry, a space group is the symmetry group of a repeating pattern in space, usually in three dimensions. The elements of a space group are the rigid transformations of the pattern that leave it unchanged. In three dimensions, space groups are classified into 219 distinct types, or 230 types if chiral copies are considered distinct. Space groups are discrete cocompact groups of isometries of an oriented Euclidean space in any number of dimensions. In dimensions other than 3, they are sometimes called Bieberbach groups.
In geometry, the Schläfli symbol is a notation of the form that defines regular polytopes and tessellations.
In crystallography, a crystallographic point group is a set of symmetry operations, corresponding to one of the point groups in three dimensions, such that each operation would leave the structure of a crystal unchanged i.e. the same kinds of atoms would be placed in similar positions as before the transformation. For example, in many crystals in the cubic crystal system, a rotation of the unit cell by 90 degrees around an axis that is perpendicular to one of the faces of the cube is a symmetry operation that moves each atom to the location of another atom of the same kind, leaving the overall structure of the crystal unaffected.
Rotational symmetry, also known as radial symmetry in geometry, is the property a shape has when it looks the same after some rotation by a partial turn. An object's degree of rotational symmetry is the number of distinct orientations in which it looks exactly the same for each rotation.
The Schoenfliesnotation, named after the German mathematician Arthur Moritz Schoenflies, is a notation primarily used to specify point groups in three dimensions. Because a point group alone is completely adequate to describe the symmetry of a molecule, the notation is often sufficient and commonly used for spectroscopy. However, in crystallography, there is additional translational symmetry, and point groups are not enough to describe the full symmetry of crystals, so the full space group is usually used instead. The naming of full space groups usually follows another common convention, the Hermann–Mauguin notation, also known as the international notation.
In geometry, a point group is a mathematical group of symmetry operations (isometries in a Euclidean space) that have a fixed point in common. The coordinate origin of the Euclidean space is conventionally taken to be a fixed point, and every point group in dimension d is then a subgroup of the orthogonal group O(d). Point groups are used to describe the symmetries of geometric figures and physical objects such as molecules.
In geometry, a point group in three dimensions is an isometry group in three dimensions that leaves the origin fixed, or correspondingly, an isometry group of a sphere. It is a subgroup of the orthogonal group O(3), the group of all isometries that leave the origin fixed, or correspondingly, the group of orthogonal matrices. O(3) itself is a subgroup of the Euclidean group E(3) of all isometries.
In geometry, orbifold notation is a system, invented by the mathematician William Thurston and promoted by John Conway, for representing types of symmetry groups in two-dimensional spaces of constant curvature. The advantage of the notation is that it describes these groups in a way which indicates many of the groups' properties: in particular, it follows William Thurston in describing the orbifold obtained by taking the quotient of Euclidean space by the group under consideration.
In geometry, dihedral symmetry in three dimensions is one of three infinite sequences of point groups in three dimensions which have a symmetry group that as an abstract group is a dihedral group Dihn.
In three dimensional geometry, there are four infinite series of point groups in three dimensions (n≥1) with n-fold rotational or reflectional symmetry about one axis (by an angle of 360°/n) that does not change the object.
In geometry, a two-dimensional point group or rosette group is a group of geometric symmetries (isometries) that keep at least one point fixed in a plane. Every such group is a subgroup of the orthogonal group O(2), including O(2) itself. Its elements are rotations and reflections, and every such group containing only rotations is a subgroup of the special orthogonal group SO(2), including SO(2) itself. That group is isomorphic to R/Z and the first unitary group, U(1), a group also known as the circle group.
A fixed point of an isometry group is a point that is a fixed point for every isometry in the group. For any isometry group in Euclidean space the set of fixed points is either empty or an affine space.
The Boerdijk–Coxeter helix, named after H. S. M. Coxeter and A. H. Boerdijk, is a linear stacking of regular tetrahedra, arranged so that the edges of the complex that belong to only one tetrahedron form three intertwined helices. There are two chiral forms, with either clockwise or counterclockwise windings. Unlike any other stacking of Platonic solids, the Boerdijk–Coxeter helix is not rotationally repetitive in 3-dimensional space. Even in an infinite string of stacked tetrahedra, no two tetrahedra will have the same orientation, because the helical pitch per cell is not a rational fraction of the circle. However, modified forms of this helix have been found which are rotationally repetitive, and in 4-dimensional space this helix repeats in rings of exactly 30 tetrahedral cells that tessellate the 3-sphere surface of the 600-cell, one of the six regular convex polychora.
In geometry, an object has symmetry if there is an operation or transformation that maps the figure/object onto itself. Thus, a symmetry can be thought of as an immunity to change. For instance, a circle rotated about its center will have the same shape and size as the original circle, as all points before and after the transform would be indistinguishable. A circle is thus said to be symmetric under rotation or to have rotational symmetry. If the isometry is the reflection of a plane figure about a line, then the figure is said to have reflectional symmetry or line symmetry; it is also possible for a figure/object to have more than one line of symmetry.
In geometry, an infinite skew polygon or skew apeirogon is an infinite 2-polytope with vertices that are not all colinear. Infinite zig-zag skew polygons are 2-dimensional infinite skew polygons with vertices alternating between two parallel lines. Infinite helical polygons are 3-dimensional infinite skew polygons with vertices on the surface of a cylinder.