Stuart Alan Kauffman is an American medical doctor, theoretical biologist, and complex systems researcher who studies the origin of life on Earth. He was a professor at the University of Chicago, University of Pennsylvania, and University of Calgary. He is currently emeritus professor of biochemistry at the University of Pennsylvania and affiliate faculty at the Institute for Systems Biology. He has a number of awards including a MacArthur Fellowship and a Wiener Medal.

In condensed matter physics, a spin glass is a magnetic state characterized by randomness, besides cooperative behavior in freezing of spins at a temperature called "freezing temperature" Tf. In ferromagnetic solids, component atoms' magnetic spins all align in the same direction. Spin glass when contrasted with a ferromagnet is defined as "disordered" magnetic state in which spins are aligned randomly or without a regular pattern and the couplings too are random.

In probability theory and statistics, the gamma distribution is a versatile two-parameter family of continuous probability distributions. The exponential distribution, Erlang distribution, and chi-squared distribution are special cases of the gamma distribution. There are two equivalent parameterizations in common use:
- With a shape parameter k and a scale parameter θ
- With a shape parameter and an inverse scale parameter , called a rate parameter.
The Ising model, named after the physicists Ernst Ising and Wilhelm Lenz, is a mathematical model of ferromagnetism in statistical mechanics. The model consists of discrete variables that represent magnetic dipole moments of atomic "spins" that can be in one of two states. The spins are arranged in a graph, usually a lattice, allowing each spin to interact with its neighbors. Neighboring spins that agree have a lower energy than those that disagree; the system tends to the lowest energy but heat disturbs this tendency, thus creating the possibility of different structural phases. The model allows the identification of phase transitions as a simplified model of reality. The two-dimensional square-lattice Ising model is one of the simplest statistical models to show a phase transition.
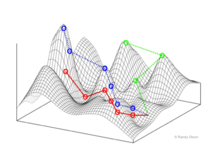
In evolutionary biology, fitness landscapes or adaptive landscapes are used to visualize the relationship between genotypes and reproductive success. It is assumed that every genotype has a well-defined replication rate. This fitness is the "height" of the landscape. Genotypes which are similar are said to be "close" to each other, while those that are very different are "far" from each other. The set of all possible genotypes, their degree of similarity, and their related fitness values is then called a fitness landscape. The idea of a fitness landscape is a metaphor to help explain flawed forms in evolution by natural selection, including exploits and glitches in animals like their reactions to supernormal stimuli.
A conformal field theory (CFT) is a quantum field theory that is invariant under conformal transformations. In two dimensions, there is an infinite-dimensional algebra of local conformal transformations, and conformal field theories can sometimes be exactly solved or classified.
In physics and probability theory, Mean-field theory (MFT) or Self-consistent field theory studies the behavior of high-dimensional random (stochastic) models by studying a simpler model that approximates the original by averaging over degrees of freedom. Such models consider many individual components that interact with each other.

Haldane's dilemma, also known as the waiting time problem, is a limit on the speed of beneficial evolution, calculated by J. B. S. Haldane in 1957. Before the invention of DNA sequencing technologies, it was not known how much polymorphism DNA harbored, although alloenzymes were beginning to make it clear that substantial polymorphism existed. This was puzzling because the amount of polymorphism known to exist seemed to exceed the theoretical limits that Haldane calculated, that is, the limits imposed if polymorphisms present in the population generally influence an organism's fitness. Motoo Kimura's landmark paper on neutral theory in 1968 built on Haldane's work to suggest that most molecular evolution is neutral, resolving the dilemma. Although neutral evolution remains the consensus theory among modern biologists, and thus Kimura's resolution of Haldane's dilemma is widely regarded as correct, some biologists argue that adaptive evolution explains a large fraction of substitutions in protein coding sequence, and they propose alternative solutions to Haldane's dilemma.
The classical XY model is a lattice model of statistical mechanics. In general, the XY model can be seen as a specialization of Stanley's n-vector model for n = 2.
In the theory of evolution and natural selection, the Price equation describes how a trait or allele changes in frequency over time. The equation uses a covariance between a trait and fitness, to give a mathematical description of evolution and natural selection. It provides a way to understand the effects that gene transmission and natural selection have on the frequency of alleles within each new generation of a population. The Price equation was derived by George R. Price, working in London to re-derive W.D. Hamilton's work on kin selection. Examples of the Price equation have been constructed for various evolutionary cases. The Price equation also has applications in economics.
In statistical mechanics, the Potts model, a generalization of the Ising model, is a model of interacting spins on a crystalline lattice. By studying the Potts model, one may gain insight into the behaviour of ferromagnets and certain other phenomena of solid-state physics. The strength of the Potts model is not so much that it models these physical systems well; it is rather that the one-dimensional case is exactly solvable, and that it has a rich mathematical formulation that has been studied extensively.
In statistical mechanics, the n-vector model or O(n) model is a simple system of interacting spins on a crystalline lattice. It was developed by H. Eugene Stanley as a generalization of the Ising model, XY model and Heisenberg model. In the n-vector model, n-component unit-length classical spins are placed on the vertices of a d-dimensional lattice. The Hamiltonian of the n-vector model is given by:
A Hopfield network is a spin glass system used to model neural networks, based on Ernst Ising's work with Wilhelm Lenz on the Ising model of magnetic materials. Hopfield networks were first described with respect to recurrent neural networks independently by Kaoru Nakano in 1971 and Shun'ichi Amari in 1972, and with respect to biological neural networks by William Little in 1974, and were popularised by John Hopfield in 1982. Hopfield networks serve as content-addressable ("associative") memory systems with binary threshold nodes, or with continuous variables. Hopfield networks also provide a model for understanding human memory.
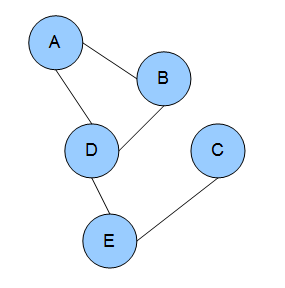
In the domain of physics and probability, a Markov random field (MRF), Markov network or undirected graphical model is a set of random variables having a Markov property described by an undirected graph. In other words, a random field is said to be a Markov random field if it satisfies Markov properties. The concept originates from the Sherrington–Kirkpatrick model.
In physics and mathematics, the Gibbs measure, named after Josiah Willard Gibbs, is a probability measure frequently seen in many problems of probability theory and statistical mechanics. It is a generalization of the canonical ensemble to infinite systems. The canonical ensemble gives the probability of the system X being in state x as
In statistical mechanics, the two-dimensional square lattice Ising model is a simple lattice model of interacting magnetic spins. The model is notable for having nontrivial interactions, yet having an analytical solution. The model was solved by Lars Onsager for the special case that the external magnetic field H = 0. An analytical solution for the general case for has yet to be found.
Wagner's gene network model is a computational model of artificial gene networks, which explicitly modeled the developmental and evolutionary process of genetic regulatory networks. A population with multiple organisms can be created and evolved from generation to generation. It was first developed by Andreas Wagner in 1996 and has been investigated by other groups to study the evolution of gene networks, gene expression, robustness, plasticity and epistasis.
In statistical mechanics, the Griffiths inequality, sometimes also called Griffiths–Kelly–Sherman inequality or GKS inequality, named after Robert B. Griffiths, is a correlation inequality for ferromagnetic spin systems. Informally, it says that in ferromagnetic spin systems, if the 'a-priori distribution' of the spin is invariant under spin flipping, the correlation of any monomial of the spins is non-negative; and the two point correlation of two monomial of the spins is non-negative.
Construction of an Irreducible Markov Chain in the Ising model is a mathematical method to prove results.

Epistasis is a phenomenon in genetics in which the effect of a gene mutation is dependent on the presence or absence of mutations in one or more other genes, respectively termed modifier genes. In other words, the effect of the mutation is dependent on the genetic background in which it appears. Epistatic mutations therefore have different effects on their own than when they occur together. Originally, the term epistasis specifically meant that the effect of a gene variant is masked by that of different gene.