
A pentomino is a polyomino of order 5; that is, a polygon in the plane made of 5 equal-sized squares connected edge to edge. The term is derived from the Greek word for '5' and "domino". When rotations and reflections are not considered to be distinct shapes, there are 12 different free pentominoes. When reflections are considered distinct, there are 18 one-sided pentominoes. When rotations are also considered distinct, there are 63 fixed pentominoes.

A polyomino is a plane geometric figure formed by joining one or more equal squares edge to edge. It is a polyform whose cells are squares. It may be regarded as a finite subset of the regular square tiling.
A polyiamond is a polyform whose base form is an equilateral triangle. The word polyiamond is a back-formation from diamond, because this word is often used to describe the shape of a pair of equilateral triangles placed base to base, and the initial 'di-' looks like a Greek prefix meaning 'two-'. The name was suggested by recreational mathematics writer Thomas H. O'Beirne in New Scientist 1961 number 1, page 164.

A tessellation or tiling is the covering of a surface, often a plane, using one or more geometric shapes, called tiles, with no overlaps and no gaps. In mathematics, tessellation can be generalized to higher dimensions and a variety of geometries.

A wallpaper group is a mathematical classification of a two-dimensional repetitive pattern, based on the symmetries in the pattern. Such patterns occur frequently in architecture and decorative art, especially in textiles, tiles, and wallpaper.

In recreational mathematics, a polydrafter is a polyform with a 30°–60°–90° right triangle as the base form. This triangle is also called a drafting triangle, hence the name. This triangle is also half of an equilateral triangle, and a polydrafter's cells must consist of halves of triangles in the triangular tiling of the plane; consequently, when two drafters share an edge that is the middle of their three edge lengths, they must be reflections rather than rotations of each other. Any contiguous subset of halves of triangles in this tiling is allowed, so unlike most polyforms, a polydrafter may have cells joined along unequal edges: a hypotenuse and a short leg.

In recreational mathematics, a polyabolo is a shape formed by gluing isosceles right triangles edge-to-edge, making a polyform with the isosceles right triangle as the base form. Polyaboloes were introduced by Martin Gardner in his June 1967 "Mathematical Games column" in Scientific American.

Euclidean plane tilings by convex regular polygons have been widely used since antiquity. The first systematic mathematical treatment was that of Kepler in his Harmonices Mundi.

A heptomino is a polyomino of order 7; that is, a polygon in the plane made of 7 equal-sized squares connected edge to edge. The name of this type of figure is formed with the prefix hept(a)-. When rotations and reflections are not considered to be distinct shapes, there are 108 different free heptominoes. When reflections are considered distinct, there are 196 one-sided heptominoes. When rotations are also considered distinct, there are 760 fixed heptominoes.

A nonomino is a polyomino of order 9; that is, a polygon in the plane made of 9 equal-sized squares connected edge to edge. The name of this type of figure is formed with the prefix non(a)-. When rotations and reflections are not considered to be distinct shapes, there are 1,285 different free nonominoes. When reflections are considered distinct, there are 2,500 one-sided nonominoes. When rotations are also considered distinct, there are 9,910 fixed nonominoes.

In geometry, the hexagonal tiling or hexagonal tessellation is a regular tiling of the Euclidean plane, in which exactly three hexagons meet at each vertex. It has Schläfli symbol of {6,3} or t{3,6} .
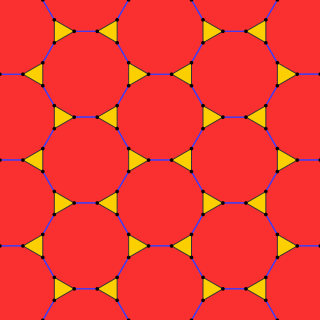
In geometry, the truncated hexagonal tiling is a semiregular tiling of the Euclidean plane. There are 2 dodecagons (12-sides) and one triangle on each vertex.

In geometry, the truncated trihexagonal tiling is one of eight semiregular tilings of the Euclidean plane. There are one square, one hexagon, and one dodecagon on each vertex. It has Schläfli symbol of tr{3,6}.

In geometry, the rhombitrihexagonal tiling is a semiregular tiling of the Euclidean plane. There are one triangle, two squares, and one hexagon on each vertex. It has Schläfli symbol of rr{3,6}.

The quarter cubic honeycomb, quarter cubic cellulation or bitruncated alternated cubic honeycomb is a space-filling tessellation in Euclidean 3-space. It is composed of tetrahedra and truncated tetrahedra in a ratio of 1:1. It is called "quarter-cubic" because its symmetry unit – the minimal block from which the pattern is developed by reflections – is four times that of the cubic honeycomb.

In geometry, a pentagonal tiling is a tiling of the plane where each individual piece is in the shape of a pentagon.

An octomino is a polyomino of order 8; that is, a polygon in the plane made of 8 equal-sized squares connected edge to edge. When rotations and reflections are not considered to be distinct shapes, there are 369 different free octominoes. When reflections are considered distinct, there are 704 one-sided octominoes. When rotations are also considered distinct, there are 2,725 fixed octominoes.

In geometry, an octadecagon or 18-gon is an eighteen-sided polygon.

In the geometry of tessellations, a rep-tile or reptile is a shape that can be dissected into smaller copies of the same shape. The term was coined as a pun on animal reptiles by recreational mathematician Solomon W. Golomb and popularized by Martin Gardner in his "Mathematical Games" column in the May 1963 issue of Scientific American. In 2012 a generalization of rep-tiles called self-tiling tile sets was introduced by Lee Sallows in Mathematics Magazine.
A decomino, or 10-omino, is a polyomino of order 10; that is, a polygon in the plane made of 10 equal-sized squares connected edge to edge. When rotations and reflections are not considered to be distinct shapes, there are 4,655 different free decominoes. When reflections are considered distinct, there are 9,189 one-sided decominoes. When rotations are also considered distinct, there are 36,446 fixed decominoes.
























