The mathematical formulations of quantum mechanics are those mathematical formalisms that permit a rigorous description of quantum mechanics. This mathematical formalism uses mainly a part of functional analysis, especially Hilbert spaces, which are a kind of linear space. Such are distinguished from mathematical formalisms for physics theories developed prior to the early 1900s by the use of abstract mathematical structures, such as infinite-dimensional Hilbert spaces, and operators on these spaces. In brief, values of physical observables such as energy and momentum were no longer considered as values of functions on phase space, but as eigenvalues; more precisely as spectral values of linear operators in Hilbert space.
In quantum mechanics, a density matrix is a matrix that describes the quantum state of a physical system. It allows for the calculation of the probabilities of the outcomes of any measurement performed upon this system, using the Born rule. It is a generalization of the more usual state vectors or wavefunctions: while those can only represent pure states, density matrices can also represent mixed states. Mixed states arise in quantum mechanics in two different situations:
- when the preparation of the system is not fully known, and thus one must deal with a statistical ensemble of possible preparations, and
- when one wants to describe a physical system which is entangled with another, without describing their combined state.
In physics, the CHSH inequality can be used in the proof of Bell's theorem, which states that certain consequences of entanglement in quantum mechanics cannot be reproduced by local hidden-variable theories. Experimental verification of the inequality being violated is seen as confirmation that nature cannot be described by such theories. CHSH stands for John Clauser, Michael Horne, Abner Shimony, and Richard Holt, who described it in a much-cited paper published in 1969. They derived the CHSH inequality, which, as with John Stewart Bell's original inequality, is a constraint on the statistical occurrence of "coincidences" in a Bell test which is necessarily true if there exist underlying local hidden variables, an assumption that is sometimes termed local realism. In practice, the inequality is routinely violated by modern experiments in quantum mechanics.

Quantum decoherence is the loss of quantum coherence, the process in which a system's behaviour changes from that which can be explained by quantum mechanics to that which can be explained by classical mechanics. In quantum mechanics, particles such as electrons are described by a wave function, a mathematical representation of the quantum state of a system; a probabilistic interpretation of the wave function is used to explain various quantum effects. As long as there exists a definite phase relation between different states, the system is said to be coherent. A definite phase relationship is necessary to perform quantum computing on quantum information encoded in quantum states. Coherence is preserved under the laws of quantum physics.
In quantum physics, a measurement is the testing or manipulation of a physical system to yield a numerical result. A fundamental feature of quantum theory is that the predictions it makes are probabilistic. The procedure for finding a probability involves combining a quantum state, which mathematically describes a quantum system, with a mathematical representation of the measurement to be performed on that system. The formula for this calculation is known as the Born rule. For example, a quantum particle like an electron can be described by a quantum state that associates to each point in space a complex number called a probability amplitude. Applying the Born rule to these amplitudes gives the probabilities that the electron will be found in one region or another when an experiment is performed to locate it. This is the best the theory can do; it cannot say for certain where the electron will be found. The same quantum state can also be used to make a prediction of how the electron will be moving, if an experiment is performed to measure its momentum instead of its position. The uncertainty principle implies that, whatever the quantum state, the range of predictions for the electron's position and the range of predictions for its momentum cannot both be narrow. Some quantum states imply a near-certain prediction of the result of a position measurement, but the result of a momentum measurement will be highly unpredictable, and vice versa. Furthermore, the fact that nature violates the statistical conditions known as Bell inequalities indicates that the unpredictability of quantum measurement results cannot be explained away as due to ignorance about "hidden variables" within quantum systems.
Quantum statistical mechanics is statistical mechanics applied to quantum mechanical systems. In quantum mechanics a statistical ensemble is described by a density operator S, which is a non-negative, self-adjoint, trace-class operator of trace 1 on the Hilbert space H describing the quantum system. This can be shown under various mathematical formalisms for quantum mechanics. One such formalism is provided by quantum logic.
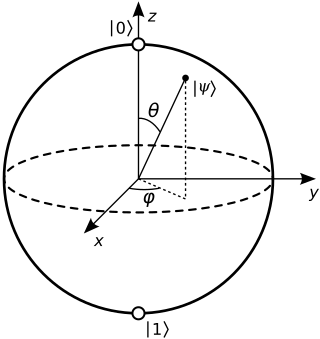
In quantum mechanics and computing, the Bloch sphere is a geometrical representation of the pure state space of a two-level quantum mechanical system (qubit), named after the physicist Felix Bloch.
In physics, the von Neumann entropy, named after John von Neumann, is an extension of the concept of Gibbs entropy from classical statistical mechanics to quantum statistical mechanics. For a quantum-mechanical system described by a density matrix ρ, the von Neumann entropy is
In functional analysis and quantum information science, a positive operator-valued measure (POVM) is a measure whose values are positive semi-definite operators on a Hilbert space. POVMs are a generalization of projection-valued measures (PVM) and, correspondingly, quantum measurements described by POVMs are a generalization of quantum measurement described by PVMs.
The Born rule is a postulate of quantum mechanics which gives the probability that a measurement of a quantum system will yield a given result. In its simplest form, it states that the probability density of finding a system in a given state, when measured, is proportional to the square of the amplitude of the system's wavefunction at that state. It was formulated by German physicist Max Born in 1926.
In quantum mechanics, notably in quantum information theory, fidelity is a measure of the "closeness" of two quantum states. It expresses the probability that one state will pass a test to identify as the other. The fidelity is not a metric on the space of density matrices, but it can be used to define the Bures metric on this space.
In quantum mechanics, the expectation value is the probabilistic expected value of the result (measurement) of an experiment. It can be thought of as an average of all the possible outcomes of a measurement as weighted by their likelihood, and as such it is not the most probable value of a measurement; indeed the expectation value may have zero probability of occurring. It is a fundamental concept in all areas of quantum physics.
A Werner state is a × -dimensional bipartite quantum state density matrix that is invariant under all unitary operators of the form . That is, it is a bipartite quantum state that satisfies
In quantum mechanics, and especially quantum information and the study of open quantum systems, the trace distanceT is a metric on the space of density matrices and gives a measure of the distinguishability between two states. It is the quantum generalization of the Kolmogorov distance for classical probability distributions.
This is a glossary for the terminology often encountered in undergraduate quantum mechanics courses.
The Maxwell–Bloch equations, also called the optical Bloch equations describe the dynamics of a two-state quantum system interacting with the electromagnetic mode of an optical resonator. They are analogous to the Bloch equations which describe the motion of the nuclear magnetic moment in an electromagnetic field. The equations can be derived either semiclassically or with the field fully quantized when certain approximations are made.
The Koopman–von Neumann mechanics is a description of classical mechanics in terms of Hilbert space, introduced by Bernard Koopman and John von Neumann in 1931 and 1932, respectively.
The entropy of entanglement is a measure of the degree of quantum entanglement between two subsystems constituting a two-part composite quantum system. Given a pure bipartite quantum state of the composite system, it is possible to obtain a reduced density matrix describing knowledge of the state of a subsystem. The entropy of entanglement is the Von Neumann entropy of the reduced density matrix for any of the subsystems. If it is non-zero, i.e. the subsystem is in a mixed state, it indicates the two subsystems are entangled.
In quantum information theory and quantum optics, the Schrödinger–HJW theorem is a result about the realization of a mixed state of a quantum system as an ensemble of pure quantum states and the relation between the corresponding purifications of the density operators. The theorem is named after physicists and mathematicians Erwin Schrödinger, Lane P. Hughston, Richard Jozsa and William Wootters. The result was also found independently by Nicolas Gisin, and by Nicolas Hadjisavvas building upon work by Ed Jaynes, while a significant part of it was likewise independently discovered by N. David Mermin. Thanks to its complicated history, it is also known by various other names such as the GHJW theorem, the HJW theorem, and the purification theorem.
The quantum Fisher information is a central quantity in quantum metrology and is the quantum analogue of the classical Fisher information. The quantum Fisher information of a state with respect to the observable is defined as
























































