Sea ice emissivity modelling

Last updated September 28, 2022

With increased interest in sea ice and its effects on the global climate, efficient methods are required to monitor both its extent and exchange processes. Satellite-mounted, microwave radiometers, such SSMI, AMSR and AMSU, are an ideal tool for the task because they can see through cloud cover, and they have frequent, global coverage. A passive microwave instrument detects objects through emitted radiation since different substance have different emission spectra. To detect sea ice more efficiently, there is a need to model these emission processes. The interaction of sea ice with electromagnetic radiation in the microwave range is still not well understood.^[1]^[2]^[3] In general is collected information limited because of the large-scale variability due to the emissivity of sea ice.^[4]

General

Satellite microwave data (and visible, infrared data depending on the conditions) collected from sensors assumes that ocean surface is a binary (ice covered or ice free) and observations are used to quantify the radiative flux. During the melt seasons in spring and summer, sea ice surface temperature goes above freezing. Thus, passive microwave measurements are able to detect rising brightness temperatures, as the emissivity increases to almost that of a blackbody, and as liquid starts to form around the ice crystals, but when melting continues, slush forms and then melt ponds and the brightness temperature goes down to that of ice free water. Because the emissivity of sea ice changes over time and often in short time spans, data and algorithms used to interpret findings are crucial.^[5]

Effective permittivity

As established in the previous section, the most important quantity in radiative transfer calculations of sea ice is the relative permittivity. Sea ice is a complex composite composed of pure ice and included pockets of air and highly saline brine. The electro-magnetic properties of such a mixture will be different from, and normally somewhere in between (though not always—see, for instance, metamaterial), those of its constituents. Since it is not just the relative composition that is important, but also the geometry, the calculation of effective permittivities introduces a high level of uncertainty.

Vant et al. ^[6] have performed actual measurements of sea ice relative permittivities at frequencies between 0.1 and 4.0 GHz which they have encapsulated in the following formula:

$\epsilon ^{*}=aV_{b}+b$

where $\epsilon ^{*}$ is the real or imaginary effective relative permittivity, V_b is the relative brine volume—see sea ice growth processes—and a and b are constants. This empirical model shows some agreement with dielectric mixture models based on Maxwell's equations in the low frequency limit, such as this formula from Sihvola and Kong

^[7]

$\epsilon _{eff}=\epsilon _{1}+{\frac {V_{b}\epsilon _{1}(\epsilon _{2}-\epsilon _{1})/(\epsilon _{1}+P(\epsilon _{2}-\epsilon 1)}{1-PV_{b}(\epsilon _{2}-\epsilon _{1})/\left[\epsilon _{1}+P(\epsilon _{2}-\epsilon _{1})\right]}}$

where $\epsilon _{1}$ is the relative permittivity of the background material (pure ice), $\epsilon _{2}$ is the relative permittivity of the inclusion material (brine) and P is a depolarization factor based on the geometry of the brine inclusions. Brine inclusions are frequently modelled as vertically oriented needles for which the depolarization factor is P=0.5 in the vertical direction and P=0. in the horizontal. The two formulas, while they correlate strongly, disagree in both relative and absolute magnitudes. ^[2]

Pure ice is an almost perfect dielectric with a real permittivity of roughly 3.15 in the microwave range which is fairly independent of frequency while the imaginary component is negligible, especially in comparison with the brine which is extremely lossy. ^[8] Meanwhile, the permittivity of the brine, which has both a large real part and a large imaginary part, is normally calculated with a complex formula based on Debye relaxation curves.^[8]

Electromagnetic properties of ice

When scattering is neglected, sea ice emissivity can be modelled through radiative transfer. The diagram to the right shows a ray passing through an ice sheet with several layers. These layers represent the air above the ice, the snow layer (if applicable), ice with different electro-magnetic properties and the water below the ice. Interfaces between the layers may be continuous (in the case of ice with varying salt content along the vertical axis, but formed in the same way and in the same time period), in which case the reflection coefficients, R_i will be zero, or discontinuous (in the case of the ice-snow interface), in which case reflection coefficients must be calculated—see below. Each layer is characterized by its physical properties: temperature, T_i, complex permittivity, $\epsilon _{i}$ and thickness, $\Delta z_{i}$ , and will have an upwards component of the radiation, $T_{i}\uparrow$ , and a downwards component, $T_{i}\downarrow$ , passing through it. Since we assume plane-parallel geometry, all reflected rays will be at the same angle and we need only account for radiation along a single line-of-sight.

Summing the contributions from each layer generates the following sparse system of linear equations:

T_{i}\uparrow -\tau _{i}(1-R_{i})T_{i+1}\uparrow -\tau _{i}R_{i}T_{i}\downarrow =(1-\tau _{i})T_{i}

T_{i}\downarrow -\tau _{i}(1-R_{i-1})T_{i-1}\downarrow -\tau _{i}R_{i-1}T_{i}\uparrow =(1-\tau _{i})T_{i}

^[2]

where R_i is the ith reflection coefficient, calculated via the Fresnel equations and $\tau _{i}$ is the ith transmission coefficient:

\tau _{i}=\exp \left(-{\frac {\alpha _{i}\,\Delta z_{i}}{\cos \theta _{i}}}\right)

where $\theta _{i}$ is the transmission angle in the ith layer, from Snell's law, $\Delta z_{i}$ is the layer thickness and $\alpha _{i}$ is the attenuation coefficient:

\alpha _{i}={\frac {4\pi \nu }{c}}\mathrm {Imag} \,n_{i}

where $\nu$ is the frequency and c is the speed of light—see Beer's law. The most important quantity in this calculation, and also the most difficult to establish with any certainty, is the complex refractive index, n_i.^[2] Since sea ice is non-magnetic, it can be calculated from relative permittivity alone:

$n_{i}={\sqrt {\epsilon _{i}}}$

Scattering

Emissivity calculations based strictly on radiative transfer tend to underestimate the brightness temperatures of sea ice, especially in the higher frequencies, because both included brine and air pockets within the ice will tend to scatter the radiation. ^[9] Indeed, as ice becomes more opaque with higher frequency, radiative transfer becomes less important while scattering processes begin to dominate. Scattering in sea ice is frequently modelled with a Born approximation ^[10] such as in strong fluctuation theory. ^[11]^[12]

Scattering coefficients calculated at each layer must also be vertically integrated. The Microwave Emission Model of Layered Snowpack (MEMLS) ^[13] uses a six-flux radiative transfer model to integrate both the scattering coefficients and the effective permittivities with scattering coefficients calculated either empirically or with a distorted Born approximation.

Scattering processes in sea ice are relatively poorly understood and scattering models poorly validated empirically. ^[1]^[3]

Other factors

There are many other factors not accounted for in the models described above. Mills and Heygster,^[2] for instance, show that sea ice ridging may have a significant effect on the signal. In such case, the ice can no longer be modelled using plane-parallel geometry. In addition to ridging, surface scattering from smaller-scale roughness must also be considered.

Since the microstructural properties of sea ice tend to be anisotropic, permittivity is ideally modelled as a tensor. This anisotropy will also affect the signal in the higher Stokes components, relevant for polarimetric radiometers such as WINDSAT. Both a sloping ice surface, as in the case of ridging—see polarization mixing, ^[1] as well as scattering, especially from non-symmetric scatterers, ^[14] will cause a transfer of intensity between the different Stokes components—see vector radiative transfer.

Related Research Articles

In physics, optical depth or optical thickness is the natural logarithm of the ratio of incident to transmitted radiant power through a material. Thus, the larger the optical depth, the smaller the amount of transmitted radiant power through the material. Spectral optical depth or spectral optical thickness is the natural logarithm of the ratio of incident to transmitted spectral radiant power through a material. Optical depth is dimensionless, and in particular is not a length, though it is a monotonically increasing function of optical path length, and approaches zero as the path length approaches zero. The use of the term "optical density" for optical depth is discouraged.

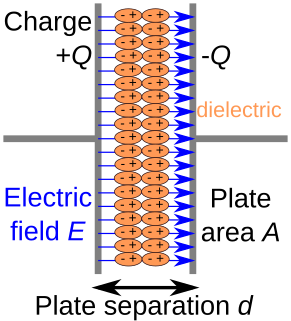
In electromagnetism, a dielectric is an electrical insulator that can be polarised by an applied electric field. When a dielectric material is placed in an electric field, electric charges do not flow through the material as they do in an electrical conductor, because they have no loosely bound, or free, electrons that may drift through the material, but instead they shift, only slightly, from their average equilibrium positions, causing dielectric polarisation. Because of dielectric polarisation, positive charges are displaced in the direction of the field and negative charges shift in the direction opposite to the field. This creates an internal electric field that reduces the overall field within the dielectric itself. If a dielectric is composed of weakly bonded molecules, those molecules not only become polarised, but also reorient so that their symmetry axes align to the field.

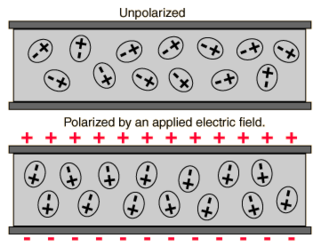
<span class="mw-page-title-main">Permittivity</span> Measure of the electric polarizability of a dielectric

In electromagnetism, the absolute permittivity, often simply called permittivity and denoted by the Greek letter ε (epsilon), is a measure of the electric polarizability of a dielectric. A material with high permittivity polarizes more in response to an applied electric field than a material with low permittivity, thereby storing more energy in the material. In electrostatics, the permittivity plays an important role in determining the capacitance of a capacitor.

Thermal radiation is electromagnetic radiation generated by the thermal motion of particles in matter. Thermal radiation is generated when heat from the movement of charges in the material is converted to electromagnetic radiation. All matter with a temperature greater than absolute zero emits thermal radiation. At room temperature, most of the emission is in the infrared (IR) spectrum. Particle motion results in charge-acceleration or dipole oscillation which produces electromagnetic radiation.

The laser diode rate equations model the electrical and optical performance of a laser diode. This system of ordinary differential equations relates the number or density of photons and charge carriers (electrons) in the device to the injection current and to device and material parameters such as carrier lifetime, photon lifetime, and the optical gain.

The Drude model of electrical conduction was proposed in 1900 by Paul Drude to explain the transport properties of electrons in materials. Basically, Ohm's law was well established and stated that the current J and voltage V driving the current are related to the resistance R of the material. The inverse of the resistance is known as the conductance. When we consider a metal of unit length and unit cross sectional area, the conductance is known as the conductivity, which is the inverse of resistivity. The Drude model attempts to explain the resistivity of a conductor in terms of the scattering of electrons by the relatively immobile ions in the metal that act like obstructions to the flow of electrons.

Thomson scattering is the elastic scattering of electromagnetic radiation by a free charged particle, as described by classical electromagnetism. It is the low-energy limit of Compton scattering: the particle's kinetic energy and photon frequency do not change as a result of the scattering. This limit is valid as long as the photon energy is much smaller than the mass energy of the particle: $, or equivalently, if the wavelength of the light is much greater than the Compton wavelength of the particle.$

The Havriliak–Negami relaxation is an empirical modification of the Debye relaxation model in electromagnetism. Unlike the Debye model, the Havriliak–Negami relaxation accounts for the asymmetry and broadness of the dielectric dispersion curve. The model was first used to describe the dielectric relaxation of some polymers, by adding two exponential parameters to the Debye equation:

Non-line-of-sight (NLOS) radio propagation occurs outside of the typical line-of-sight (LOS) between the transmitter and receiver, such as in ground reflections. Near-line-of-sight conditions refer to partial obstruction by a physical object present in the innermost Fresnel zone.

Radiative transfer is the physical phenomenon of energy transfer in the form of electromagnetic radiation. The propagation of radiation through a medium is affected by absorption, emission, and scattering processes. The equation of radiative transfer describes these interactions mathematically. Equations of radiative transfer have application in a wide variety of subjects including optics, astrophysics, atmospheric science, and remote sensing. Analytic solutions to the radiative transfer equation (RTE) exist for simple cases but for more realistic media, with complex multiple scattering effects, numerical methods are required. The present article is largely focused on the condition of radiative equilibrium.

Kenneth Stewart Cole was an American biophysicist described by his peers as "a pioneer in the application of physical science to biology". Cole was awarded the National Medal of Science in 1967.

The surface of the Sun radiates light and heat at approximately 5,500 °C. The Earth is much cooler and so radiates heat back away from itself at much longer wavelengths, mostly in the infrared range. The idealized greenhouse model is based on the fact that certain gases in the Earth's atmosphere, including carbon dioxide and water vapour, are transparent to the high-frequency, high-energy solar radiation, but are much more opaque to the lower frequency infrared radiation leaving the surface of the earth. Thus heat is easily let in, but is partially trapped by these gases as it tries to leave. Rather than get hotter and hotter, Kirchhoff's law of thermal radiation says that the gases of the atmosphere also have to re-emit the infrared energy that they absorb, and they do so, also at long infrared wavelengths, both upwards into space as well as downwards back towards the Earth's surface. In the long-term, thermal equilibrium is reached when all the heat energy arriving on the planet is leaving again at the same rate. In this idealized model, the greenhouse gases cause the surface of the planet to be warmer than it would be without them, in order for the required amount of heat energy finally to be radiated out into space from the top of the atmosphere.

The Stoner criterion is a condition to be fulfilled for the ferromagnetic order to arise in a simplified model of a solid. It is named after Edmund Clifton Stoner.

A microwave cavity or radio frequency (RF) cavity is a special type of resonator, consisting of a closed metal structure that confines electromagnetic fields in the microwave region of the spectrum. The structure is either hollow or filled with dielectric material. The microwaves bounce back and forth between the walls of the cavity. At the cavity's resonant frequencies they reinforce to form standing waves in the cavity. Therefore, the cavity functions similarly to an organ pipe or sound box in a musical instrument, oscillating preferentially at a series of frequencies, its resonant frequencies. Thus it can act as a bandpass filter, allowing microwaves of a particular frequency to pass while blocking microwaves at nearby frequencies.

In dielectric spectroscopy, large frequency dependent contributions to the dielectric response, especially at low frequencies, may come from build-ups of charge. This Maxwell–Wagner–Sillars polarization, occurs either at inner dielectric boundary layers on a mesoscopic scale, or at the external electrode-sample interface on a macroscopic scale. In both cases this leads to a separation of charges. The charges are often separated over a considerable distance, and the contribution to dielectric loss can therefore be orders of magnitude larger than the dielectric response due to molecular fluctuations.

Ultrasound-modulated optical tomography (UOT) is a form of tomography involving ultrasound. It is used in imaging of biological soft tissues and has potential applications for early cancer detection. Like optical techniques, this method provides high contrast, and the use of ultrasound also provides high resolution.

The Grey atmosphere is a useful set of approximations made for radiative transfer applications in studies of stellar atmospheres based on the simplified notion that the absorption coefficient $of matter within a star's atmosphere is constant—that is, unchanging—for all frequencies of the star's incident radiation.$

<span class="mw-page-title-main">Semiconductor laser theory</span> Theory of laser diodes

Semiconductor lasers or laser diodes play an important part in our everyday lives by providing cheap and compact-size lasers. They consist of complex multi-layer structures requiring nanometer scale accuracy and an elaborate design. Their theoretical description is important not only from a fundamental point of view, but also in order to generate new and improved designs. It is common to all systems that the laser is an inverted carrier density system. The carrier inversion results in an electromagnetic polarization which drives an electric field $. In most cases, the electric field is confined in a resonator, the properties of which are also important factors for laser performance.$

Satellite surface salinity refers to measurements of surface salinity made by remote sensing satellites. The radiative properties of the ocean surface are exploited in order to estimate the salinity of the water's surface layer.

The Cole-Davidson equation is a model used to describe dielectric relaxation in glass-forming liquids. The equation for the complex permittivity is

References

1 2 3 4 G. Heygster; S. Hendricks; L. Kaleschke; N. Maass; et al. (2009). L-Band Radiometry for Sea-Ice Applications (Technical report). Institute of Environmental Physics, University of Bremen. ESA/ESTEC Contract N. 21130/08/NL/EL.
1 2 3 4 5 Peter Mills & Georg Heygster (2011). "Sea ice emissivity modelling at L-band and application to Pol-Ice campaign field data" (PDF). IEEE Transactions on Geoscience and Remote Sensing. 49 (2): 612–627. Bibcode:2011ITGRS..49..612M. doi:10.1109/TGRS.2010.2060729. S2CID 20981849.
1 2 Peter Mills & Georg Heygster (2011). Sea ice brightness temperature as a function of ice thickness: Computed curves for AMSR-E and SMOS (frequencies from 1.4 to 89 GHz) (PDF) (Technical report). Institute of Environmental Physics, University of Bremen. DFG project HE-1746-15.
↑ Rothrock, D. A.; Thomas, Donald R.; Thorndike, Alan S. (March 15, 1988). "Principal component analysis of satellite passive microwave data over sea ice". Journal of Geophysical Research: Oceans. 93 (C3): 2321–2332. Bibcode:1988JGR....93.2321R. doi:10.1029/JC093iC03p02321.
↑ Josefino C. Comiso (2009). "Enhanced Sea Ice Concentrations from Passive Microwave Data" (PDF). J. Remote Sens. Society Japan. 29: 199–215. ISSN 1883-1184.
↑ M. R. Vant; R. O. Ramseier & V. Makios (1978). "The complex-dielectric constant of sea ice at frequencies in the range 0.1-4.0 GHz". Journal of Applied Physics. 49 (3): 1246–1280. Bibcode:1978JAP....49.1264V. doi:10.1063/1.325018.
↑ A. H. Sihvola nd J. Kong (1988). "Effective Permittivity of Dielectric Mixtures". IEEE Transactions on Geoscience and Remote Sensing. 26 (4): 420. Bibcode:1988ITGRS..26..420S. doi:10.1109/36.3045.
1 2 W. B. Tucker; D. K. Prerovich; A. J. Gow; W. F. Weeks; M. R. Drinkwater (eds.). Microwave Remote Sensing of Sea Ice. American Geophysical Union.
↑ F. T. Ulaby; R. K. Moore; A. K. Fung, eds. (1986). Microwave Remote Sensing, Active and Passive. London, England: Addison Wesley.
↑ Christian Maetzler (1998). "Improved born approximation for scattering in a granular medium". Journal of Applied Physics. 83 (11): 6111–6117. Bibcode:1998JAP....83.6111M. doi:10.1063/1.367496.
↑ A. Stogryn (1986). "A study of microwave brightness temperatures of snow from the point of view of strong fluctuation theory". IEEE Transactions on Geoscience and Remote Sensing. 24 (2): 220–231. Bibcode:1986ITGRS..24..220S. doi:10.1109/TGRS.1986.289641. S2CID 32207638.
↑ Klaus-Peter Johnsen (1998). Radiometrische Messungen im Arktischen Ozean-Vergleich von Theorie und Experiement (Thesis). University of Bremen.
↑ A. Wiesmann & C. Maetzler (1999). "Microwave emission model for layered snowpacks". Remote Sensing of Environment. 70 (3): 307–316. Bibcode:1999RSEnv..70..307W. doi:10.1016/s0034-4257(99)00046-2.
↑ Emde, Claudia (2005). A Polarized Discrete Ordinate Scattering Model for Radiative Transfer Simulations in Spherical Atmospheres (PDF) (Thesis).

This page is based on this Wikipedia article
Text is available under the CC BY-SA 4.0 license; additional terms may apply.
Images, videos and audio are available under their respective licenses.

[smos_final-1] 1 2 3 4 G. Heygster; S. Hendricks; L. Kaleschke; N. Maass; et al. (2009). L-Band Radiometry for Sea-Ice Applications (Technical report). Institute of Environmental Physics, University of Bremen. ESA/ESTEC Contract N. 21130/08/NL/EL.

[Mills_Heygster2010-2] 1 2 3 4 5 Peter Mills & Georg Heygster (2011). "Sea ice emissivity modelling at L-band and application to Pol-Ice campaign field data" (PDF). IEEE Transactions on Geoscience and Remote Sensing. 49 (2): 612–627. Bibcode:2011ITGRS..49..612M. doi:10.1109/TGRS.2010.2060729. S2CID 20981849.

[Mills_Heygster2011-3] 1 2 Peter Mills & Georg Heygster (2011). Sea ice brightness temperature as a function of ice thickness: Computed curves for AMSR-E and SMOS (frequencies from 1.4 to 89 GHz) (PDF) (Technical report). Institute of Environmental Physics, University of Bremen. DFG project HE-1746-15.

[4] Rothrock, D. A.; Thomas, Donald R.; Thorndike, Alan S. (March 15, 1988). "Principal component analysis of satellite passive microwave data over sea ice". Journal of Geophysical Research: Oceans. 93 (C3): 2321–2332. Bibcode:1988JGR....93.2321R. doi:10.1029/JC093iC03p02321.

[5] Josefino C. Comiso (2009). "Enhanced Sea Ice Concentrations from Passive Microwave Data" (PDF). J. Remote Sens. Society Japan. 29: 199–215. ISSN 1883-1184.

[Vant_etal1978-6] M. R. Vant; R. O. Ramseier & V. Makios (1978). "The complex-dielectric constant of sea ice at frequencies in the range 0.1-4.0 GHz". Journal of Applied Physics. 49 (3): 1246–1280. Bibcode:1978JAP....49.1264V. doi:10.1063/1.325018.

[Sihvola_Kong1988-7] A. H. Sihvola nd J. Kong (1988). "Effective Permittivity of Dielectric Mixtures". IEEE Transactions on Geoscience and Remote Sensing. 26 (4): 420. Bibcode:1988ITGRS..26..420S. doi:10.1109/36.3045.

[Tucker_etal1992-8] 1 2 W. B. Tucker; D. K. Prerovich; A. J. Gow; W. F. Weeks; M. R. Drinkwater (eds.). Microwave Remote Sensing of Sea Ice. American Geophysical Union.

[Ulaby_etal1986-9] F. T. Ulaby; R. K. Moore; A. K. Fung, eds. (1986). Microwave Remote Sensing, Active and Passive. London, England: Addison Wesley.

[Maetzler1998-10] Christian Maetzler (1998). "Improved born approximation for scattering in a granular medium". Journal of Applied Physics. 83 (11): 6111–6117. Bibcode:1998JAP....83.6111M. doi:10.1063/1.367496.

[Stogryn1986-11] A. Stogryn (1986). "A study of microwave brightness temperatures of snow from the point of view of strong fluctuation theory". IEEE Transactions on Geoscience and Remote Sensing. 24 (2): 220–231. Bibcode:1986ITGRS..24..220S. doi:10.1109/TGRS.1986.289641. S2CID 32207638.

[Johnsen1998-12] Klaus-Peter Johnsen (1998). Radiometrische Messungen im Arktischen Ozean-Vergleich von Theorie und Experiement (Thesis). University of Bremen.

[Wiesmann_Maetzler1999-13] A. Wiesmann & C. Maetzler (1999). "Microwave emission model for layered snowpacks". Remote Sensing of Environment. 70 (3): 307–316. Bibcode:1999RSEnv..70..307W. doi:10.1016/s0034-4257(99)00046-2.

[Emde2005-14] Emde, Claudia (2005). A Polarized Discrete Ordinate Scattering Model for Radiative Transfer Simulations in Spherical Atmospheres (PDF) (Thesis).

[1]

[2]

[3]

[4]

[5]

[6]

[7]

[8]

[9]

[10]

[11]

[12]

[13]

[14]