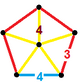
In geometry, a 4-polytope is a four-dimensional polytope. It is a connected and closed figure, composed of lower-dimensional polytopal elements: vertices, edges, faces (polygons), and cells (polyhedra). Each face is shared by exactly two cells. The 4-polytopes were discovered by the Swiss mathematician Ludwig Schläfli before 1853.

In geometry, a uniform 4-polytope is a 4-dimensional polytope which is vertex-transitive and whose cells are uniform polyhedra, and faces are regular polygons.

In four-dimensional geometry, a runcinated 5-cell is a convex uniform 4-polytope, being a runcination of the regular 5-cell.

In geometry, a dodecahedral prism is a convex uniform 4-polytope. This 4-polytope has 14 polyhedral cells: 2 dodecahedra connected by 12 pentagonal prisms. It has 54 faces: 30 squares and 24 pentagons. It has 80 edges and 40 vertices.

In geometry, the rectified 24-cell or rectified icositetrachoron is a uniform 4-dimensional polytope, which is bounded by 48 cells: 24 cubes, and 24 cuboctahedra. It can be obtained by rectification of the 24-cell, reducing its octahedral cells to cubes and cuboctahedra.

In four-dimensional geometry, a cantellated 24-cell is a convex uniform 4-polytope, being a cantellation of the regular 24-cell.

In geometry, a cuboctahedral prism is a convex uniform 4-polytope. This 4-polytope has 16 polyhedral cells: 2 cuboctahedra connected by 8 triangular prisms and 6 cubes.

In geometry, a truncated tetrahedral prism is a convex uniform polychoron. This polychoron has 10 polyhedral cells: 2 truncated tetrahedra connected by 4 triangular prisms and 4 hexagonal prisms. It has 24 faces: 8 triangular, 18 square, and 8 hexagons. It has 48 edges and 24 vertices.

In geometry, an icosahedral prism is a convex uniform 4-polytope. This 4-polytope has 22 polyhedral cells: 2 icosahedra connected by 20 triangular prisms. It has 70 faces: 30 squares and 40 triangles. It has 72 edges and 24 vertices.
In 4-dimensional geometry, a truncated octahedral prism or omnitruncated tetrahedral prism is a convex uniform 4-polytope. This 4-polytope has 16 cells It has 64 faces, and 96 edges and 48 vertices.

In geometry, an icosidodecahedral prism is a convex uniform polychoron.

In geometry, a truncated dodecahedral prism is a convex uniform polychoron.

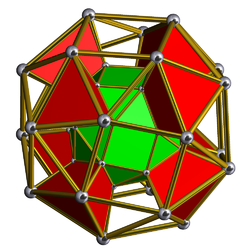
In geometry, a rhombicosidodecahedral prism or small rhombicosidodecahedral prism is a convex uniform polychoron.
In geometry, a rhombicuboctahedral prism is a convex uniform polychoron.
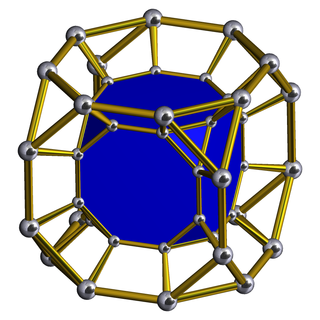
In geometry, a truncated cubic prism is a convex uniform polychoron.

In geometry, a truncated cuboctahedral prism or great rhombicuboctahedral prism is a convex uniform polychoron.

In geometry, a truncated icosahedral prism is a convex uniform polychoron.

In geometry, a truncated icosidodecahedral prism or great rhombicosidodecahedral prism is a convex uniform 4-polytope.
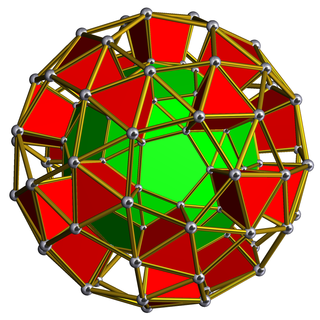
In geometry, a snub dodecahedral prism or snub icosidodecahedral prism is a convex uniform polychoron.