In geometry, an Archimedean solid is one of the 13 solids first enumerated by Archimedes. They are the semi-regular convex polyhedra composed of regular polygons meeting in identical vertices, excluding the 5 Platonic solids and excluding the prisms and antiprisms. They differ from the Johnson solids, whose regular polygonal faces do not meet in identical vertices.

In geometry, the snub cube, or snub cuboctahedron, is an Archimedean solid with 38 faces: 6 squares and 32 equilateral triangles. It has 60 edges and 24 vertices.

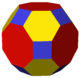
In geometry, the truncated cuboctahedron is an Archimedean solid, named by Kepler as a truncation of a cuboctahedron. It has 12 square faces, 8 regular hexagonal faces, 6 regular octagonal faces, 48 vertices and 72 edges. Since each of its faces has point symmetry, the truncated cuboctahedron is a zonohedron. The truncated cuboctahedron can tessellate with the octagonal prism.
In geometry, this may refer to:
- Truncated cuboctahedron - an Archimedean solid, with Schläfli symbol tr{4,3}, and Coxeter diagram .
- Nonconvex great rhombicuboctahedron - a uniform star polyhedron, with Schläfli symbol r{4,3/2}, and Coxeter diagram .
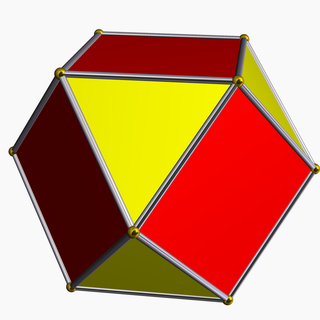
In Euclidean geometry, rectification or complete-truncation is the process of truncating a polytope by marking the midpoints of all its edges, and cutting off its vertices at those points. The resulting polytope will be bounded by vertex figure facets and the rectified facets of the original polytope.

In four-dimensional geometry, a runcinated tesseract is a convex uniform 4-polytope, being a runcination of the regular tesseract.

In four-dimensional geometry, a cantellated tesseract is a convex uniform 4-polytope, being a cantellation of the regular tesseract.

The cubic honeycomb or cubic cellulation is the only regular space-filling tessellation in Euclidean 3-space, made up of cubic cells. It has 4 cubes around every edge, and 8 cubes around each vertex. Its vertex figure is a regular octahedron. It is a self-dual tessellation with Schläfli symbol {4,3,4}. John Horton Conway calls this honeycomb a cubille.

In geometry, the nonconvex great rhombicuboctahedron is a nonconvex uniform polyhedron, indexed as U17. It is represented by Schläfli symbol t0,2{4,3/2} and Coxeter-Dynkin diagram of . Its vertex figure is a crossed quadrilateral.

In geometry, Conway polyhedron notation, invented by John Horton Conway and promoted by George W. Hart, is used to describe polyhedra based on a seed polyhedron modified by various prefix operations.

In four-dimensional geometry, a cantellated 24-cell is a convex uniform 4-polytope, being a cantellation of the regular 24-cell.

In geometry, a snub is an operation applied to a polyhedron. The term originates from Kepler's names of two Archimedean solids, for the snub cube and snub dodecahedron. In general, snubs have chiral symmetry with two forms, with clockwise or counterclockwise orientations. By Kepler's names, a snub can be seen as an expansion of a regular polyhedron, with the faces moved apart, and twists on their centers, adding new polygons centered on the original vertices, and pairs of triangles fitting between the original edges.

In the geometry of hyperbolic 3-space, the square tiling honeycomb, is one of 11 paracompact regular honeycombs. It is called paracompact because it has infinite cells, whose vertices exist on horospheres and converge to a single ideal point at infinity. Given by Schläfli symbol {4,4,3}, has three square tilings, {4,4} around each edge, and 6 square tilings around each vertex in a cubic {4,3} vertex figure.

In the geometry of hyperbolic 3-space, the order-4 octahedral honeycomb is a regular paracompact honeycomb. It is called paracompact because it has infinite vertex figures, with all vertices as ideal points at infinity. Given by Schläfli symbol {3,4,4}, it has four octahedra, {3,4} around each edge, and infinite octahedra around each vertex in a square tiling {4,4} vertex arrangement.
In the geometry of hyperbolic 3-space, the cube-octahedron honeycomb is a compact uniform honeycomb, constructed from cube, octahedron, and cuboctahedron cells, in a rhombicuboctahedron vertex figure. It has a single-ring Coxeter diagram, , and is named by its two regular cells.

The truncated rhombicuboctahedron is a polyhedron, constructed as a truncated rhombicuboctahedron. It has 50 faces, 18 octagons, 8 hexagons, and 24 squares. It can fill space with the truncated cube, truncated tetrahedron and triangular prism as a truncated runcic cubic honeycomb.

John Horton Conway FRS is an English mathematician active in the theory of finite groups, knot theory, number theory, combinatorial game theory and coding theory. He has also contributed to many branches of recreational mathematics, notably the invention of the cellular automaton called the Game of Life. Conway spent the first half of his long career at the University of Cambridge, in England, and the second half at Princeton University in New Jersey, where he now holds the title Professor Emeritus.

Chaim Goodman-Strauss is an American mathematician who works in convex geometry, especially aperiodic tiling. He is on the faculty of the University of Arkansas and is a co-author with John H. Conway of The Symmetries of Things, a comprehensive book surveying the mathematical theory of patterns.

The International Standard Book Number (ISBN) is a numeric commercial book identifier which is intended to be unique. Publishers purchase ISBNs from an affiliate of the International ISBN Agency.