Solids with icosahedral symmetry

Main article: Icosahedral symmetry

Solids with full icosahedral symmetry

Platonic solids - regular polyhedra (all faces of the same type)

{5,3}	{3,5}

Archimedean solids - polyhedra with more than one polygon face type.

3.10.10

4.6.10

5.6.6

3.4.5.4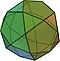

3.5.3.5

Catalan solids - duals of the Archimedean solids.

V3.10.10

V4.6.10

V5.6.6

V3.4.5.4

V3.5.3.5

Platonic solids

Name	Picture	Faces	Edges	Vertices	Edges per face	Faces meeting at each vertex
dodecahedron	(Animation)	12	30	20	5	3
icosahedron	(Animation)	20	30	12	3	5

Achiral Archimedean solids

Name	picture	Faces		Edges	Vertices	Vertex configuration
icosidodecahedron (quasi-regular: vertex- and edge-uniform)	(Video)	32	20 triangles 12 pentagons	60	30	3,5,3,5
truncated dodecahedron	(Video)	32	20 triangles 12 decagons	90	60	3,10,10
truncated icosahedron or commonly football (soccer ball)	(Video)	32	12 pentagons 20 hexagons	90	60	5,6,6
rhombicosidodecahedron or small rhombicosidodecahedron	(Video)	62	20 triangles 30 squares 12 pentagons	120	60	3,4,5,4
truncated icosidodecahedron or great rhombicosidodecahedron	(Video)	62	30 squares 20 hexagons 12 decagons	180	120	4,6,10

Achiral Catalan solids

Name	picture	Dual Archimedean solid	Faces	Edges	Vertices	Face Polygon
rhombic triacontahedron (quasi-regular dual: face- and edge-uniform)	(Video)	icosidodecahedron	30	60	32	rhombus
triakis icosahedron	(Video)	truncated dodecahedron	60	90	32	isosceles triangle
pentakis dodecahedron	(Video)	truncated icosahedron	60	90	32	isosceles triangle
deltoidal hexecontahedron	(Video)	rhombicosidodecahedron	60	120	62	kite
disdyakis triacontahedron or hexakis icosahedron	(Video)	truncated icosidodecahedron	120	180	62	scalene triangle

Kepler-Poinsot solids

Achiral nonconvex uniform polyhedra

Chiral Archimedean and Catalan solids

Archimedean solids:

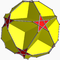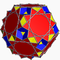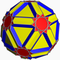Name	picture	Faces		Edges	Vertices	Vertex configuration
snub dodecahedron or snub icosidodecahedron (2 chiral forms)	(Video) (Video)	92	80 triangles 12 pentagons	150	60	3,3,3,3,5

Catalan solids:

Name	picture	Dual Archimedean solid	Faces	Edges	Vertices	Face Polygon
pentagonal hexecontahedron	(Video)(Video)	snub dodecahedron	60	50	92	irregular pentagon

Chiral nonconvex uniform polyhedra

See also

• The Fifty Nine Icosahedra