In mathematics, a continuous function is a function such that a continuous variation of the argument induces a continuous variation of the value of the function. This means that there are no abrupt changes in value, known as discontinuities. More precisely, a function is continuous if arbitrarily small changes in its value can be assured by restricting to sufficiently small changes of its argument. A discontinuous function is a function that is not continuous. Up until the 19th century, mathematicians largely relied on intuitive notions of continuity, and considered only continuous functions. The epsilon–delta definition of a limit was introduced to formalize the definition of continuity.

In mathematics, the derivative shows the sensitivity of change of a function's output with respect to the input. Derivatives are a fundamental tool of calculus. For example, the derivative of the position of a moving object with respect to time is the object's velocity: this measures how quickly the position of the object changes when time advances.

The history of calculus is fraught with philosophical debates about the meaning and logical validity of fluxions or infinitesimal numbers. The standard way to resolve these debates is to define the operations of calculus using epsilon–delta procedures rather than infinitesimals. Nonstandard analysis instead reformulates the calculus using a logically rigorous notion of infinitesimal numbers.
In mathematics, the branch of real analysis studies the behavior of real numbers, sequences and series of real numbers, and real functions. Some particular properties of real-valued sequences and functions that real analysis studies include convergence, limits, continuity, smoothness, differentiability and integrability.

In mathematics, the system of hyperreal numbers is a way of treating infinite and infinitesimal quantities. The hyperreals, or nonstandard reals, *R, are an extension of the real numbers R that contains numbers greater than anything of the form

In mathematics, an infinitesimal number is a quantity that is closer to zero than any standard real number, but that is not zero. The word infinitesimal comes from a 17th-century Modern Latin coinage infinitesimus, which originally referred to the "infinity-th" item in a sequence.
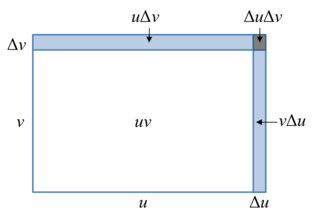
In calculus, the product rule is a formula used to find the derivatives of products of two or more functions. For two functions, it may be stated in Lagrange's notation as

In mathematics, the limit of a function is a fundamental concept in calculus and analysis concerning the behavior of that function near a particular input.

In calculus, Leibniz's notation, named in honor of the 17th-century German philosopher and mathematician Gottfried Wilhelm Leibniz, uses the symbols dx and dy to represent infinitely small increments of x and y, respectively, just as Δx and Δy represent finite increments of x and y, respectively.
In nonstandard analysis, a branch of mathematics, overspill is a widely used proof technique. It is based on the fact that the set of standard natural numbers N is not an internal subset of the internal set *N of hypernatural numbers.
In model theory, a transfer principle states that all statements of some language that are true for some structure are true for another structure. One of the first examples was the Lefschetz principle, which states that any sentence in the first-order language of fields that is true for the complex numbers is also true for any algebraically closed field of characteristic 0.
In mathematics, nonstandard calculus is the modern application of infinitesimals, in the sense of nonstandard analysis, to infinitesimal calculus. It provides a rigorous justification for some arguments in calculus that were previously considered merely heuristic.
In mathematics, differential refers to several related notions derived from the early days of calculus, put on a rigorous footing, such as infinitesimal differences and the derivatives of functions.
In mathematical logic, in particular in model theory and nonstandard analysis, an internal set is a set that is a member of a model.
In nonstandard analysis, a field of mathematics, the increment theorem states the following: Suppose a function y = f(x) is differentiable at x and that Δx is infinitesimal. Then
In nonstandard analysis, a hyperintegern is a hyperreal number that is equal to its own integer part. A hyperinteger may be either finite or infinite. A finite hyperinteger is an ordinary integer. An example of an infinite hyperinteger is given by the class of the sequence (1, 2, 3, ...) in the ultrapower construction of the hyperreals.
Elementary Calculus: An Infinitesimal approach is a textbook by H. Jerome Keisler. The subtitle alludes to the infinitesimal numbers of the hyperreal number system of Abraham Robinson and is sometimes given as An approach using infinitesimals. The book is available freely online and is currently published by Dover.

In calculus, the differential represents the principal part of the change in a function with respect to changes in the independent variable. The differential is defined by
In mathematics, a limit is the value that a function approaches as the input approaches some value. Limits are essential to calculus and mathematical analysis, and are used to define continuity, derivatives, and integrals.
In nonstandard analysis, a discipline within classical mathematics, microcontinuity (or S-continuity) of an internal function f at a point a is defined as follows: