Related Research Articles
In combinatorial mathematics, the Lubell–Yamamoto–Meshalkin inequality, more commonly known as the LYM inequality, is an inequality on the sizes of sets in a Sperner family, proved by Bollobás (1965), Lubell (1966), Meshalkin (1963), and Yamamoto (1954). It is named for the initials of three of its discoverers. To include the initials of all four discoverers, it is sometimes referred to as the YBLM inequality.
In mathematics, in the areas of order theory and combinatorics, Dilworth's theorem characterizes the width of any finite partially ordered set in terms of a partition of the order into a minimum number of chains. It is named for the mathematician Robert P. Dilworth (1950).
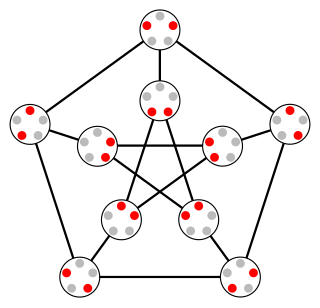
In graph theory, the Kneser graphK(n, k) is the graph whose vertices correspond to the k-element subsets of a set of n elements, and where two vertices are adjacent if and only if the two corresponding sets are disjoint. Kneser graphs are named after Martin Kneser, who first investigated them in 1955.
In mathematics, Bondy's theorem is a bound on the number of elements needed to distinguish the sets in a family of sets from each other. It belongs to the field of combinatorics, and is named after John Adrian Bondy, who published it in 1972.
In mathematics, the Milliken–Taylor theorem in combinatorics is a generalization of both Ramsey's theorem and Hindman's theorem. It is named after Keith Milliken and Alan D. Taylor.

Daniel J. Kleitman is an American mathematician and professor of applied mathematics at MIT. His research interests include combinatorics, graph theory, genomics, and operations research.
Robert Palmer Dilworth was an American mathematician. His primary research area was lattice theory; his biography at the MacTutor History of Mathematics archive states "it would not be an exaggeration to say that he was one of the main factors in the subject moving from being merely a tool of other disciplines to an important subject in its own right". He is best known for Dilworth's theorem relating chains and antichains in partial orders; he was also the first to study antimatroids.
G. W. Peck is a pseudonymous attribution used as the author or co-author of a number of published mathematics academic papers. Peck is sometimes humorously identified with George Wilbur Peck, a former governor of the US state of Wisconsin.
The Journal of Combinatorial Theory, Series A and Series B, are mathematical journals specializing in combinatorics and related areas. They are published by Elsevier. Series A is concerned primarily with structures, designs, and applications of combinatorics. Series B is concerned primarily with graph and matroid theory. The two series are two of the leading journals in the field and are widely known as JCTA and JCTB.

In number theory, and especially the study of diophantine approximation, the lonely runner conjecture is a conjecture originally due to J. M. Wills in 1967. Applications of the conjecture are widespread in mathematics; they include view obstruction problems and calculating the chromatic number of distance graphs and circulant graphs. The conjecture was given its picturesque name by L. Goddyn in 1998.
Polyhedral combinatorics is a branch of mathematics, within combinatorics and discrete geometry, that studies the problems of counting and describing the faces of convex polyhedra and higher-dimensional convex polytopes.
In mathematics, Dixon's identity is any of several different but closely related identities proved by A. C. Dixon, some involving finite sums of products of three binomial coefficients, and some evaluating a hypergeometric sum. These identities famously follow from the MacMahon Master theorem, and can now be routinely proved by computer algorithms.
The mathematical field of combinatorics was studied to varying degrees in numerous ancient societies. Its study in Europe dates to the work of Leonardo Fibonacci in the 13th century AD, which introduced Arabian and Indian ideas to the continent. It has continued to be studied in the modern era.
In algebraic combinatorics, a Bender–Knuth involution is an involution on the set of semistandard tableaux, introduced by Bender & Knuth in their study of plane partitions.
Thomas Zaslavsky is an American mathematician specializing in combinatorics.
In geometry, the moment curve is an algebraic curve in d-dimensional Euclidean space given by the set of points with Cartesian coordinates of the form
Haim Hanani was a Polish-born Israeli mathematician, known for his contributions to combinatorial design theory, in particular for the theory of pairwise balanced designs and for the proof of an existence theorem for Steiner quadruple systems. He is also known for the Hanani–Tutte theorem on odd crossings in non-planar graphs.
Rodica Eugenia Simion was a Romanian-American mathematician. She was the Columbian School Professor of Mathematics at George Washington University. Her research concerned combinatorics: she was a pioneer in the study of permutation patterns, and an expert on noncrossing partitions.
Richard Ehrenborg is a Swedish mathematician working in algebraic combinatorics. He is known for developing the quasisymmetric function of a poset. He currently holds the Ralph E. and Norma L. Edwards Research Professorship at the University of Kentucky and is the first recipient of the Royster Research Professor at University of Kentucky.
In mathematics, the polynomial method is an algebraic approach to combinatorics problems that involves capturing some combinatorial structure using polynomials and proceeding to argue about their algebraic properties. Recently, the polynomial method has led to the development of remarkably simple solutions to several long-standing open problems. The polynomial method encompasses a wide range of specific techniques for using polynomials and ideas from areas such as algebraic geometry to solve combinatorics problems. While a few techniques that follow the framework of the polynomial method, such as Alon's Combinatorial Nullstellensatz, have been known since the 1990s, it was not until around 2010 that a broader framework for the polynomial method has been developed.
References
- 1 2 Faculty profile and home page Archived 2012-04-13 at the Wayback Machine , Haverford College, retrieved 2012-02-20.
- ↑ Curtis Greene at the Mathematics Genealogy Project
- ↑ Greene, Curtis; Kleitman, Daniel J. (1976), "The structure of Sperner k-families", Journal of Combinatorial Theory , Series A, 20 (1): 41–68, doi: 10.1016/0097-3165(76)90077-7 , MR 0398844 . Greene, Curtis; Kleitman, Daniel J. (1976), "Strong versions of Sperner's theorem", Journal of Combinatorial Theory , Series A, 20 (1): 80–88, doi: 10.1016/0097-3165(76)90079-0 , MR 0389608 . Greene, Curtis (1976), "Some partitions associated with a partially ordered set", Journal of Combinatorial Theory , Series A, 20 (1): 69–79, doi: 10.1016/0097-3165(76)90078-9 , MR 0398912
- ↑ Greene, Curtis (1974), "An extension of Schensted's theorem", Advances in Mathematics , 14 (2): 254–265, doi: 10.1016/0001-8708(74)90031-0 , MR 0354395 . Edelman, Paul; Greene, Curtis (1987), "Balanced tableaux", Advances in Mathematics , 63 (1): 42–99, doi: 10.1016/0001-8708(87)90063-6 , MR 0871081 . Greene, Curtis; Nijenhuis, Albert; Wilf, Herbert S. (1979), "A probabilistic proof of a formula for the number of Young tableaux of a given shape", Advances in Mathematics , 31 (1): 104–109, doi: 10.1016/0001-8708(79)90023-9 , MR 0521470 .
- ↑ Greene, Curtis; Zaslavsky, Thomas (1983), "On the interpretation of Whitney numbers through arrangements of hyperplanes, zonotopes, non-Radon partitions, and orientations of graphs", Transactions of the American Mathematical Society , 280 (1): 97–126, doi: 10.2307/1999604 , JSTOR 1999604, MR 0712251 .
- ↑ Greene, Curtis; Kleitman, Daniel J. (1978), "Proof techniques in the theory of finite sets", Studies in combinatorics, MAA Stud. Math., 17, Washington, D.C.: Math. Assoc. America, pp. 22–79, MR 0513002 .
- ↑ List of Fellows of the American Mathematical Society, retrieved 2013-01-19.