In geometry, the four-vertex theorem states that the curvature along a simple, closed, smooth plane curve has at least four local extrema (specifically, at least two local maxima and at least two local minima). The name of the theorem derives from the convention of calling an extreme point of the curvature function a vertex. This theorem has many generalizations, including a version for space curves where a vertex is defined as a point of vanishing torsion.
The curvature at any point of a smooth curve in the plane can be defined as the reciprocal of the radius of an osculating circle at that point, or as the norm of the second derivative of a parametric representation of the curve, parameterized consistently with the length along the curve. [1] For the vertices of a curve to be well-defined, the curvature itself should vary continuously, [2] as happens for curves of smoothness . [3] A vertex is then a local maximum or local minimum of curvature. If the curvature is constant over an arc of the curve, all points of that arc are considered to be vertices. The four-vertex theorem states that a smooth closed curve always has at least four vertices.
An ellipse has exactly four vertices: two local maxima of curvature where it is crossed by the major axis of the ellipse, and two local minima of curvature where it is crossed by the minor axis. In a circle, every point is both a local maximum and a local minimum of curvature, so there are infinitely many vertices. [3] If a smooth closed curve crosses a circle times, then it has at least vertices, so a curve with exactly four vertices such as an ellipse can cross any circle at most four times. [4]
Every curve of constant width has at least six vertices. Although many curves of constant width, such as the Reuleaux triangle, are non-smooth or have circular arcs on their boundaries, there exist smooth curves of constant width that have exactly six vertices. [5] [6]
The four-vertex theorem was first proved for convex curves (i.e. curves with strictly positive curvature) in 1909 by Syamadas Mukhopadhyaya. [7] His proof utilizes the fact that a point on the curve is an extremum of the curvature function if and only if the osculating circle at that point has fourth-order contact with the curve; in general the osculating circle has only third-order contact with the curve. The four-vertex theorem was proved for more general curves by Adolf Kneser in 1912 using a projective argument. [8]
For many years the proof of the four-vertex theorem remained difficult, but a simple and conceptual proof was given by Osserman (1985), based on the idea of the minimum enclosing circle. [9] This is a circle that contains the given curve and has the smallest possible radius. If the curve includes an arc of the circle, it has infinitely many vertices. Otherwise, the curve and circle must be tangent at at least two points, because a circle that touched the curve at fewer points could be reduced in size while still enclosing it. At each tangency, the curvature of the curve is greater than that of the circle, for otherwise the curve would continue from the tangency outside the circle rather than inside. However, between each pair of tangencies, the curvature must decrease to less than that of the circle, for instance at a point obtained by translating the circle until it no longer contains any part of the curve between the two points of tangency and considering the last point of contact between the translated circle and the curve. Therefore, there is a local minimum of curvature between each pair of tangencies, giving two of the four vertices. There must be a local maximum of curvature between each pair of local minima (not necessarily at the points of tangency), giving the other two vertices. [9] [3]
The converse to the four-vertex theorem states that any continuous, real-valued function of the circle that has at least two local maxima and two local minima is the curvature function of a simple, closed plane curve. The converse was proved for strictly positive functions in 1971 by Herman Gluck as a special case of a general theorem on pre-assigning the curvature of n-spheres. [10] The full converse to the four-vertex theorem was proved by Björn Dahlberg shortly before his death in January 1998, and published posthumously. [11] Dahlberg's proof uses a winding number argument which is in some ways reminiscent of the standard topological proof of the Fundamental Theorem of Algebra. [12]
One corollary of the theorem is that a homogeneous, planar disk rolling on a horizontal surface under gravity has at least 4 balance points. A discrete version of this is that there cannot be a monostatic polygon. However, in three dimensions there do exist monostatic polyhedra, and there also exists a convex, homogeneous object with exactly 2 balance points (one stable, and the other unstable), the Gömböc.
There are several discrete versions of the four-vertex theorem, both for convex and non-convex polygons. [13] Here are some of them:
Some of these variations are stronger than the other, and all of them imply the (usual) four-vertex theorem by a limit argument.[ citation needed ]
The stereographic projection from the once-punctured sphere to the plane preserves critical points of geodesic curvature. Thus simple closed spherical curves have four vertices. Furthermore, on the sphere vertices of a curve correspond to points where its torsion vanishes. So for space curves a vertex is defined as a point of vanishing torsion. Every simple closed space curve which lies on the boundary of a convex body has four vertices. [14] This theorem can be generalized to all curves which bound a locally convex disk. [15]
A triangle is a polygon with three corners and three sides, one of the basic shapes in geometry. The corners, also called vertices, are zero-dimensional points while the sides connecting them, also called edges, are one-dimensional line segments. A triangle has three internal angles, each one bounded by a pair of adjacent edges; the sum of angles of a triangle always equals a straight angle. The triangle is a plane figure and its interior is a planar region. Sometimes an arbitrary edge is chosen to be the base, in which case the opposite vertex is called the apex; the shortest segment between the base and apex is the height. The area of a triangle equals one-half the product of height and base length.
In mathematics, curvature is any of several strongly related concepts in geometry that intuitively measure the amount by which a curve deviates from being a straight line or by which a surface deviates from being a plane. If a curve or surface is contained in a larger space, curvature can be defined extrinsically relative to the ambient space. Curvature of Riemannian manifolds of dimension at least two can be defined intrinsically without reference to a larger space.
In mathematics, two functions have a contact of order k if, at a point P, they have the same value and their first k derivatives are equal. This is an equivalence relation, whose equivalence classes are generally called jets. The point of osculation is also called the double cusp. Contact is a geometric notion; it can be defined algebraically as a valuation.
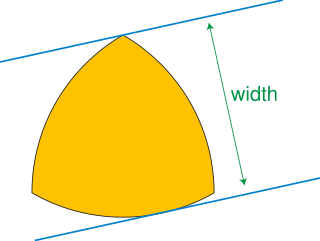
In geometry, a curve of constant width is a simple closed curve in the plane whose width is the same in all directions. The shape bounded by a curve of constant width is a body of constant width or an orbiform, the name given to these shapes by Leonhard Euler. Standard examples are the circle and the Reuleaux triangle. These curves can also be constructed using circular arcs centered at crossings of an arrangement of lines, as the involutes of certain curves, or by intersecting circles centered on a partial curve.
In geometry, a convex polygon is a polygon that is the boundary of a convex set. This means that the line segment between two points of the polygon is contained in the union of the interior and the boundary of the polygon. In particular, it is a simple polygon. Equivalently, a polygon is convex if every line that does not contain any edge intersects the polygon in at most two points.
In geometry, a simple polygon is a polygon that does not intersect itself and has no holes. That is, it is a piecewise-linear Jordan curve consisting of finitely many line segments. These polygons include as special cases the convex polygons, star-shaped polygons, and monotone polygons.
An osculating circle is a circle that best approximates the curvature of a curve at a specific point. It is tangent to the curve at that point and has the same curvature as the curve at that point. The osculating circle provides a way to understand the local behavior of a curve and is commonly used in differential geometry and calculus.
Geometric graph theory in the broader sense is a large and amorphous subfield of graph theory, concerned with graphs defined by geometric means. In a stricter sense, geometric graph theory studies combinatorial and geometric properties of geometric graphs, meaning graphs drawn in the Euclidean plane with possibly intersecting straight-line edges, and topological graphs, where the edges are allowed to be arbitrary continuous curves connecting the vertices; thus, it can be described as "the theory of geometric and topological graphs". Geometric graphs are also known as spatial networks.
In the geometry of plane curves, a vertex is a point of where the first derivative of curvature is zero. This is typically a local maximum or minimum of curvature, and some authors define a vertex to be more specifically a local extremum of curvature. However, other special cases may occur, for instance when the second derivative is also zero, or when the curvature is constant. For space curves, on the other hand, a vertex is a point where the torsion vanishes.
In Euclidean plane geometry, a pseudotriangle (pseudo-triangle) is the simply connected subset of the plane that lies between any three mutually tangent convex sets. A pseudotriangulation (pseudo-triangulations) is a partition of a region of the plane into pseudotriangles, and a pointed pseudotriangulation is a pseudotriangulation in which at each vertex the incident edges span an angle of less than π.
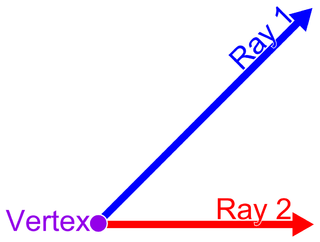
In geometry, a vertex is a point where two or more curves, lines, or edges meet or intersect. As a consequence of this definition, the point where two lines meet to form an angle and the corners of polygons and polyhedra are vertices.
In mathematical study of the differential geometry of curves, the total curvature of an immersed plane curve is the integral of curvature along a curve taken with respect to arc length:
In polyhedral combinatorics, a branch of mathematics, Steinitz's theorem is a characterization of the undirected graphs formed by the edges and vertices of three-dimensional convex polyhedra: they are exactly the 3-vertex-connected planar graphs. That is, every convex polyhedron forms a 3-connected planar graph, and every 3-connected planar graph can be represented as the graph of a convex polyhedron. For this reason, the 3-connected planar graphs are also known as polyhedral graphs.
The Alexandrov uniqueness theorem is a rigidity theorem in mathematics, describing three-dimensional convex polyhedra in terms of the distances between points on their surfaces. It implies that convex polyhedra with distinct shapes from each other also have distinct metric spaces of surface distances, and it characterizes the metric spaces that come from the surface distances on polyhedra. It is named after Soviet mathematician Aleksandr Danilovich Aleksandrov, who published it in the 1940s.
In geometry, a convex curve is a plane curve that has a supporting line through each of its points. There are many other equivalent definitions of these curves, going back to Archimedes. Examples of convex curves include the convex polygons, the boundaries of convex sets, and the graphs of convex functions. Important subclasses of convex curves include the closed convex curves, the smooth curves that are convex, and the strictly convex curves, which have the additional property that each supporting line passes through a unique point of the curve.
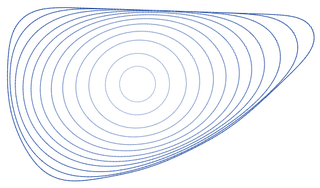
In mathematics, the curve-shortening flow is a process that modifies a smooth curve in the Euclidean plane by moving its points perpendicularly to the curve at a speed proportional to the curvature. The curve-shortening flow is an example of a geometric flow, and is the one-dimensional case of the mean curvature flow. Other names for the same process include the Euclidean shortening flow, geometric heat flow, and arc length evolution.
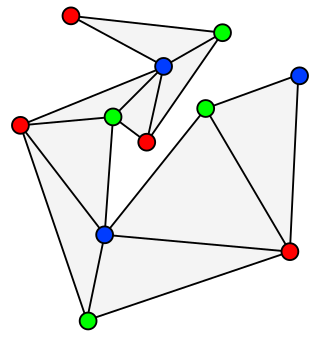
In geometry, the two ears theorem states that every simple polygon with more than three vertices has at least two ears, vertices that can be removed from the polygon without introducing any crossings. The two ears theorem is equivalent to the existence of polygon triangulations. It is frequently attributed to Gary H. Meisters, but was proved earlier by Max Dehn.
In geometry, the tennis ball theorem states that any smooth curve on the surface of a sphere that divides the sphere into two equal-area subsets without touching or crossing itself must have at least four inflection points, points at which the curve does not consistently bend to only one side of its tangent line. The tennis ball theorem was first published under this name by Vladimir Arnold in 1994, and is often attributed to Arnold, but a closely related result appears earlier in a 1968 paper by Beniamino Segre, and the tennis ball theorem itself is a special case of a theorem in a 1977 paper by Joel L. Weiner. The name of the theorem comes from the standard shape of a tennis ball, whose seam forms a curve that meets the conditions of the theorem; the same kind of curve is also used for the seams on baseballs.
In differential geometry, the Tait–Kneser theorem states that, if a smooth plane curve has monotonic curvature, then the osculating circles of the curve are disjoint and nested within each other. The logarithmic spiral or the pictured Archimedean spiral provide examples of curves whose curvature is monotonic for the entire curve. This monotonicity cannot happen for a simple closed curve but for such curves the theorem can be applied to the arcs of the curves between its vertices.
