
Euclidean geometry is a mathematical system attributed to ancient Greek mathematician Euclid, which he described in his textbook on geometry, Elements. Euclid's approach consists in assuming a small set of intuitively appealing axioms (postulates) and deducing many other propositions (theorems) from these. Although many of Euclid's results had been stated earlier, Euclid was the first to organize these propositions into a logical system in which each result is proved from axioms and previously proved theorems.

In mathematics, a Möbius strip, Möbius band, or Möbius loop is a surface that can be formed by attaching the ends of a strip of paper together with a half-twist. As a mathematical object, it was discovered by Johann Benedict Listing and August Ferdinand Möbius in 1858, but it had already appeared in Roman mosaics from the third century CE. The Möbius strip is a non-orientable surface, meaning that within it one cannot consistently distinguish clockwise from counterclockwise turns. Every non-orientable surface contains a Möbius strip.
In mathematics, the Erlangen program is a method of characterizing geometries based on group theory and projective geometry. It was published by Felix Klein in 1872 as Vergleichende Betrachtungen über neuere geometrische Forschungen. It is named after the University Erlangen-Nürnberg, where Klein worked.
In mathematics, projective geometry is the study of geometric properties that are invariant with respect to projective transformations. This means that, compared to elementary Euclidean geometry, projective geometry has a different setting, projective space, and a selective set of basic geometric concepts. The basic intuitions are that projective space has more points than Euclidean space, for a given dimension, and that geometric transformations are permitted that transform the extra points to Euclidean points, and vice versa.

In mathematics, the conformal group of an inner product space is the group of transformations from the space to itself that preserve angles. More formally, it is the group of transformations that preserve the conformal geometry of the space.
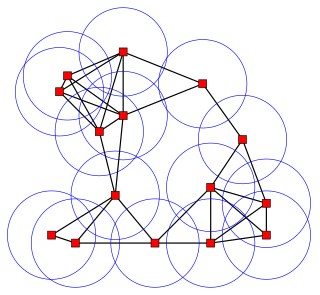
Discrete geometry and combinatorial geometry are branches of geometry that study combinatorial properties and constructive methods of discrete geometric objects. Most questions in discrete geometry involve finite or discrete sets of basic geometric objects, such as points, lines, planes, circles, spheres, polygons, and so forth. The subject focuses on the combinatorial properties of these objects, such as how they intersect one another, or how they may be arranged to cover a larger object.
In geometry, hyperbolic motions are isometric automorphisms of a hyperbolic space. Under composition of mappings, the hyperbolic motions form a continuous group. This group is said to characterize the hyperbolic space. Such an approach to geometry was cultivated by Felix Klein in his Erlangen program. The idea of reducing geometry to its characteristic group was developed particularly by Mario Pieri in his reduction of the primitive notions of geometry to merely point and motion.
Geometry is a branch of mathematics concerned with questions of shape, size, relative position of figures, and the properties of space. Geometry is one of the oldest mathematical sciences.

Indra's Pearls: The Vision of Felix Klein is a geometry book written by David Mumford, Caroline Series and David Wright, and published by Cambridge University Press in 2002 and 2015.
In mathematics, a Benz plane is a type of 2-dimensional geometrical structure, named after the German mathematician Walter Benz. The term was applied to a group of objects that arise from a common axiomatization of certain structures and split into three families, which were introduced separately: Möbius planes, Laguerre planes, and Minkowski planes.

In mathematics, a Cayley–Klein metric is a metric on the complement of a fixed quadric in a projective space which is defined using a cross-ratio. The construction originated with Arthur Cayley's essay "On the theory of distance" where he calls the quadric the absolute. The construction was developed in further detail by Felix Klein in papers in 1871 and 1873, and subsequent books and papers. The Cayley–Klein metrics are a unifying idea in geometry since the method is used to provide metrics in hyperbolic geometry, elliptic geometry, and Euclidean geometry. The field of non-Euclidean geometry rests largely on the footing provided by Cayley–Klein metrics.

Hans Wilhelm Eduard Schwerdtfeger was a German-Canadian-Australian mathematician who worked in Galois theory, matrix theory, theory of groups and their geometries, and complex analysis.
In mathematics, a geometric transformation is any bijection of a set to itself with some salient geometrical underpinning. More specifically, it is a function whose domain and range are sets of points — most often both or both — such that the function is bijective so that its inverse exists. The study of geometry may be approached by the study of these transformations.
In geometry, an object has symmetry if there is an operation or transformation that maps the figure/object onto itself. Thus, a symmetry can be thought of as an immunity to change. For instance, a circle rotated about its center will have the same shape and size as the original circle, as all points before and after the transform would be indistinguishable. A circle is thus said to be symmetric under rotation or to have rotational symmetry. If the isometry is the reflection of a plane figure about a line, then the figure is said to have reflectional symmetry or line symmetry; it is also possible for a figure/object to have more than one line of symmetry.
A Treatise on the Circle and the Sphere is a mathematics book on circles, spheres, and inversive geometry. It was written by Julian Coolidge, and published by the Clarendon Press in 1916. The Chelsea Publishing Company published a corrected reprint in 1971, and after the American Mathematical Society acquired Chelsea Publishing it was reprinted again in 1997.
Regular Figures is a book on polyhedra and symmetric patterns, by Hungarian geometer László Fejes Tóth. It was published in 1964 by Pergamon in London and Macmillan in New York.

Geometric and Topological Inference is a monograph in computational geometry, computational topology, geometry processing, and topological data analysis, on the problem of inferring properties of an unknown space from a finite point cloud of noisy samples from the space. It was written by Jean-Daniel Boissonnat, Frédéric Chazal, and Mariette Yvinec, and published in 2018 by the Cambridge University Press in their Cambridge Texts in Applied Mathematics book series. The Basic Library List Committee of the Mathematical Association of America has suggested its inclusion in undergraduate mathematics libraries.
The Symmetries of Things is a book on mathematical symmetry and the symmetries of geometric objects, aimed at audiences of multiple levels. It was written over the course of many years by John Horton Conway, Heidi Burgiel, and Chaim Goodman-Strauss, and published in 2008 by A K Peters. Its critical reception was mixed, with some reviewers praising it for its accessible and thorough approach to its material and for its many inspiring illustrations, and others complaining about its inconsistent level of difficulty, overuse of neologisms, failure to adequately cite prior work, and technical errors.

Curvature of Space and Time, with an Introduction to Geometric Analysis is an undergraduate-level textbook for mathematics and physics students on differential geometry, focusing on applications to general relativity. It was written by Iva Stavrov, based on a course she taught at the 2013 Park City Mathematics Institute and subsequently at Lewis & Clark College, and was published in 2020 by the American Mathematical Society, as part of their Student Mathematical Library book series.

The Geometry of the Octonions is a mathematics book on the octonions, a system of numbers generalizing the complex numbers and quaternions, presenting its material at a level suitable for undergraduate mathematics students. It was written by Tevian Dray and Corinne Manogue, and published in 2015 by World Scientific. The Basic Library List Committee of the Mathematical Association of America has suggested its inclusion in undergraduate mathematics libraries.












