In mathematics, complex geometry is the study of geometric structures and constructions arising out of, or described by, the complex numbers. In particular, complex geometry is concerned with the study of spaces such as complex manifolds and complex algebraic varieties, functions of several complex variables, and holomorphic constructions such as holomorphic vector bundles and coherent sheaves. Application of transcendental methods to algebraic geometry falls in this category, together with more geometric aspects of complex analysis.

In algebraic and differential geometry, a Calabi–Yau manifold, also known as a Calabi–Yau space, is a particular type of manifold which has properties, such as Ricci flatness, yielding applications in theoretical physics. Particularly in superstring theory, the extra dimensions of spacetime are sometimes conjectured to take the form of a 6-dimensional Calabi–Yau manifold, which led to the idea of mirror symmetry. Their name was coined by Candelas et al. (1985), after Eugenio Calabi who first conjectured that such surfaces might exist, and Shing-Tung Yau who proved the Calabi conjecture.
In mathematics, specifically in homology theory and algebraic topology, cohomology is a general term for a sequence of abelian groups, usually one associated with a topological space, often defined from a cochain complex. Cohomology can be viewed as a method of assigning richer algebraic invariants to a space than homology. Some versions of cohomology arise by dualizing the construction of homology. In other words, cochains are functions on the group of chains in homology theory.
In mathematics, a Lie groupoid is a groupoid where the set of objects and the set of morphisms are both manifolds, all the category operations are smooth, and the source and target operations
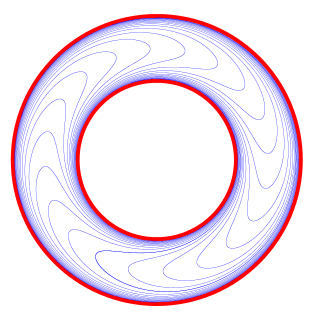
In mathematics, a foliation is an equivalence relation on an n-manifold, the equivalence classes being connected, injectively immersed submanifolds, all of the same dimension p, modeled on the decomposition of the real coordinate space Rn into the cosets x + Rp of the standardly embedded subspace Rp. The equivalence classes are called the leaves of the foliation. If the manifold and/or the submanifolds are required to have a piecewise-linear, differentiable, or analytic structure then one defines piecewise-linear, differentiable, or analytic foliations, respectively. In the most important case of differentiable foliation of class Cr it is usually understood that r ≥ 1. The number p is called the dimension of the foliation and q = n − p is called its codimension.
In mathematics and especially differential geometry, a Kähler manifold is a manifold with three mutually compatible structures: a complex structure, a Riemannian structure, and a symplectic structure. The concept was first studied by Jan Arnoldus Schouten and David van Dantzig in 1930, and then introduced by Erich Kähler in 1933. The terminology has been fixed by André Weil. Kähler geometry refers to the study of Kähler manifolds, their geometry and topology, as well as the study of structures and constructions that can be performed on Kähler manifolds, such as the existence of special connections like Hermitian Yang–Mills connections, or special metrics such as Kähler–Einstein metrics.

In mathematics, Frobenius' theorem gives necessary and sufficient conditions for finding a maximal set of independent solutions of an overdetermined system of first-order homogeneous linear partial differential equations. In modern geometric terms, given a family of vector fields, the theorem gives necessary and sufficient integrability conditions for the existence of a foliation by maximal integral manifolds whose tangent bundles are spanned by the given vector fields. The theorem generalizes the existence theorem for ordinary differential equations, which guarantees that a single vector field always gives rise to integral curves; Frobenius gives compatibility conditions under which the integral curves of r vector fields mesh into coordinate grids on r-dimensional integral manifolds. The theorem is foundational in differential topology and calculus on manifolds.

In differential geometry, the holonomy of a connection on a smooth manifold is a general geometrical consequence of the curvature of the connection measuring the extent to which parallel transport around closed loops fails to preserve the geometrical data being transported. For flat connections, the associated holonomy is a type of monodromy and is an inherently global notion. For curved connections, holonomy has nontrivial local and global features.
In differential geometry, a hyperkähler manifold is a Riemannian manifold endowed with three integrable almost complex structures that are Kähler with respect to the Riemannian metric and satisfy the quaternionic relations . In particular, it is a hypercomplex manifold. All hyperkähler manifolds are Ricci-flat and are thus Calabi–Yau manifolds.
In differential geometry, a field in mathematics, a Poisson manifold is a smooth manifold endowed with a Poisson structure. The notion of Poisson manifold generalises that of symplectic manifold, which in turn generalises the phase space from Hamiltonian mechanics.

In mathematics, a 3-manifold is a topological space that locally looks like a three-dimensional Euclidean space. A 3-manifold can be thought of as a possible shape of the universe. Just as a sphere looks like a plane to a small and close enough observer, all 3-manifolds look like our universe does to a small enough observer. This is made more precise in the definition below.
In mathematics, tautness is a rigidity property of foliations. A taut foliation is a codimension 1 foliation of a closed manifold with the property that every leaf meets a transverse circle. By transverse circle, is meant a closed loop that is always transverse to the tangent field of the foliation.
In differential geometry, a quaternion-Kähler manifold (or quaternionic Kähler manifold) is a Riemannian 4n-manifold whose Riemannian holonomy group is a subgroup of Sp(n)·Sp(1) for some . Here Sp(n) is the sub-group of consisting of those orthogonal transformations that arise by left-multiplication by some quaternionic matrix, while the group of unit-length quaternions instead acts on quaternionic -space by right scalar multiplication. The Lie group generated by combining these actions is then abstractly isomorphic to .
In mathematics, Floer homology is a tool for studying symplectic geometry and low-dimensional topology. Floer homology is a novel invariant that arises as an infinite-dimensional analogue of finite-dimensional Morse homology. Andreas Floer introduced the first version of Floer homology, now called symplectic Floer homology, in his proof of the Arnold conjecture in symplectic geometry. Floer also developed a closely related theory for Lagrangian submanifolds of a symplectic manifold. A third construction, also due to Floer, associates homology groups to closed three-dimensional manifolds using the Yang–Mills functional. These constructions and their descendants play a fundamental role in current investigations into the topology of symplectic and contact manifolds as well as (smooth) three- and four-dimensional manifolds.
In mathematics, a CR manifold, or Cauchy–Riemann manifold, is a differentiable manifold together with a geometric structure modeled on that of a real hypersurface in a complex vector space, or more generally modeled on an edge of a wedge.

Georges Henri Reeb was a French mathematician. He worked in differential topology, differential geometry, differential equations, topological dynamical systems theory and non-standard analysis.
In mathematics, Novikov's compact leaf theorem, named after Sergei Novikov, states that
In mathematics, Reeb sphere theorem, named after Georges Reeb, states that
In mathematics, the Thurston boundary of Teichmüller space of a surface is obtained as the boundary of its closure in the projective space of functionals on simple closed curves on the surface. The Thurston boundary can be interpreted as the space of projective measured foliations on the surface.
In gravitation theory, a world manifold endowed with some Lorentzian pseudo-Riemannian metric and an associated space-time structure is a space-time. Gravitation theory is formulated as classical field theory on natural bundles over a world manifold.
























