In geometry, a pseudosphere is a surface with constant negative Gaussian curvature.
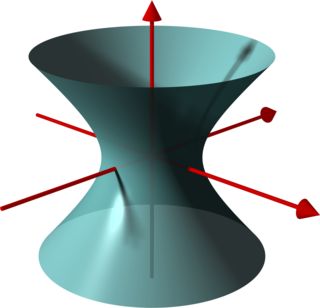
In geometry, a hyperboloid of revolution, sometimes called a circular hyperboloid, is the surface generated by rotating a hyperbola around one of its principal axes. A hyperboloid is the surface obtained from a hyperboloid of revolution by deforming it by means of directional scalings, or more generally, of an affine transformation.

In geometry, a paraboloid is a quadric surface that has exactly one axis of symmetry and no center of symmetry. The term "paraboloid" is derived from parabola, which refers to a conic section that has a similar property of symmetry.

Mathematics and architecture are related, since, as with other arts, architects use mathematics for several reasons. Apart from the mathematics needed when engineering buildings, architects use geometry: to define the spatial form of a building; from the Pythagoreans of the sixth century BC onwards, to create forms considered harmonious, and thus to lay out buildings and their surroundings according to mathematical, aesthetic and sometimes religious principles; to decorate buildings with mathematical objects such as tessellations; and to meet environmental goals, such as to minimise wind speeds around the bases of tall buildings.

In mathematics, a saddle point or minimax point is a point on the surface of the graph of a function where the slopes (derivatives) in orthogonal directions are all zero, but which is not a local extremum of the function. An example of a saddle point is when there is a critical point with a relative minimum along one axial direction and at a relative maximum along the crossing axis. However, a saddle point need not be in this form. For example, the function has a critical point at that is a saddle point since it is neither a relative maximum nor relative minimum, but it does not have a relative maximum or relative minimum in the -direction.
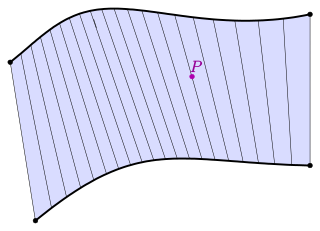
In geometry, a surface S is ruled if through every point of S there is a straight line that lies on S. Examples include the plane, the lateral surface of a cylinder or cone, a conical surface with elliptical directrix, the right conoid, the helicoid, and the tangent developable of a smooth curve in space.
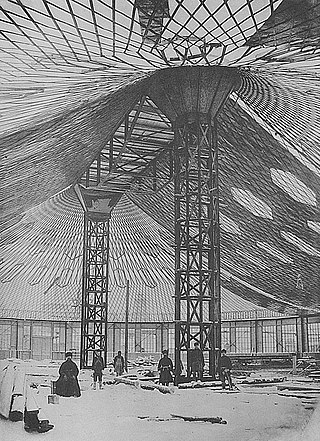
In structural engineering, a tensile structure is a construction of elements carrying only tension and no compression or bending. The term tensile should not be confused with tensegrity, which is a structural form with both tension and compression elements. Tensile structures are the most common type of thin-shell structures.
Hyperbolic is an adjective describing something that resembles or pertains to a hyperbola, to hyperbole, or to hyperbolic geometry.

The Cathedral of Saint Mary of the Assumption, also known locally as Saint Mary's Cathedral, is the principal church of the Roman Catholic Archdiocese of San Francisco in San Francisco, California. It is the mother church of the Catholic faithful in the California counties of Marin, San Francisco and San Mateo and is the metropolitan cathedral for the Ecclesiastical province of San Francisco.

Eduardo Fernando Catalano was an Argentine architect.

Hyperboloid structures are architectural structures designed using a hyperboloid in one sheet. Often these are tall structures, such as towers, where the hyperboloid geometry's structural strength is used to support an object high above the ground. Hyperboloid geometry is often used for decorative effect as well as structural economy. The first hyperboloid structures were built by Russian engineer Vladimir Shukhov (1853–1939), including the Shukhov Tower in Polibino, Dankovsky District, Lipetsk Oblast, Russia.

The Cathedral of Brasília is the Roman Catholic cathedral serving Brasília, Brazil, and serves as the seat of the Archdiocese of Brasília. It was designed by Brazilian architect Oscar Niemeyer and calculated by Brazilian structural engineer Joaquim Cardozo, and was completed and dedicated on May 31, 1970. The cathedral is a hyperboloid structure constructed from 16 concrete columns, weighing 90 tons each.

English Gothic is an architectural style that flourished from the late 12th until the mid-17th century. The style was most prominently used in the construction of cathedrals and churches. Gothic architecture's defining features are pointed arches, rib vaults, buttresses, and extensive use of stained glass. Combined, these features allowed the creation of buildings of unprecedented height and grandeur, filled with light from large stained glass windows. Important examples include Westminster Abbey, Canterbury Cathedral and Salisbury Cathedral. The Gothic style endured in England much longer than in Continental Europe.

A parabolic arch is an arch in the shape of a parabola. In structures, their curve represents an efficient method of load, and so can be found in bridges and in architecture in a variety of forms.

The Church Army Chapel at Vanbrugh Park, Blackheath, Greater London, designed by project architect E.T. Spashett for Austin Vernon & Partners, opened in 1965 by Princess Alexandra and consecrated by Michael Ramsey, is a locally listed building of outstanding architectural significance, and is notable for originally having had the tallest sectional aluminium spire of its time, and for being one of the earliest 20th-century chapels of modern design to have been conceived with a central altar. It is now part of Blackheath High School.

Hugh Segar "Sam" Scorer FRIBA FRSA was an English architect who worked in Lincoln, England and was a leading pioneer in the development of hyperbolic paraboloid roof structures using concrete. He also was involved in architectural conservation and research into the work of local 19th-century architects, as well as founding an art gallery in Lincoln, now known as the Sam Scorer Gallery. He held the rare distinction of having two of his buildings listed within his lifetime.

























