Related Research Articles

In geometry, the rhombicuboctahedron, or small rhombicuboctahedron, is an Archimedean solid with eight triangular and eighteen square faces. There are 24 identical vertices, with one triangle and three squares meeting at each one. The polyhedron has octahedral symmetry, like the cube and octahedron. Its dual is called the deltoidal icositetrahedron or trapezoidal icositetrahedron, although its faces are not really true trapezoids.

Packing problems are a class of optimization problems in mathematics that involve attempting to pack objects together into containers. The goal is to either pack a single container as densely as possible or pack all objects using as few containers as possible. Many of these problems can be related to real life packaging, storage and transportation issues. Each packing problem has a dual covering problem, which asks how many of the same objects are required to completely cover every region of the container, where objects are allowed to overlap.
The Kepler conjecture, named after the 17th-century mathematician and astronomer Johannes Kepler, is a mathematical theorem about sphere packing in three-dimensional Euclidean space. It states that no arrangement of equally sized spheres filling space has a greater average density than that of the cubic close packing and hexagonal close packing arrangements. The density of these arrangements is around 74.05%.

In geometry, a sphere packing is an arrangement of non-overlapping spheres within a containing space. The spheres considered are usually all of identical size, and the space is usually three-dimensional Euclidean space. However, sphere packing problems can be generalised to consider unequal spheres, spaces of other dimensions or to non-Euclidean spaces such as hyperbolic space.
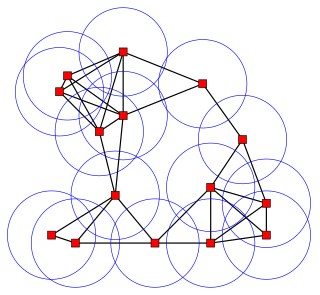
Discrete geometry and combinatorial geometry are branches of geometry that study combinatorial properties and constructive methods of discrete geometric objects. Most questions in discrete geometry involve finite or discrete sets of basic geometric objects, such as points, lines, planes, circles, spheres, polygons, and so forth. The subject focuses on the combinatorial properties of these objects, such as how they intersect one another, or how they may be arranged to cover a larger object.

In geometry, close-packing of equal spheres is a dense arrangement of congruent spheres in an infinite, regular arrangement. Carl Friedrich Gauss proved that the highest average density – that is, the greatest fraction of space occupied by spheres – that can be achieved by a lattice packing is
The Fulkerson Prize for outstanding papers in the area of discrete mathematics is sponsored jointly by the Mathematical Optimization Society (MOS) and the American Mathematical Society (AMS). Up to three awards of $1500 each are presented at each (triennial) International Symposium of the MOS. Originally, the prizes were paid out of a memorial fund administered by the AMS that was established by friends of the late Delbert Ray Fulkerson to encourage mathematical excellence in the fields of research exemplified by his work. The prizes are now funded by an endowment administered by MPS.

A rhombic enneacontahedron is a polyhedron composed of 90 rhombic faces; with three, five, or six rhombi meeting at each vertex. It has 60 broad rhombi and 30 slim. The rhombic enneacontahedron is a zonohedron with a superficial resemblance to the rhombic triacontahedron.
Hilbert's eighteenth problem is one of the 23 Hilbert problems set out in a celebrated list compiled in 1900 by mathematician David Hilbert. It asks three separate questions about lattices and sphere packing in Euclidean space.

Thomas Callister Hales is an American mathematician working in the areas of representation theory, discrete geometry, and formal verification. In representation theory he is known for his work on the Langlands program and the proof of the fundamental lemma over the group Sp(4). In discrete geometry, he settled the Kepler conjecture on the density of sphere packings and the honeycomb conjecture. In 2014, he announced the completion of the Flyspeck Project, which formally verified the correctness of his proof of the Kepler conjecture.
László Fejes Tóth was a Hungarian mathematician who specialized in geometry. He proved that a lattice pattern is the most efficient way to pack centrally symmetric convex sets on the Euclidean plane. He also investigated the sphere packing problem. He was the first to show, in 1953, that proof of the Kepler conjecture can be reduced to a finite case analysis and, later, that the problem might be solved using a computer.
Denis Lawrence Weaire FRS is an Irish physicist and an emeritus professor of Trinity College Dublin (TCD).
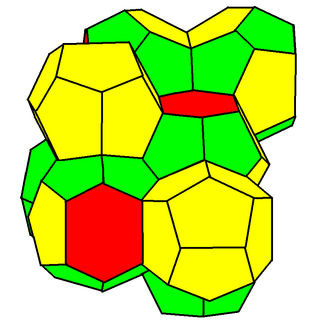
In geometry, the Weaire–Phelan structure is a complex 3-dimensional structure representing an idealised foam of equal-sized bubbles. In 1993, Trinity College Dublin physicist Denis Weaire and his student Robert Phelan found in computer simulations of foam that this structure was a better solution of the "Kelvin problem" than the previous best-known solution, the Kelvin structure.
A computer-assisted proof is a mathematical proof that has been at least partially generated by computer.
The dodecahedral conjecture in geometry is intimately related to sphere packing.

In geometry, circle packing is the study of the arrangement of circles on a given surface such that no overlapping occurs and so that no circle can be enlarged without creating an overlap. The associated packing density, η, of an arrangement is the proportion of the surface covered by the circles. Generalisations can be made to higher dimensions – this is called sphere packing, which usually deals only with identical spheres.

In geometry, the Tammes problem is a problem in packing a given number of circles on the surface of a sphere such that the minimum distance between circles is maximized. It is named after a Dutch botanist who posed the problem in 1930 while studying the distribution of pores on pollen grains. It can be viewed as a particular special case of the generalized Thomson problem.

In the mathematical theory of minimal surfaces, the double bubble conjecture states that the shape that encloses and separates two given volumes and has the minimum possible surface area is a standard double bubble — three spherical surfaces meeting at angles of 2π/3 on a common circle. It is now a theorem, as a proof of it was published in 2002.
Ulam's packing conjecture, named for Stanislaw Ulam, is a conjecture about the highest possible packing density of identical convex solids in three-dimensional Euclidean space. The conjecture says that the optimal density for packing congruent spheres is smaller than that for any other convex body. That is, according to the conjecture, the ball is the convex solid which forces the largest fraction of space to remain empty in its optimal packing structure. This conjecture is therefore related to the Kepler conjecture about sphere packing. Since the solution to the Kepler conjecture establishes that identical balls must leave ≈25.95% of the space empty, Ulam's conjecture is equivalent to the statement that no other convex solid forces that much space to be left empty.
References
- 1 2 3 "Review of The Pursuit of Perfect Packing", MAA Reviews, Mathematical Association of America, August 2008
- 1 2 3 4 Fox, Michael (Jul 2001), "Review of The Pursuit of Perfect Packing", The Mathematical Gazette , 85 (503): 370–372, doi:10.2307/3622070, JSTOR 3622070
- 1 2 3 4 Blundell, S. J. (January 2010), "Review of The Pursuit of Perfect Packing", Contemporary Physics , 51 (1): 94–95, doi:10.1080/00107510903021467
- 1 2 3 4 5 6 Linhart, Johann (2001), "Review of The Pursuit of Perfect Packing", Mathematical Reviews , MR 1786410
- 1 2 Radin, Charles (January 2006), "Review of The Pursuit of Perfect Packing", American Mathematical Monthly , 113 (1): 87–90, doi:10.2307/27641857, JSTOR 27641857