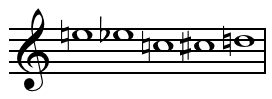In music theory, a scale is any set of musical notes ordered by fundamental frequency or pitch. A scale ordered by increasing pitch is an ascending scale, and a scale ordered by decreasing pitch is a descending scale.
In music theory, an interval is a difference in pitch between two sounds. An interval may be described as horizontal, linear, or melodic if it refers to successively sounding tones, such as two adjacent pitches in a melody, and vertical or harmonic if it pertains to simultaneously sounding tones, such as in a chord.
The chromatic scale is a set of twelve pitches used in tonal music, with notes separated by the interval of a semitone. Chromatic instruments, such as the piano, are made to produce the chromatic scale, while other instruments capable of continuously variable pitch, such as the trombone and violin, can also produce microtones, or notes between those available on a piano.
In music, two written notes have enharmonic equivalence if they produce the same pitch but are notated differently. This relation extends to pitch classes, chords, and key signatures. The term derives from Latin enharmonicus, in turn from Late Latin enarmonius, from Ancient Greek ἐναρμόνιος, from ἐν ('in') and ἁρμονία ('harmony').
An octatonic scale is any eight-note musical scale. However, the term most often refers to the ancohemitonic symmetric scale composed of alternating whole and half steps, as shown at right. In classical theory, this symmetrical scale is commonly called the octatonic scale, although there are a total of 43 enharmonically non-equivalent, transpositionally non-equivalent eight-note sets.
In music, a pitch class (p.c. or pc) is a set of all pitches that are a whole number of octaves apart; for example, the pitch class C consists of the Cs in all octaves. "The pitch class C stands for all possible Cs, in whatever octave position." Important to musical set theory, a pitch class is "all pitches related to each other by octave, enharmonic equivalence, or both." Thus, using scientific pitch notation, the pitch class "C" is the set

Musical set theory provides concepts for categorizing musical objects and describing their relationships. Howard Hanson first elaborated many of the concepts for analyzing tonal music. Other theorists, such as Allen Forte, further developed the theory for analyzing atonal music, drawing on the twelve-tone theory of Milton Babbitt. The concepts of musical set theory are very general and can be applied to tonal and atonal styles in any equal temperament tuning system, and to some extent more generally than that.
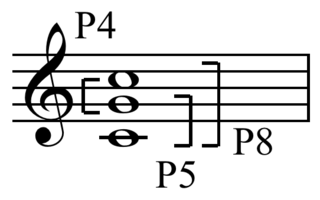
In music theory, complement refers to either traditional interval complementation, or the aggregate complementation of twelve-tone and serialism.

In music, an interval cycle is a collection of pitch classes created from a sequence of the same interval class. In other words, a collection of pitches by starting with a certain note and going up by a certain interval until the original note is reached. In other words, interval cycles "unfold a single recurrent interval in a series that closes with a return to the initial pitch class". See: wikt:cycle.
Modes of limited transposition are musical modes or scales that fulfill specific criteria relating to their symmetry and the repetition of their interval groups. These scales may be transposed to all twelve notes of the chromatic scale, but at least two of these transpositions must result in the same pitch classes, thus their transpositions are "limited". They were compiled by the French composer Olivier Messiaen, and published in his book La technique de mon langage musical.

The mathematical operations of multiplication have several applications to music. Other than its application to the frequency ratios of intervals, it has been used in other ways for twelve-tone technique, and musical set theory. Additionally ring modulation is an electrical audio process involving multiplication that has been used for musical effect.
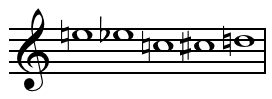
A set in music theory, as in mathematics and general parlance, is a collection of objects. In musical contexts the term is traditionally applied most often to collections of pitches or pitch-classes, but theorists have extended its use to other types of musical entities, so that one may speak of sets of durations or timbres, for example.

In musical set theory, an interval vector is an array of natural numbers which summarize the intervals present in a set of pitch classes. Other names include: ic vector, PIC vector and APIC vector

Music theory analyzes the pitch, timing, and structure of music. It uses mathematics to study elements of music such as tempo, chord progression, form, and meter. The attempt to structure and communicate new ways of composing and hearing music has led to musical applications of set theory, abstract algebra and number theory.

Diatonic and chromatic are terms in music theory that are most often used to characterize scales, and are also applied to musical instruments, intervals, chords, notes, musical styles, and kinds of harmony. They are very often used as a pair, especially when applied to contrasting features of the common practice music of the period 1600–1900.
In music theory, an inversion is a type of change to intervals, chords, voices, and melodies. In each of these cases, "inversion" has a distinct but related meaning. The concept of inversion also plays an important role in musical set theory.

In musical set theory, a Forte number is the pair of numbers Allen Forte assigned to the prime form of each pitch class set of three or more members in The Structure of Atonal Music. The first number indicates the number of pitch classes in the pitch class set and the second number indicates the set's sequence in Forte's ordering of all pitch class sets containing that number of pitches.
In music theory, equivalence class is an equality (=) or equivalence between properties of sets (unordered) or twelve-tone rows. A relation rather than an operation, it may be contrasted with derivation. "It is not surprising that music theorists have different concepts of equivalence [from each other]..." "Indeed, an informal notion of equivalence has always been part of music theory and analysis. Pitch class set theory, however, has adhered to formal definitions of equivalence." Traditionally, octave equivalency is assumed, while inversional, permutational, and transpositional equivalency may or may not be considered.

In music, an all-interval twelve-tone row, series, or chord, is a twelve-tone tone row arranged so that it contains one instance of each interval within the octave, 1 through 11. A "twelve-note spatial set made up of the eleven intervals [between consecutive pitches]." There are 1,928 distinct all-interval twelve-tone rows. These sets may be ordered in time or in register. "Distinct" in this context means in transpositionally and rotationally normal form, and disregarding inversionally related forms. These 1,928 tone rows have been independently rediscovered several times, their first computation probably was by Andre Riotte in 1961.

In musical set theory, there are four kinds of interval: