
In the mathematical field of graph theory, the Petersen graph is an undirected graph with 10 vertices and 15 edges. It is a small graph that serves as a useful example and counterexample for many problems in graph theory. The Petersen graph is named after Julius Petersen, who in 1898 constructed it to be the smallest bridgeless cubic graph with no three-edge-coloring.
In graph theory, an undirected graph H is called a minor of the graph G if H can be formed from G by deleting edges, vertices and by contracting edges.

In hyperbolic geometry, the Klein quartic, named after Felix Klein, is a compact Riemann surface of genus 3 with the highest possible order automorphism group for this genus, namely order 168 orientation-preserving automorphisms, and 168 × 2 = 336 automorphisms if orientation may be reversed. As such, the Klein quartic is the Hurwitz surface of lowest possible genus; see Hurwitz's automorphisms theorem. Its (orientation-preserving) automorphism group is isomorphic to PSL(2, 7), the second-smallest non-abelian simple group after the alternating group A5. The quartic was first described in (Klein 1878b).

In the mathematical field of graph theory, a cubic graph is a graph in which all vertices have degree three. In other words, a cubic graph is a 3-regular graph. Cubic graphs are also called trivalent graphs.
In mathematics, a dessin d'enfant is a type of graph embedding used to study Riemann surfaces and to provide combinatorial invariants for the action of the absolute Galois group of the rational numbers. The name of these embeddings is French for a "child's drawing"; its plural is either dessins d'enfant, "child's drawings", or dessins d'enfants, "children's drawings".

In the mathematical discipline of graph theory, the dual graph of a planar graph G is a graph that has a vertex for each face of G. The dual graph has an edge for each pair of faces in G that are separated from each other by an edge, and a self-loop when the same face appears on both sides of an edge. Thus, each edge e of G has a corresponding dual edge, whose endpoints are the dual vertices corresponding to the faces on either side of e. The definition of the dual depends on the choice of embedding of the graph G, so it is a property of plane graphs rather than planar graphs. For planar graphs generally, there may be multiple dual graphs, depending on the choice of planar embedding of the graph.

In the mathematical field of graph theory, the Gray graph is an undirected bipartite graph with 54 vertices and 81 edges. It is a cubic graph: every vertex touches exactly three edges. It was discovered by Marion C. Gray in 1932 (unpublished), then discovered independently by Bouwer 1968 in reply to a question posed by Jon Folkman 1967. The Gray graph is interesting as the first known example of a cubic graph having the algebraic property of being edge but not vertex transitive.
In combinatorial mathematics, rotation systems encode embeddings of graphs onto orientable surfaces by describing the circular ordering of a graph's edges around each vertex. A more formal definition of a rotation system involves pairs of permutations; such a pair is sufficient to determine a multigraph, a surface, and a 2-cell embedding of the multigraph onto the surface.

In the mathematical field of graph theory, the Hoffman–Singleton graph is a 7-regular undirected graph with 50 vertices and 175 edges. It is the unique strongly regular graph with parameters (50,7,0,1). It was constructed by Alan Hoffman and Robert Singleton while trying to classify all Moore graphs, and is the highest-order Moore graph known to exist. Since it is a Moore graph where each vertex has degree 7, and the girth is 5, it is a (7,5)-cage.
In the mathematical field of graph theory, a quartic graph is a graph where all vertices have degree 4. In other words, a quartic graph is a 4-regular graph.

In the mathematical field of graph theory, the Möbius–Kantor graph is a symmetric bipartite cubic graph with 16 vertices and 24 edges named after August Ferdinand Möbius and Seligmann Kantor. It can be defined as the generalized Petersen graph G(8,3): that is, it is formed by the vertices of an octagon, connected to the vertices of an eight-point star in which each point of the star is connected to the points three steps away from it.
In mathematics, the graph structure theorem is a major result in the area of graph theory. The result establishes a deep and fundamental connection between the theory of graph minors and topological embeddings. The theorem is stated in the seventeenth of a series of 23 papers by Neil Robertson and Paul Seymour. Its proof is very long and involved. Kawarabayashi & Mohar (2007) and Lovász (2006) are surveys accessible to nonspecialists, describing the theorem and its consequences.

In mathematics, a regular map is a symmetric tessellation of a closed surface. More precisely, a regular map is a decomposition of a two-dimensional manifold into topological disks such that every flag can be transformed into any other flag by a symmetry of the decomposition. Regular maps are, in a sense, topological generalizations of Platonic solids. The theory of maps and their classification is related to the theory of Riemann surfaces, hyperbolic geometry, and Galois theory. Regular maps are classified according to either: the genus and orientability of the supporting surface, the underlying graph, or the automorphism group.
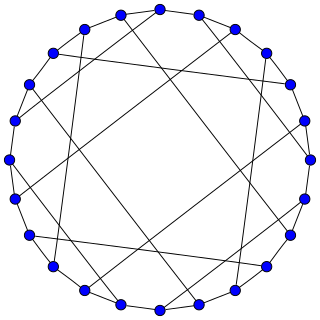
In the mathematical field of graph theory, the Nauru graph is a symmetric, bipartite, cubic graph with 24 vertices and 36 edges. It was named by David Eppstein after the twelve-pointed star in the flag of Nauru.
In the mathematics of infinite graphs, an end of a graph represents, intuitively, a direction in which the graph extends to infinity. Ends may be formalized mathematically as equivalence classes of infinite paths, as havens describing strategies for pursuit–evasion games on the graph, or as topological ends of topological spaces associated with the graph.
In mathematics, a k-ultrahomogeneous graph is a graph in which every isomorphism between two of its induced subgraphs of at most k vertices can be extended to an automorphism of the whole graph. A k-homogeneous graph obeys a weakened version of the same property in which every isomorphism between two induced subgraphs implies the existence of an automorphism of the whole graph that maps one subgraph to the other.

In topological graph theory, the Petrie dual of an embedded graph is another embedded graph that has the Petrie polygons of the first embedding as its faces.

In graph theory, a Xuong tree is a spanning tree of a given graph with the property that, in the remaining graph , the number of connected components with an odd number of edges is as small as possible. They are named after Nguyen Huy Xuong, who used them to characterize the cellular embeddings of a given graph having the largest possible genus.

In topological graph theory, a graph-encoded map or gem is a method of encoding a cellular embedding of a graph using a different graph with four vertices per edge of the original graph. It is the topological analogue of runcination, a geometric operation on polyhedra. Graph-encoded maps were formulated and named by Lins (1982). Alternative and equivalent systems for representing cellular embeddings include signed rotation systems and ribbon graphs.

Tamás Terlaky is a Hungarian-Canadian-American professor of Industrial and Systems Engineering at Lehigh University. He is especially well known for his work on criss-cross algorithms, interior-point methods, Klee-Minty examples for path following algorithms, and optimization.














