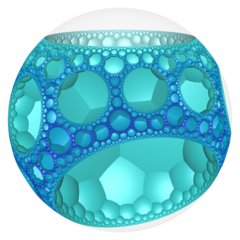
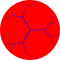
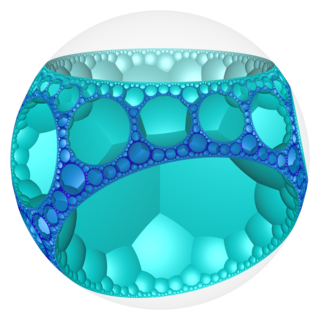
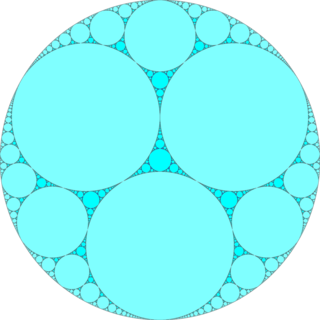
In the geometry of hyperbolic 3-space, the order-7 tetrahedral honeycomb is a regular space-filling tessellation with Schläfli symbol {3,3,7}. It has seven tetrahedra {3,3} around each edge. All vertices are ultra-ideal with infinitely many tetrahedra existing around each vertex in an order-7 triangular tiling vertex arrangement.
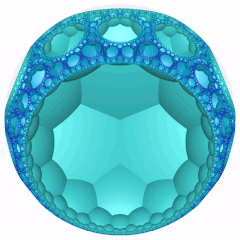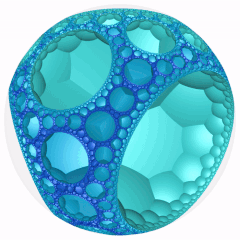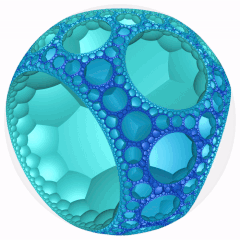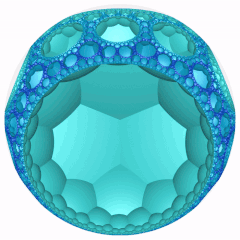
In the geometry of hyperbolic 3-space, the order-7 dodecahedral honeycomb is a regular space-filling tessellation.

In the geometry of hyperbolic 3-space, the order-3-7 hexagonal honeycomb or a regular space-filling tessellation with Schläfli symbol {6,3,7}.
In the geometry of hyperbolic 3-space, the order-7 cubic honeycomb is a regular space-filling tessellation. With Schläfli symbol {4,3,7}, it has seven cubes {4,3} around each edge. All vertices are ultra-ideal with infinitely many cubes existing around each vertex in an order-7 triangular tiling vertex arrangement.
In the geometry of hyperbolic 3-space, the order-3-4 heptagonal honeycomb or 7,3,4 honeycomb a regular space-filling tessellation. Each infinite cell consists of a heptagonal tiling whose vertices lie on a 2-hypercycle, each of which has a limiting circle on the ideal sphere.
In the geometry of hyperbolic 3-space, the order-3-5 heptagonal honeycomb a regular space-filling tessellation. Each infinite cell consists of a heptagonal tiling whose vertices lie on a 2-hypercycle, each of which has a limiting circle on the ideal sphere.
In the geometry of hyperbolic 3-space, the order-3-6 heptagonal honeycomb a regular space-filling tessellation. Each infinite cell consists of a heptagonal tiling whose vertices lie on a 2-hypercycle, each of which has a limiting circle on the ideal sphere.
In the geometry of hyperbolic 3-space, the order-3-7 heptagonal honeycomb a regular space-filling tessellation with Schläfli symbol {7,3,7}.
In the geometry of hyperbolic 3-space, the order-5 octahedral honeycomb is a regular space-filling tessellation with Schläfli symbol {3,4,5}. It has five octahedra {3,4} around each edge. All vertices are ultra-ideal with infinitely many octahedra existing around each vertex in an order-5 square tiling vertex arrangement.
In the geometry of hyperbolic 3-space, the order-4 icosahedral honeycomb is a regular space-filling tessellation with Schläfli symbol {3,5,4}.
In the geometry of hyperbolic 3-space, the order-4-5 square honeycomb is a regular space-filling tessellation with Schläfli symbol {4,4,5}. It has five square tiling {4,4} around each edge. All vertices are ultra-ideal with infinitely many square tiling existing around each vertex in an order-5 square tiling vertex arrangement.
In the geometry of hyperbolic 3-space, the order-4-3 pentagonal honeycomb or 5,4,3 honeycomb is a regular space-filling tessellation. Each infinite cell is an order-4 pentagonal tiling whose vertices lie on a 2-hypercycle, each of which has a limiting circle on the ideal sphere.
In the geometry of hyperbolic 3-space, the order-4-4 pentagonal honeycomb a regular space-filling tessellation. Each infinite cell consists of a pentagonal tiling whose vertices lie on a 2-hypercycle, each of which has a limiting circle on the ideal sphere.
In the geometry of hyperbolic 3-space, the order-5-3 square honeycomb or 4,5,3 honeycomb a regular space-filling tessellation. Each infinite cell consists of a pentagonal tiling whose vertices lie on a 2-hypercycle, each of which has a limiting circle on the ideal sphere.
In the geometry of hyperbolic 3-space, the order-5-4 square honeycomb a regular space-filling tessellation with Schläfli symbol {4,5,4}.
In the geometry of hyperbolic 3-space, the order-7-3 triangular honeycomb is a regular space-filling tessellation with Schläfli symbol {3,7,3}.
In the geometry of hyperbolic 3-space, the order-6-3 square honeycomb or 4,6,3 honeycomb is a regular space-filling tessellation. Each infinite cell consists of a hexagonal tiling whose vertices lie on a 2-hypercycle, each of which has a limiting circle on the ideal sphere.
In the geometry of hyperbolic 3-space, the order-6-4 square honeycomb a regular space-filling tessellation with Schläfli symbol {4,6,4}.
In the geometry of hyperbolic 3-space, the order-8-3 triangular honeycomb is a regular space-filling tessellation with Schläfli symbol {3,8,3}.
In the geometry of hyperbolic 3-space, the order-infinite-3 triangular honeycomb is a regular space-filling tessellation with Schläfli symbol {3,∞,3}.