Logic programming is a programming, database and knowledge representation paradigm based on formal logic. A logic program is a set of sentences in logical form, representing knowledge about some problem domain. Computation is performed by applying logical reasoning to that knowledge, to solve problems in the domain. Major logic programming language families include Prolog, Answer Set Programming (ASP) and Datalog. In all of these languages, rules are written in the form of clauses:

In computer science and operations research, a genetic algorithm (GA) is a metaheuristic inspired by the process of natural selection that belongs to the larger class of evolutionary algorithms (EA). Genetic algorithms are commonly used to generate high-quality solutions to optimization and search problems by relying on biologically inspired operators such as mutation, crossover and selection. Some examples of GA applications include optimizing decision trees for better performance, solving sudoku puzzles, hyperparameter optimization, causal inference, etc.

Breadth-first search (BFS) is an algorithm for searching a tree data structure for a node that satisfies a given property. It starts at the tree root and explores all nodes at the present depth prior to moving on to the nodes at the next depth level. Extra memory, usually a queue, is needed to keep track of the child nodes that were encountered but not yet explored.
A* is a graph traversal and pathfinding algorithm, which is used in many fields of computer science due to its completeness, optimality, and optimal efficiency. Given a weighted graph, a source node and a goal node, the algorithm finds the shortest path from source to goal.
In computer science, declarative programming is a programming paradigm—a style of building the structure and elements of computer programs—that expresses the logic of a computation without describing its control flow.
Backtracking is a class of algorithms for finding solutions to some computational problems, notably constraint satisfaction problems, that incrementally builds candidates to the solutions, and abandons a candidate ("backtracks") as soon as it determines that the candidate cannot possibly be completed to a valid solution.
In computer science, a topological sort or topological ordering of a directed graph is a linear ordering of its vertices such that for every directed edge (u,v) from vertex u to vertex v, u comes before v in the ordering. For instance, the vertices of the graph may represent tasks to be performed, and the edges may represent constraints that one task must be performed before another; in this application, a topological ordering is just a valid sequence for the tasks. Precisely, a topological sort is a graph traversal in which each node v is visited only after all its dependencies are visited. A topological ordering is possible if and only if the graph has no directed cycles, that is, if it is a directed acyclic graph (DAG). Any DAG has at least one topological ordering, and algorithms are known for constructing a topological ordering of any DAG in linear time. Topological sorting has many applications, especially in ranking problems such as feedback arc set. Topological sorting is possible even when the DAG has disconnected components.
GOMS is a specialized human information processor model for human-computer interaction observation that describes a user's cognitive structure on four components. In the book The Psychology of Human Computer Interaction. written in 1983 by Stuart K. Card, Thomas P. Moran and Allen Newell, the authors introduce: "a set of Goals, a set of Operators, a set of Methods for achieving the goals, and a set of Selections rules for choosing among competing methods for goals." GOMS is a widely used method by usability specialists for computer system designers because it produces quantitative and qualitative predictions of how people will use a proposed system.
Automated planning and scheduling, sometimes denoted as simply AI planning, is a branch of artificial intelligence that concerns the realization of strategies or action sequences, typically for execution by intelligent agents, autonomous robots and unmanned vehicles. Unlike classical control and classification problems, the solutions are complex and must be discovered and optimized in multidimensional space. Planning is also related to decision theory.
The Planning Domain Definition Language (PDDL) is an attempt to standardize Artificial Intelligence (AI) planning languages. It was first developed by Drew McDermott and his colleagues in 1998 mainly to make the 1998/2000 International Planning Competition (IPC) possible, and then evolved with each competition. The standardization provided by PDDL has the benefit of making research more reusable and easily comparable, though at the cost of some expressive power, compared to domain-specific systems.
The Stanford Research Institute Problem Solver, known by its acronym STRIPS, is an automated planner developed by Richard Fikes and Nils Nilsson in 1971 at SRI International. The same name was later used to refer to the formal language of the inputs to this planner. This language is the base for most of the languages for expressing automated planning problem instances in use today; such languages are commonly known as action languages. This article only describes the language, not the planner.
In artificial intelligence, hierarchical task network (HTN) planning is an approach to automated planning in which the dependency among actions can be given in the form of hierarchically structured networks.

In computer science, recursion is a method of solving a computational problem where the solution depends on solutions to smaller instances of the same problem. Recursion solves such recursive problems by using functions that call themselves from within their own code. The approach can be applied to many types of problems, and recursion is one of the central ideas of computer science.
The power of recursion evidently lies in the possibility of defining an infinite set of objects by a finite statement. In the same manner, an infinite number of computations can be described by a finite recursive program, even if this program contains no explicit repetitions.
Motion planning, also path planning is a computational problem to find a sequence of valid configurations that moves the object from the source to destination. The term is used in computational geometry, computer animation, robotics and computer games.

A schedule or a timetable, as a basic time-management tool, consists of a list of times at which possible tasks, events, or actions are intended to take place, or of a sequence of events in the chronological order in which such things are intended to take place. The process of creating a schedule — deciding how to order these tasks and how to commit resources between the variety of possible tasks — is called scheduling, and a person responsible for making a particular schedule may be called a scheduler. Making and following schedules is an ancient human activity.
In computer science, graph traversal refers to the process of visiting each vertex in a graph. Such traversals are classified by the order in which the vertices are visited. Tree traversal is a special case of graph traversal.
In software engineering, graphical user interface testing is the process of testing a product's graphical user interface (GUI) to ensure it meets its specifications. This is normally done through the use of a variety of test cases.
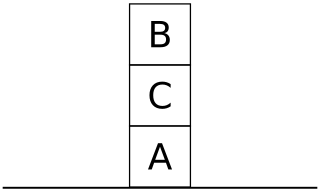
The Sussman anomaly is a problem in artificial intelligence, first described by Gerald Sussman, that illustrates a weakness of noninterleaved planning algorithms, which were prominent in the early 1970s. Most modern planning systems are not restricted to noninterleaved planning and thus can handle this anomaly. While the significance/value of the problem is now a historical one, it is still useful for explaining why planning is non-trivial. In the problem, three blocks rest on a table. The agent must stack the blocks such that A is atop B, which in turn is atop C. However, it may only move one block at a time. The problem starts with B on the table, C atop A, and A on the table:
In artificial intelligence, action description language (ADL) is an automated planning and scheduling system in particular for robots. It is considered an advancement of STRIPS. Edwin Pednault proposed this language in 1987. It is an example of an action language.
Action model learning is an area of machine learning concerned with creation and modification of software agent's knowledge about effects and preconditions of the actions that can be executed within its environment. This knowledge is usually represented in logic-based action description language and used as the input for automated planners.




