Product of group subsets

Last updated

In mathematics, one can define a product of group subsets in a natural way. If S and T are subsets of a group G, then their product is the subset of G defined by

Contents

The subsets S and T need not be subgroups for this product to be well defined. The associativity of this product follows from that of the group product. The product of group subsets therefore defines a natural monoid structure on the power set of G.

A lot more can be said in the case where S and T are subgroups. The product of two subgroups S and T of a group G is itself a subgroup of G if and only if ST = TS.

Product of subgroups

If S and T are subgroups of G, their product need not be a subgroup (for example, two distinct subgroups of order 2 in the symmetric group on 3 symbols). This product is sometimes called the Frobenius product. [1] In general, the product of two subgroups S and T is a subgroup if and only if ST = TS, [2] and the two subgroups are said to permute. (Walter Ledermann has called this fact the Product Theorem, [3] but this name, just like "Frobenius product" is by no means standard.) In this case, ST is the group generated by S and T; i.e., ST = TS = ⟨S ∪ T⟩.

If either S or T is normal then the condition ST = TS is satisfied and the product is a subgroup. [4] [5] If both S and T are normal, then the product is normal as well. [4]

If S and T are finite subgroups of a group G, then ST is a subset of G of size |ST| given by the product formula:

Note that this applies even if neither S nor T is normal.

Modular law

The following modular law (for groups) holds for any Q a subgroup of S, where T is any other arbitrary subgroup (and both S and T are subgroups of some group G):

Q(S ∩ T) = S ∩ (QT).

The two products that appear in this equality are not necessarily subgroups.

If QT is a subgroup (equivalently, as noted above, if Q and T permute) then QT = ⟨Q ∪ T⟩ = Q ∨ T; i.e., QT is the join of Q and T in the lattice of subgroups of G, and the modular law for such a pair may also be written as Q ∨ (S ∩ T) = S ∩ (Q ∨ T), which is the equation that defines a modular lattice if it holds for any three elements of the lattice with Q ≤ S. In particular, since normal subgroups permute with each other, they form a modular sublattice.

A group in which every subgroup permutes is called an Iwasawa group. The subgroup lattice of an Iwasawa group is thus a modular lattice, so these groups are sometimes called modular groups [6] (although this latter term may have other meanings.)

The assumption in the modular law for groups (as formulated above) that Q is a subgroup of S is essential. If Q is not a subgroup of S, then the tentative, more general distributive property that one may consider S ∩ (QT) = (S ∩ Q)(S ∩ T) is false. [7] [8]

Product of subgroups with trivial intersection

In particular, if S and T intersect only in the identity, then every element of ST has a unique expression as a product st with s in S and t in T. If S and T also commute, then ST is a group, and is called a Zappa–Szép product. Even further, if S or T is normal in ST, then ST coincides with the semidirect product of S and T. Finally, if both S and T are normal in ST, then ST coincides with the direct product of S and T.

If S and T are subgroups whose intersection is the trivial subgroup (identity element) and additionally ST = G, then S is called a complement of T and vice versa.

By a (locally unambiguous) abuse of terminology, two subgroups that intersect only on the (otherwise obligatory) identity are sometimes called disjoint. [9]

Product of subgroups with non-trivial intersection

A question that arises in the case of a non-trivial intersection between a normal subgroup N and a subgroup K is what is the structure of the quotient NK/N. Although one might be tempted to just "cancel out" N and say the answer is K, that is not correct because a homomorphism with kernel N will also "collapse" (map to 1) all elements of K that happen to be in N. Thus the correct answer is that NK/N is isomorphic with K/(N∩K). This fact is sometimes called the second isomorphism theorem, [10] (although the numbering of these theorems sees some variation between authors); it has also been called the diamond theorem by I. Martin Isaacs because of the shape of subgroup lattice involved, [11] and has also been called the parallelogram rule by Paul Moritz Cohn, who thus emphasized analogy with the parallelogram rule for vectors because in the resulting subgroup lattice the two sides assumed to represent the quotient groups (SN) / N and S / (S ∩ N) are "equal" in the sense of isomorphism. [12]

Frattini's argument guarantees the existence of a product of subgroups (giving rise to the whole group) in a case where the intersection is not necessarily trivial (and for this latter reason the two subgroups are not complements). More specifically, if G is a finite group with normal subgroup N, and if P is a Sylow p-subgroup of N, then G = NG(P)N, where NG(P) denotes the normalizer of P in G. (Note that the normalizer of P includes P, so the intersection between N and NG(P) is at least P.)

Generalization to semigroups

In a semigroup S, the product of two subsets defines a structure of a semigroup on P(S), the power set of the semigroup S; furthermore P(S) is a semiring with addition as union (of subsets) and multiplication as product of subsets. [13]

See also

Related Research Articles

Normal subgroup Subgroup invariant under conjugation

In abstract algebra, a normal subgroup is a subgroup that is invariant under conjugation by members of the group of which it is a part. In other words, a subgroup of the group is normal in if and only if for all and The usual notation for this relation is

Semigroup Algebraic structure consisting of a set with an associative binary operation

In mathematics, a semigroup is an algebraic structure consisting of a set together with an associative binary operation.

Subgroup Subset of a group that forms a group itself

In group theory, a branch of mathematics, given a group G under a binary operation ∗, a subset H of G is called a subgroup of G if H also forms a group under the operation ∗. More precisely, H is a subgroup of G if the restriction of ∗ to H × H is a group operation on H. This is often denoted H ≤ G, read as "H is a subgroup of G".

In abstract algebra, a congruence relation is an equivalence relation on an algebraic structure that is compatible with the structure in the sense that algebraic operations done with equivalent elements will yield equivalent elements. Every congruence relation has a corresponding quotient structure, whose elements are the equivalence classes for the relation.

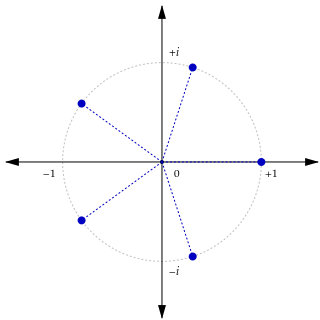
Generating set of a group Abstract algebra concept

In abstract algebra, a generating set of a group is a subset of the group set such that every element of the group can be expressed as a combination of finitely many elements of the subset and their inverses.

General linear group Set of n×n invertible matrices

In mathematics, the general linear group of degree n is the set of n×n invertible matrices, together with the operation of ordinary matrix multiplication. This forms a group, because the product of two invertible matrices is again invertible, and the inverse of an invertible matrix is invertible, with identity matrix as the identity element of the group. The group is so named because the columns of an invertible matrix are linearly independent, hence the vectors/points they define are in general linear position, and matrices in the general linear group take points in general linear position to points in general linear position.

In mathematics, especially group theory, the centralizer of a subset S in a group G is the set of elements of G such that each member commutes with each element of S, or equivalently, such that conjugation by leaves each element of S fixed. The normalizer of S in G is the set of elements of G that satisfy the weaker condition of leaving the set fixed under conjugation. The centralizer and normalizer of S are subgroups of G. Many techniques in group theory are based on studying the centralizers and normalizers of suitable subsets S.

Glossary of group theory

A group is a set together with an associative operation which admits an identity element and such that every element has an inverse.

In mathematics, a building is a combinatorial and geometric structure which simultaneously generalizes certain aspects of flag manifolds, finite projective planes, and Riemannian symmetric spaces. Buildings were initially introduced by Jacques Tits as a means to understand the structure of exceptional groups of Lie type. The more specialized theory of Bruhat–Tits buildings plays a role in the study of p-adic Lie groups analogous to that of the theory of symmetric spaces in the theory of Lie groups.

In mathematics, a congruence subgroup of a matrix group with integer entries is a subgroup defined by congruence conditions on the entries. A very simple example would be invertible 2 × 2 integer matrices of determinant 1, in which the off-diagonal entries are even. More generally, the notion of congruence subgroup can be defined for arithmetic subgroups of algebraic groups; that is, those for which we have a notion of 'integral structure' and can define reduction maps modulo an integer.

In mathematics, the Iwahori–Hecke algebra, or Hecke algebra, named for Erich Hecke and Nagayoshi Iwahori, is a deformation of the group algebra of a Coxeter group.

Arithmetic group

In mathematics, an arithmetic group is a group obtained as the integer points of an algebraic group, for example They arise naturally in the study of arithmetic properties of quadratic forms and other classical topics in number theory. They also give rise to very interesting examples of Riemannian manifolds and hence are objects of interest in differential geometry and topology. Finally, these two topics join in the theory of automorphic forms which is fundamental in modern number theory.

Reductive group

In mathematics, a reductive group is a type of linear algebraic group over a field. One definition is that a connected linear algebraic group G over a perfect field is reductive if it has a representation with finite kernel which is a direct sum of irreducible representations. Reductive groups include some of the most important groups in mathematics, such as the general linear group GL(n) of invertible matrices, the special orthogonal group SO(n), and the symplectic group Sp(2n). Simple algebraic groups and semisimple algebraic groups are reductive.

Modular lattice

In the branch of mathematics called order theory, a modular lattice is a lattice that satisfies the following self-dual condition,

In mathematics, in the field of group theory, a quasinormal subgroup, or permutable subgroup, is a subgroup of a group that commutes (permutes) with every other subgroup with respect to the product of subgroups. The term quasinormal subgroup was introduced by Øystein Ore in 1937.

Lattice of subgroups

In mathematics, the lattice of subgroups of a group is the lattice whose elements are the subgroups of , with the partial order relation being set inclusion. In this lattice, the join of two subgroups is the subgroup generated by their union, and the meet of two subgroups is their intersection.

Lattice (discrete subgroup)

In Lie theory and related areas of mathematics, a lattice in a locally compact group is a discrete subgroup with the property that the quotient space has finite invariant measure. In the special case of subgroups of Rn, this amounts to the usual geometric notion of a lattice as a periodic subset of points, and both the algebraic structure of lattices and the geometry of the space of all lattices are relatively well understood.

In mathematics, a group is called an Iwasawa group, M-group or modular group if its lattice of subgroups is modular. Alternatively, a group G is called an Iwasawa group when every subgroup of G is permutable in G.

In mathematics, the Krull–Schmidt theorem states that a group subjected to certain finiteness conditions on chains of subgroups, can be uniquely written as a finite direct product of indecomposable subgroups.

References

  1. ↑ Adolfo Ballester-Bolinches; Ramon Esteban-Romero; Mohamed Asaad (2010). Products of Finite Groups . Walter de Gruyter. p.  1. ISBN   978-3-11-022061-2.
  2. ↑ W. Keith Nicholson (2012). Introduction to Abstract Algebra (4th ed.). John Wiley & Sons. Lemma 2, p. 125. ISBN   978-1-118-13535-8.
  3. ↑ Walter Ledermann, Introduction to Group Theory, 1976, Longman, ISBN   0-582-44180-3, p. 52
  4. 1 2 Nicholson, 2012, Theorem 5, p. 125
  5. ↑ David A.R. Wallace (1998). Groups, Rings and Fields. Springer Science & Business Media. Theorem 14, p. 123. ISBN   978-3-540-76177-8.
  6. ↑ Ballester-Bolinches, Esteban-Romero, Asaad, p. 24
  7. ↑ Derek Robinson (1996). A Course in the Theory of Groups. Springer Science & Business Media. p. 15. ISBN   978-0-387-94461-6.
  8. ↑ Paul Moritz Cohn (2000). Classic algebra . Wiley. pp.  248. ISBN   978-0-471-87731-8.
  9. ↑ L. Fuchs (1970). Infinite Abelian Groups. Volume I. Academic Press. p. 37. ISBN   978-0-08-087348-0.
  10. ↑ Dan Saracino (1980). Abstract Algebra: A First Course . Addison-Wesley. p.  123. ISBN   0-201-07391-9.
  11. ↑ I. Martin Isaacs (1994). Algebra: A Graduate Course . American Mathematical Soc. p.  33. ISBN   978-0-8218-4799-2.
  12. ↑ Paul Moritz Cohn (2000). Classic Algebra . Wiley. p.  245. ISBN   978-0-471-87731-8.
  13. ↑ Jean E. Pin (1989). Formal Properties of Finite Automata and Applications: LITP Spring School on Theoretical Computer Science, Ramatuelle, France, May 23–27, 1988. Proceedings. Springer Science & Business Media. p. 35. ISBN   978-3-540-51631-6.
This page is based on this Wikipedia article
Text is available under the CC BY-SA 4.0 license; additional terms may apply.
Images, videos and audio are available under their respective licenses.