The Pyraminx Duo (originally known as Rob's Pyraminx) [1] is a tetrahedral twisty puzzle in the style of the Rubik's Cube. It was suggested by Rob Stegmann, [1] invented by Oskar van Deventer, [1] [2] and has now been mass-produced by Meffert's. [1] [3]
The Pyraminx Duo is a puzzle in the shape of a tetrahedron, divided into 4 corner pieces and 4 face centre pieces. Each corner piece has three colours, while the centre pieces each have a single colour. Each face of the puzzle contains one face centre piece and three corner pieces.
The puzzle can be thought of as twisting around its corner pieces - each twist rotates one corner piece and permutates the three face centre pieces around it. An interesting feature is that the face centre pieces go "underneath" corner pieces during a twist.
The purpose of the puzzle is to scramble the colours, and then restore them to their original configuration of one colour per face.
Mechanically, the puzzle is similar to the Skewb, with all corner pieces of the Skewb visible (although shaped differently) and all centre pieces hidden.
There are 4 corner pieces. Each corner can be twisted in 3 different orientations, independently of the other corners. Therefore, the corners can be orientated in 34 different ways. They cannot be permutated, therefore there is only one possible corner permutation.
There are 4 face centre pieces. These can be permutated in at most 4! different ways. However, the exact number of these permutations is not yet reached due to two constraints. The first constraint is that only even permutations of the face centers are possible (e.g. it is impossible to have only two face centre pieces swapped); this divides the limit by 2. The second constraint is that all centre permutations are dependent on the orientation of the corner pieces. Some permutations of centres are only possible when the total number of clockwise rotations of corner pieces is divisible by 3; other permutations are only possible when the total number of clockwise rotations is equivalent to 1 modulo 3; others are only possible when the number is equivalent to 2 modulo 3. This divides the limit by 3.
The face centre pieces have no obvious orientation, therefore this does not affect the total number of combinations.
The full number is therefore: [4]
This number, in relative terms, is extremely low compared to other puzzles like the Rubik's Cube (which has over 43 quintillion combinations), the Pocket Cube (with over 3.6 million combinations), or even the Pyraminx (with just over 930 thousand combinations, excluding rotations of the trivial tips).
As explained above, the total number of possible configurations of the Pyraminx Duo is 324, which is sufficiently small to allow a computer search for optimal solutions. The table below summarises the result of such a search, stating the number p of positions that require n twists to solve the Pyraminx Duo: [4]
| n | 0 | 1 | 2 | 3 | 4 | Total |
|---|---|---|---|---|---|---|
| p | 1 | 8 | 48 | 188 | 79 | 324 |
The above table shows that the God's Number of the Pyraminx Duo is 4 (i.e. the puzzle is always at most 4 twists away from its solved state). Similarly to the total number of combinations, this number is very low compared to the Rubik's Cube (20), the Pocket Cube (11) or the Pyraminx (11, excluding the trivial tips).
Due to its substantially low number of combinations and its low God's Number, the Pyraminx Duo is a relatively easy puzzle to solve; it has been described as "arguably the easiest non-trivial twisty puzzle". [2] Because of this, cubers usually come up with their own methods of solving the puzzle. For an extra challenge, it is also not uncommon for cubers to invent their own "optimal" methods - i.e. methods that guarantee to solve the puzzle in no more than 4 moves.
There are several variations of the Pyraminx Duo that have been invented. These variations all look the same as the original puzzle but use different colour schemes; usually these colour schemes make the orientations of the face centre pieces visible, which makes the puzzle slightly more challenging. [4]
The Rubik's Cube is a 3D combination puzzle invented in 1974 by Hungarian sculptor and professor of architecture Ernő Rubik. Originally called the Magic Cube, the puzzle was licensed by Rubik to be sold by Pentangle Puzzles in the UK in 1978, and then by Ideal Toy Corp in 1980 via businessman Tibor Laczi and Seven Towns founder Tom Kremer. The cube was released internationally in 1980 and became one of the most recognized icons in popular culture. It won the 1980 German Game of the Year special award for Best Puzzle. As of January 2024, around 500 million cubes had been sold worldwide, making it the world's bestselling puzzle game and bestselling toy. The Rubik's Cube was inducted into the US National Toy Hall of Fame in 2014.
The Rubik's Revenge is a 4×4×4 version of the Rubik's Cube. It was released in 1981. Invented by Péter Sebestény, the cube was nearly called the Sebestény Cube until a somewhat last-minute decision changed the puzzle's name to attract fans of the original Rubik's Cube. Unlike the original puzzle, it has no fixed faces: the center faces are free to move to different positions.
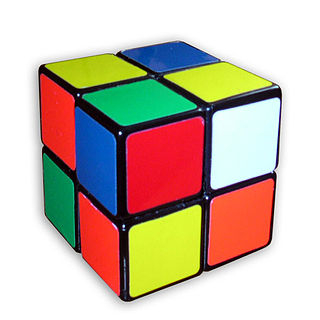
The Pocket Cube is a 2×2×2 combination puzzle invented in 1970 by American puzzle designer Larry D. Nichols. The cube consists of 8 pieces, all corners.
The Professor's Cube is a 5×5×5 version of the original Rubik's Cube. It has qualities in common with both the 3×3×3 Rubik's Cube and the 4×4×4 Rubik's Revenge, and solution strategies for both can be applied.
The Pyraminx is a regular tetrahedron puzzle in the style of Rubik's Cube. It was made and patented by Uwe Mèffert after the original 3 layered Rubik's Cube by Ernő Rubik, and introduced by Tomy Toys of Japan in 1981.
The Square-1 is a variant of the Rubik's Cube. Its distinguishing feature among the numerous Rubik's Cube variants is that it can change shape as it is twisted, due to the way it is cut, thus adding an extra level of challenge and difficulty. The Super Square One and Square Two puzzles have also been introduced. The Super Square One has two additional layers that can be scrambled and solved independently of the rest of the puzzle, and the Square Two has extra cuts made to the top and bottom layer, making the edge and corner wedges the same size.
The Megaminx or Mégaminx is a dodecahedron-shaped puzzle similar to the Rubik's Cube. It has a total of 50 movable pieces to rearrange, compared to the 20 movable pieces of the Rubik's Cube.
The Dogic is an icosahedron-shaped puzzle like the Rubik's Cube. The 5 triangles meeting at its tips may be rotated, or 5 entire faces around the tip may be rotated. It has a total of 80 movable pieces to rearrange, compared to the 20 pieces in the Rubik's Cube.
The Skewb Diamond is an octahedron-shaped combination puzzle similar to the Rubik's Cube. It has 14 movable pieces which can be rearranged in a total of 138,240 possible combinations. This puzzle is the dual polyhedron of the Skewb. It was invented by Uwe Mèffert, a German puzzle inventor and designer.
The Skewb is a combination puzzle and a mechanical puzzle in the style of the Rubik's Cube. It was invented by Tony Durham and marketed by Uwe Mèffert. Although it is cubical, it differs from the typical cubes' construction; its axes of rotation pass through the corners of the cube, rather than the centers of the faces. There are four axes, one for each space diagonal of the cube. As a result, it is a deep-cut puzzle in which each twist affects all six faces.
The Skewb Ultimate, originally marketed as the Pyraminx Ball, is a twelve-sided puzzle derivation of the Skewb, produced by German toy-maker Uwe Mèffert. Most versions of this puzzle are sold with six different colors of stickers attached, with opposite sides of the puzzle having the same color; however, some early versions of the puzzle have a full set of 12 colors.
The Pyramorphix, also called Pyramorphinx, is a tetrahedral puzzle similar to the Rubik's Cube. It has a total of 8 movable pieces to rearrange, compared to the 20 of the Rubik's Cube. Although it looks like a trivially simple version of the Pyraminx, it is an edge-turning puzzle with the mechanism identical to that of the Pocket Cube.
The V-Cube 6 is a 6×6×6 version of the original Rubik's Cube. The first mass-produced 6×6×6 was invented by Panagiotis Verdes and is produced by the Greek company Verdes Innovations SA. Other such puzzles have since been introduced by a number of Chinese companies, most of which have mechanisms which improve on the original. Unlike the original puzzle, it has no fixed facets: the center facets are free to move to different positions.
The Pyraminx Crystal is a dodecahedral puzzle similar to the Rubik's Cube and the Megaminx. It is manufactured by Uwe Mèffert and has been sold in his puzzle shop since 2008.
The Helicopter Cube is a Rubik's Cube-like puzzle invented by Adam G. Cowan in 2005 and built in 2006. It is also in the shape of a cube. At first glance, the Helicopter Cube may seem like a combination of the 2x2x2 and the Skewb, but it actually cuts differently, and twists around cube edges rather than cube faces. The purpose of the puzzle is to scramble the colors, and then restore them back to their original state of a single color per face.
A Tuttminx is a Rubik's Cube-like twisty puzzle, in the shape of a truncated icosahedron. It was invented by Lee Tutt in 2005. It has a total of 150 movable pieces to rearrange, compared to 20 movable pieces of the Rubik's Cube.
Oskar van Deventer is a Dutch puzzle maker. He prototypes puzzles using 3D printing. His work combines mathematics, physics, and design, and he collaborates at academic institutions. Many of his combination puzzles are in mass production by Uwe Mèffert and WitEden. Oskar van Deventer has also designed puzzles for Hanayama.
The Nine-Colour Cube is a cubic twisty puzzle. It was invented in 2005 by Milan Vodicka and mass-produced by Meffert's seven years later. Mechanically, the puzzle is identical to the Rubik's Cube; however, unlike the 3×3×3 Rubik's Cube, which only has 6 different colours, the Nine-Colour Cube has 9 colours, with the individual pieces having one colour each.
The V-Cube 8 is an 8×8×8 version of the Rubik's Cube. Unlike the original puzzle, it has no fixed facets: the center facets are free to move to different positions. The design was covered by Panagiotis Verdes' patent from 2007 but Verdes Innovations SA did not produce it for sale until 2014. Other manufacturers released their own versions of the puzzle much earlier.
The Dino Cube is a cubic twisty puzzle in the style of the Rubik's Cube. It was invented in 1985 by Robert Webb, though it was not mass-produced until ten years later. It has a total of 12 external movable pieces to rearrange, compared to 20 movable pieces on the Rubik's Cube.
