In geometry, a polyhedron is a three-dimensional shape with flat polygonal faces, straight edges and sharp corners or vertices.

The discipline of origami or paper folding has received a considerable amount of mathematical study. Fields of interest include a given paper model's flat-foldability, and the use of paper folds to solve up-to cubic mathematical equations.

Modular origami or unit origami is a two-stage paper folding technique in which several, or sometimes many, sheets of paper are first folded into individual modules or units and then assembled into an integrated flat shape or three-dimensional structure, usually by inserting flaps into pockets created by the folding process. These insertions create tension or friction that holds the model together.

In geometry, a net of a polyhedron is an arrangement of non-overlapping edge-joined polygons in the plane which can be folded to become the faces of the polyhedron. Polyhedral nets are a useful aid to the study of polyhedra and solid geometry in general, as they allow for physical models of polyhedra to be constructed from material such as thin cardboard.
Cauchy's theorem is a theorem in geometry, named after Augustin Cauchy. It states that convex polytopes in three dimensions with congruent corresponding faces must be congruent to each other. That is, any polyhedral net formed by unfolding the faces of the polyhedron onto a flat surface, together with gluing instructions describing which faces should be connected to each other, uniquely determines the shape of the original polyhedron. For instance, if six squares are connected in the pattern of a cube, then they must form a cube: there is no convex polyhedron with six square faces connected in the same way that does not have the same shape.

In geometry, a flexible polyhedron is a polyhedral surface without any boundary edges, whose shape can be continuously changed while keeping the shapes of all of its faces unchanged. The Cauchy rigidity theorem shows that in dimension 3 such a polyhedron cannot be convex.

Kawasaki's theorem or Kawasaki–Justin theorem is a theorem in the mathematics of paper folding that describes the crease patterns with a single vertex that may be folded to form a flat figure. It states that the pattern is flat-foldable if and only if alternatingly adding and subtracting the angles of consecutive folds around the vertex gives an alternating sum of zero. Crease patterns with more than one vertex do not obey such a simple criterion, and are NP-hard to fold.

In geometry, a Bricard octahedron is a member of a family of flexible polyhedra constructed by Raoul Bricard in 1897. The overall shape of one of these polyhedron may change in a continuous motion, without any changes to the lengths of its edges nor to the shapes of its faces. These octahedra were the first flexible polyhedra to be discovered.
Joseph O'Rourke is the Spencer T. and Ann W. Olin Professor of Computer Science at Smith College and the founding chair of the Smith computer science department. His main research interest is computational geometry.
The napkin folding problem is a problem in geometry and the mathematics of paper folding that explores whether folding a square or a rectangular napkin can increase its perimeter. The problem is known under several names, including the Margulis napkin problem, suggesting it is due to Grigory Margulis, and the Arnold's rouble problem referring to Vladimir Arnold and the folding of a Russian ruble bank note. Some versions of the problem were solved by Robert J. Lang, Svetlana Krat, Alexey S. Tarasov, and Ivan Yaschenko. One form of the problem remains open.
The Alexandrov uniqueness theorem is a rigidity theorem in mathematics, describing three-dimensional convex polyhedra in terms of the distances between points on their surfaces. It implies that convex polyhedra with distinct shapes from each other also have distinct metric spaces of surface distances, and it characterizes the metric spaces that come from the surface distances on polyhedra. It is named after Soviet mathematician Aleksandr Danilovich Aleksandrov, who published it in the 1940s.

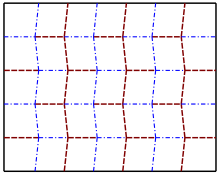
In mechanical engineering, Yoshimura buckling is a triangular mesh buckling pattern found in thin-walled cylinders under compression along the axis of the cylinder, producing a corrugated shape resembling the Schwarz lantern. The same pattern can be seen on the sleeves of Mona Lisa.
In differential geometry the theorem of the three geodesics, also known as Lyusternik–Schnirelmann theorem, states that every Riemannian manifold with the topology of a sphere has at least three simple closed geodesics. The result can also be extended to quasigeodesics on a convex polyhedron, and to closed geodesics of reversible Finsler 2-spheres. The theorem is sharp: although every Riemannian 2-sphere contains infinitely many distinct closed geodesics, only three of them are guaranteed to have no self-intersections. For example, by a result of Morse if the lengths of three principal axes of an ellipsoid are distinct, but sufficiently close to each other, then the ellipsoid has only three simple closed geodesics.
Geometric Folding Algorithms: Linkages, Origami, Polyhedra is a monograph on the mathematics and computational geometry of mechanical linkages, paper folding, and polyhedral nets, by Erik Demaine and Joseph O'Rourke. It was published in 2007 by Cambridge University Press (ISBN 978-0-521-85757-4). A Japanese-language translation by Ryuhei Uehara was published in 2009 by the Modern Science Company (ISBN 978-4-7649-0377-7).
In computational geometry, the star unfolding of a convex polyhedron is a net obtained by cutting the polyhedron along geodesics through its faces. It has also been called the inward layout of the polyhedron, or the Alexandrov unfolding after Aleksandr Danilovich Aleksandrov, who first considered it.
In the mathematics of paper folding, the big-little-big lemma is a necessary condition for a crease pattern with specified mountain folds and valley folds to be able to be folded flat. It differs from Kawasaki's theorem, which characterizes the flat-foldable crease patterns in which a mountain-valley assignment has not yet been made. Together with Maekawa's theorem on the total number of folds of each type, the big-little-big lemma is one of the two main conditions used to characterize the flat-foldability of mountain-valley assignments for crease patterns that meet the conditions of Kawasaki's theorem. Mathematical origami expert Tom Hull calls the big-little-big lemma "one of the most basic rules" for flat foldability of crease patterns.
Origami Polyhedra Design is a book on origami designs for constructing polyhedra. It was written by origami artist and mathematician John Montroll, and published in 2009 by A K Peters.
Tomohiro Tachi is a Japanese academic who studies origami from an interdisciplinary perspective, combining approaches from the mathematics of paper folding, structural rigidity, computational geometry, architecture, and materials science. His work was profiled in "The Origami Revolution" (2017), part of the Nova series of US science documentaries. He is a professor at the University of Tokyo.

In the geometry of convex polyhedra, blooming or continuous blooming is a continuous three-dimensional motion of the surface of the polyhedron, cut to form a polyhedral net, from the polyhedron into a flat and non-self-overlapping placement of the net in a plane. As in rigid origami, the polygons of the net must remain individually flat throughout the motion, and are not allowed to intersect or cross through each other. A blooming, reversed to go from the flat net to a polyhedron, can be thought of intuitively as a way to fold the polyhedron from a paper net without bending the paper except at its designated creases.
In computational geometry, the source unfolding of a convex polyhedron is a net obtained by cutting the polyhedron along the cut locus of a point on the surface of the polyhedron. The cut locus of a point consists of all points on the surface that have two or more shortest geodesics to . For every convex polyhedron, and every choice of the point on its surface, cutting the polyhedron on the cut locus will produce a result that can be unfolded into a flat plane, producing the source unfolding. The resulting net may, however, cut across some of the faces of the polyhedron rather than only cutting along its edges.