Geometric Exercises in Paper Folding

Last updated
Geometric Exercises in Paper Folding
Geometrical Exercises in Paper Folding title page.png
Title page of the first edition
AuthorT. Sundara Row
LanguageEnglish
PublisherAddison & Co.
Publication date
1893
Publication placeIndia
Media typePrint
Pages114

Geometric Exercises in Paper Folding is a book on the mathematics of paper folding. It was written by Indian mathematician T. Sundara Row, first published in India in 1893, and later republished in many other editions. Its topics include paper constructions for regular polygons, symmetry, and algebraic curves. According to the historian of mathematics Michael Friedman, it became "one of the main engines of the popularization of folding as a mathematical activity". [1]

Contents

Publication history

Geometric Exercises in Paper Folding was first published by Addison & Co. in Madras in 1893. [2] [3] The book became known in Europe through a remark of Felix Klein in his book Vorträge über ausgewählte Fragen der Elementargeometrie (1895) and its translation Famous Problems Of Elementary Geometry (1897). [4] [1] Based on the success of Geometric Exercises in Paper Folding in Germany, [5] the Open Court Press of Chicago published it in the US, with updates by Wooster Woodruff Beman and David Eugene Smith. Although Open Court listed four editions of the book, published in 1901, 1905, 1917, and 1941, [3] the content did not change between these editions. [1] The fourth edition was also published in London by La Salle, and both presses reprinted the fourth edition in 1958. [3]

The contributions of Beman and Smith to the Open Court editions have been described as "translation and adaptation", despite the fact that the original 1893 edition was already in English. [5] Beman and Smith also replaced many footnotes with references to their own work, [1] [6] replaced some of the diagrams by photographs, [4] [7] and removed some remarks specific to India. [1] In 1966, Dover Publications of New York published a reprint of the 1905 edition, and other publishers of out-of-copyright works have also printed editions of the book. [3]

Topics

Geometric Exercises in Paper Folding shows how to construct various geometric figures using paper-folding in place of the classical Greek Straightedge and compass constructions. [6]

The book begins by constructing regular polygons beyond the classical constructible polygons of 3, 4, or 5 sides, or of any power of two times these numbers, and the construction by Carl Friedrich Gauss of the heptadecagon, it also provides a paper-folding construction of the regular nonagon, not possible with compass and straightedge. [6] The nonagon construction involves angle trisection, but Rao is vague about how this can be performed using folding; an exact and rigorous method for folding-based trisection would have to wait until the work in the 1930s of Margherita Piazzola Beloch. [1] The construction of the square also includes a discussion of the Pythagorean theorem. [6] The book uses high-order regular polygons to provide a geometric calculation of pi. [7] [6]

A discussion of the symmetries of the plane includes congruence, similarity, [7] and collineations of the projective plane; this part of the book also covers some of the major theorems of projective geometry including Desargues's theorem, Pascal's theorem, and Poncelet's closure theorem. [6]

Later chapters of the book show how to construct algebraic curves including the conic sections, the conchoid, the cubical parabola, the witch of Agnesi, [7] the cissoid of Diocles, [8] and the Cassini ovals. [1] The book also provides a gnomon-based proof of Nicomachus's theorem that the sum of the first cubes is the square of the sum of the first integers, [4] and material on other arithmetic series, geometric series, and harmonic series. [6]

There are 285 exercises, and many illustrations, both in the form of diagrams and (in the updated editions) photographs. [4] [7]

Influences

Tandalam Sundara Row was born in 1853, the son of a college principal, and earned a bachelor's degree at the Kumbakonam College in 1874, with second-place honours in mathematics. He became a tax collector in Tiruchirappalli, retiring in 1913, and pursued mathematics as an amateur. As well as Geometric Exercises in Paper Folding, he also wrote a second book, Elementary Solid Geometry, published in three parts from 1906 to 1909. [1]

One of the sources of inspiration for Geometric Exercises in Paper Folding was Kindergarten Gift No. VIII: Paper-folding. This was one of the Froebel gifts, a set of kindergarten activities designed in the early 19th century by Friedrich Fröbel. [2] [9] The book was also influenced by an earlier Indian geometry textbook, First Lessons in Geometry, by Bhimanakunte Hanumantha Rao (1855–1922). First Lessons drew inspiration from Fröbel's gifts in setting exercises based on paper-folding, and from the book Elementary Geometry: Congruent Figures by Olaus Henrici in using a definition of geometric congruence based on matching shapes to each other and well-suited for folding-based geometry. [1]

In turn, Geometric Exercises in Paper Folding inspired other works of mathematics. A chapter in Mathematische Unterhaltungen und Spiele [Mathematical Recreations and Games] by Wilhelm Ahrens (1901) concerns folding and is based on Rao's book, inspiring the inclusion of this material in several other books on recreational mathematics. Other mathematical publications have studied the curves that can be generated by the folding processes used in Geometric Exercises in Paper Folding. [10] In 1934, Margherita Piazzola Beloch began her research on axiomatizing the mathematics of paper-folding, a line of work that would eventually lead to the Huzita–Hatori axioms in the late 20th century. Beloch was explicitly inspired by Rao's book, titling her first work in this area "Alcune applicazioni del metodo del ripiegamento della carta di Sundara Row" ["Several applications of the method of folding a paper of Sundara Row"]. [11]

Audience and reception

The original intent of Geometric Exercises in Paper Folding was twofold: as an aid in geometry instruction, and as a work of recreational mathematics to inspire interest in geometry in a general audience. [2] Edward Mann Langley, reviewing the 1901 edition, suggested that its content went well beyond what should be covered in a standard geometry course. [4] And in their own textbook on geometry using paper-folding exercises, The First Book of Geometry (1905), Grace Chisholm Young and William Henry Young heavily criticized Geometric Exercises in Paper Folding, writing that it is "too difficult for a child, and too infantile for a grown person". [10] However, reviewing the 1966 Dover edition, mathematics educator Pamela Liebeck called it "remarkably relevant" to the discovery learning techniques for geometry instruction of the time, [7] and in 2016 computational origami expert Tetsuo Ida, introducing an attempt to formalize the mathematics of the book, wrote "After 123 years, the significance of the book remains." [9]

Related Research Articles

<span class="mw-page-title-main">Constructible number</span> Number constructible via compass and straightedge

In geometry and algebra, a real number is constructible if and only if, given a line segment of unit length, a line segment of length can be constructed with compass and straightedge in a finite number of steps. Equivalently, is constructible if and only if there is a closed-form expression for using only integers and the operations for addition, subtraction, multiplication, division, and square roots.

<span class="mw-page-title-main">Straightedge and compass construction</span> Method of drawing geometric objects

In geometry, straightedge-and-compass construction – also known as ruler-and-compass construction, Euclidean construction, or classical construction – is the construction of lengths, angles, and other geometric figures using only an idealized ruler and a pair of compasses.

<span class="mw-page-title-main">Angle trisection</span> Construction of an angle equal to one third a given angle

Angle trisection is a classical problem of straightedge and compass construction of ancient Greek mathematics. It concerns construction of an angle equal to one third of a given arbitrary angle, using only two tools: an unmarked straightedge and a compass.

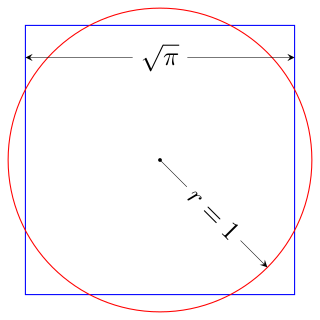
<span class="mw-page-title-main">Squaring the circle</span> Problem of constructing equal-area shapes

Squaring the circle is a problem in geometry first proposed in Greek mathematics. It is the challenge of constructing a square with the area of a given circle by using only a finite number of steps with a compass and straightedge. The difficulty of the problem raised the question of whether specified axioms of Euclidean geometry concerning the existence of lines and circles implied the existence of such a square.

<span class="mw-page-title-main">Mathematics of paper folding</span> Overview of the mathematics of paper folding

The discipline of origami or paper folding has received a considerable amount of mathematical study. Fields of interest include a given paper model's flat-foldability, and the use of paper folds to solve mathematical equations up to the third order.

The Huzita–Justin axioms or Huzita–Hatori axioms are a set of rules related to the mathematical principles of origami, describing the operations that can be made when folding a piece of paper. The axioms assume that the operations are completed on a plane, and that all folds are linear. These are not a minimal set of axioms but rather the complete set of possible single folds.

<span class="mw-page-title-main">Tomahawk (geometry)</span> Tool for trisecting angles

The tomahawk is a tool in geometry for angle trisection, the problem of splitting an angle into three equal parts. The boundaries of its shape include a semicircle and two line segments, arranged in a way that resembles a tomahawk, a Native American axe. The same tool has also been called the shoemaker's knife, but that name is more commonly used in geometry to refer to a different shape, the arbelos.

In number theory, a Pierpont prime is a prime number of the form for some nonnegative integers u and v. That is, they are the prime numbers p for which p − 1 is 3-smooth. They are named after the mathematician James Pierpont, who used them to characterize the regular polygons that can be constructed using conic sections. The same characterization applies to polygons that can be constructed using ruler, compass, and angle trisector, or using paper folding.

Pierre Laurent Wantzel was a French mathematician who proved that several ancient geometric problems were impossible to solve using only compass and straightedge.

<span class="mw-page-title-main">David Eugene Smith</span> American mathematician

David Eugene Smith was an American mathematician, educator, and editor.

Geometry is a branch of mathematics concerned with questions of shape, size, relative position of figures, and the properties of space. Geometry is one of the oldest mathematical sciences.

<span class="mw-page-title-main">Octadecagon</span> Polygon with 18 edges

In geometry, an octadecagon or 18-gon is an eighteen-sided polygon.

<i>Girih</i> Geometric patterns in Islamic architecture

Girih are decorative Islamic geometric patterns used in architecture and handicraft objects, consisting of angled lines that form an interlaced strapwork pattern.

<span class="mw-page-title-main">Square trisection</span> Cutting a square into pieces which rearrange into 3 identical squares

In geometry, a square trisection is a type of dissection problem which consists of cutting a square into pieces that can be rearranged to form three identical squares.

<span class="mw-page-title-main">Lill's method</span> Graphical method for the real roots of a polynomial

In mathematics, Lill's method is a visual method of finding the real roots of a univariate polynomial of any degree. It was developed by Austrian engineer Eduard Lill in 1867. A later paper by Lill dealt with the problem of complex roots.

Geometric Folding Algorithms: Linkages, Origami, Polyhedra is a monograph on the mathematics and computational geometry of mechanical linkages, paper folding, and polyhedral nets, by Erik Demaine and Joseph O'Rourke. It was published in 2007 by Cambridge University Press (ISBN 978-0-521-85757-4). A Japanese-language translation by Ryuhei Uehara was published in 2009 by the Modern Science Company (ISBN 978-4-7649-0377-7).

<i>A History of Folding in Mathematics</i>

A History of Folding in Mathematics: Mathematizing the Margins is a book in the history of mathematics on the mathematics of paper folding. It was written by Michael Friedman and published in 2018 by Birkhäuser as volume 59 of their Historical Studies series.

<i>The Ancient Tradition of Geometric Problems</i> 1986 book on ancient Greek mathematics by Wilbur Knorr

The Ancient Tradition of Geometric Problems is a book on ancient Greek mathematics, focusing on three problems now known to be impossible if one uses only the straightedge and compass constructions favored by the Greek mathematicians: squaring the circle, doubling the cube, and trisecting the angle. It was written by Wilbur Knorr (1945–1997), a historian of mathematics, and published in 1986 by Birkhäuser. Dover Publications reprinted it in 1993.

Geometric Constructions is a mathematics textbook on constructible numbers, and more generally on using abstract algebra to model the sets of points that can be created through certain types of geometric construction, and using Galois theory to prove limits on the constructions that can be performed. It was written by George E. Martin, and published by Springer-Verlag in 1998 as volume 81 of their Undergraduate Texts in Mathematics book series.

Geometric Origami is a book on the mathematics of paper folding, focusing on the ability to simulate and extend classical straightedge and compass constructions using origami. It was written by Austrian mathematician Robert Geretschläger and published by Arbelos Publishing in 2008. The Basic Library List Committee of the Mathematical Association of America has suggested its inclusion in undergraduate mathematics libraries.

References

  1. 1 2 3 4 5 6 7 8 9 Friedman, Michael (2018), "4.2.2.2 The Books of Tandalam Sundara Row", A History of Folding in Mathematics: Mathematizing the Margins, Birkhäuser, pp. 254–268, doi:10.1007/978-3-319-72487-4_4, ISBN   978-3-319-72486-7
  2. 1 2 3 "Books and magazines received", The Mathematical Gazette (3): 24, December 1894, JSTOR   3603999 ; includes review of Geometric Exercises in Paper Folding, Madras edition
  3. 1 2 3 4 Geometric Exercises in Paper Folding (editions), Worldcat, retrieved 2020-04-12
  4. 1 2 3 4 5 Langley, E. M. (October 1902), "Review of Geometric Exercises in Paper Folding (1st Open Court edition)", The Mathematical Gazette , 2 (35): 209, doi:10.2307/3604241, JSTOR   3604241, S2CID   186387817
  5. 1 2 "Review of Geometric Exercises in Paper Folding (1st Open Court edition)", Journal of Education , 54 (22), December 1901, JSTOR   44054257
  6. 1 2 3 4 5 6 7 Willson, F. N. (March 21, 1902), "Review of Geometric Exercises in Paper Folding (1st Open Court edition)", Science , New Series, 15 (377): 464–465, doi:10.1126/science.15.377.464, JSTOR   1629651
  7. 1 2 3 4 5 6 Liebeck, Pamela (February 1968), "Review of Geometric Exercises in Paper Folding (Dover edition)", The Mathematical Gazette , 52 (379): 75–76, doi:10.1017/s0025557200120716, S2CID   185077704
  8. ↑ Klein (1897), as quoted by the Journal of Education
  9. 1 2 Ida, Tetsuo (September 2016), "Revisit of Geometric Exercise in Paper Folding from a viewpoint of computational origami", Proceedings of the 18th International Symposium on Symbolic and Numeric Algorithms for Scientific Computing (SYNASC), IEEE, doi:10.1109/synasc.2016.017, S2CID   17423586
  10. 1 2 Friedman (2018), Section 5.1 The Influence of Row's Book, pp. 272–318
  11. ↑ Friedman (2018), p. 323.
This page is based on this Wikipedia article
Text is available under the CC BY-SA 4.0 license; additional terms may apply.
Images, videos and audio are available under their respective licenses.