In geometry, a dodecahedron or duodecahedron is any polyhedron with twelve flat faces. The most familiar dodecahedron is the regular dodecahedron with regular pentagons as faces, which is a Platonic solid. There are also three regular star dodecahedra, which are constructed as stellations of the convex form. All of these have icosahedral symmetry, order 120.

In geometry, a regular icosahedron is a convex polyhedron with 20 faces, 30 edges and 12 vertices. It is one of the five Platonic solids, and the one with the most faces.

In geometry, an octahedron is a polyhedron with eight faces. The term is most commonly used to refer to the regular octahedron, a Platonic solid composed of eight equilateral triangles, four of which meet at each vertex.

In geometry, the triangular bipyramid is a type of hexahedron, being the first in the infinite set of face-transitive bipyramids. It is the dual of the triangular prism with 6 isosceles triangle faces.
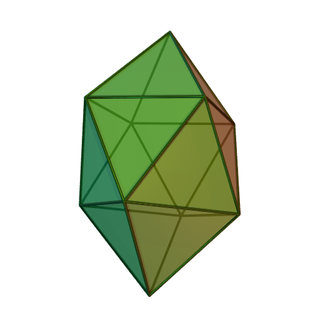
In geometry, the gyroelongated square bipyramid, heccaidecadeltahedron, or tetrakis square antiprism is one of the Johnson solids. As the name suggests, it can be constructed by gyroelongating an octahedron by inserting a square antiprism between its congruent halves. It is one of the eight strictly-convex deltahedra.
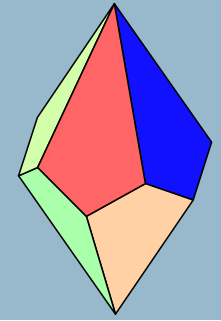
In geometry, an n-gonaltrapezohedron, antidipyramid, antibipyramid, or deltohedron is the dual polyhedron of an n-gonal antiprism. The 2n faces of an n-trapezohedron are congruent and symmetrically staggered; they are called twisted kites. With a higher symmetry, its 2n faces are kites.

In geometry, the pentagonal bipyramid is third of the infinite set of face-transitive bipyramids, and the 13th Johnson solid. Each bipyramid is the dual of a uniform prism.

A hexagonal bipyramid is a polyhedron formed from two hexagonal pyramids joined at their bases. The resulting solid has 12 triangular faces, 8 vertices and 18 edges. The 12 faces are identical isosceles triangles.
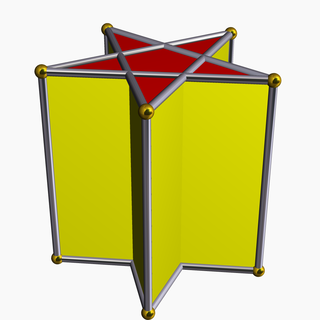
In geometry, the pentagrammic prism is one of an infinite set of nonconvex prisms formed by square sides and two regular star polygon caps, in this case two pentagrams.

In geometry, a pentagonal trapezohedron or deltohedron is the third in an infinite series of face-transitive polyhedra which are dual polyhedra to the antiprisms. It has ten faces which are congruent kites.

In geometry, an n-gonaltruncated trapezohedron is a polyhedron formed by a n-gonal trapezohedron with n-gonal pyramids truncated from its two polar axis vertices. If the polar vertices are completely truncated (diminished), a trapezohedron becomes an antiprism.

In geometry, Conway polyhedron notation, invented by John Horton Conway and promoted by George W. Hart, is used to describe polyhedra based on a seed polyhedron modified by various prefix operations.
In geometry, a simplicial polytope is a polytope whose facets are all simplices. For example, a simplicial polyhedron in three dimensions contains only triangular faces and corresponds via Steinitz's theorem to a maximal planar graph.
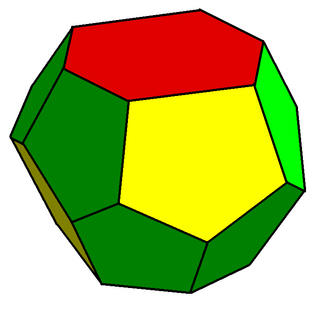
A tetradecahedron is a polyhedron with 14 faces. There are numerous topologically distinct forms of a tetradecahedron, with many constructible entirely with regular polygon faces.

In geometry, an enneahedron is a polyhedron with nine faces. There are 2606 types of convex enneahedron, each having a different pattern of vertex, edge, and face connections. None of them are regular.

In geometry, a diminished trapezohedron is a polyhedron in an infinite set of polyhedra, constructed by removing one of the polar vertices of a trapezohedron and replacing it by a new face (diminishment). It has one regular n-gonal base face, n triangle faces around the base, and n kites meeting on top. The kites can also be replaced by rhombi with specific proportions.
A hexadecahedron is a polyhedron with 16 faces. No hexadecahedron is regular; hence, the name is ambiguous. There are numerous topologically distinct forms of a hexadecahedron, for example the pentadecagonal pyramid, tetradecagonal prism and heptagonal antiprism.

In geometry, an icosahedron is a polyhedron with 20 faces. The name comes from Ancient Greek εἴκοσι (eíkosi) 'twenty' and from Ancient Greek ἕδρα (hédra) ' seat'. The plural can be either "icosahedra" or "icosahedrons".