In geometry, an n-gonal antiprism or n-antiprism is a polyhedron composed of two parallel direct copies of an n-sided polygon, connected by an alternating band of 2n triangles. They are represented by the Conway notation An.
In geometry, a bipyramid, dipyramid, or double pyramid is a polyhedron formed by fusing two pyramids together base-to-base. The polygonal base of each pyramid must therefore be the same, and unless otherwise specified the base vertices are usually coplanar and a bipyramid is usually symmetric, meaning the two pyramids are mirror images across their common base plane. When each apex of the bipyramid is on a line perpendicular to the base and passing through its center, it is a right bipyramid; otherwise it is oblique. When the base is a regular polygon, the bipyramid is also called regular.
In geometry, a dodecahedron or duodecahedron is any polyhedron with twelve flat faces. The most familiar dodecahedron is the regular dodecahedron with regular pentagons as faces, which is a Platonic solid. There are also three regular star dodecahedra, which are constructed as stellations of the convex form. All of these have icosahedral symmetry, order 120.

In Euclidean geometry, a kite is a quadrilateral with reflection symmetry across a diagonal. Because of this symmetry, a kite has two equal angles and two pairs of adjacent equal-length sides. Kites are also known as deltoids, but the word deltoid may also refer to a deltoid curve, an unrelated geometric object sometimes studied in connection with quadrilaterals. A kite may also be called a dart, particularly if it is not convex.

In geometry, a prism is a polyhedron comprising an n-sided polygon base, a second base which is a translated copy of the first, and n other faces, necessarily all parallelograms, joining corresponding sides of the two bases. All cross-sections parallel to the bases are translations of the bases. Prisms are named after their bases, e.g. a prism with a pentagonal base is called a pentagonal prism. Prisms are a subclass of prismatoids.

In geometry, the rhombic dodecahedron is a convex polyhedron with 12 congruent rhombic faces. It has 24 edges, and 14 vertices of 2 types. It is a Catalan solid, and the dual polyhedron of the cuboctahedron.

In geometry, a deltoidal hexecontahedron is a Catalan solid which is the dual polyhedron of the rhombicosidodecahedron, an Archimedean solid. It is one of six Catalan solids to not have a Hamiltonian path among its vertices.
In geometry, the term semiregular polyhedron is used variously by different authors.
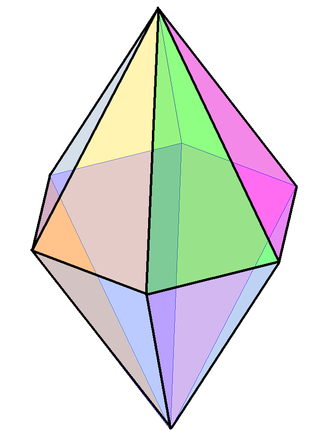
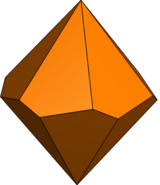
A hexagonal bipyramid is a polyhedron formed from two hexagonal pyramids joined at their bases. The resulting solid has 12 triangular faces, 8 vertices and 18 edges. The 12 faces are identical isosceles triangles.

In geometry of 4 dimensions or higher, a double prism or duoprism is a polytope resulting from the Cartesian product of two polytopes, each of two dimensions or higher. The Cartesian product of an n-polytope and an m-polytope is an (n+m)-polytope, where n and m are dimensions of 2 (polygon) or higher.

In geometry, a trigonal trapezohedron is a polyhedron with six congruent quadrilateral faces, which may be scalene or rhomboid. The variety with rhombus-shaped faces faces is a rhombohedron. An alternative name for the same shape is the trigonal deltohedron.
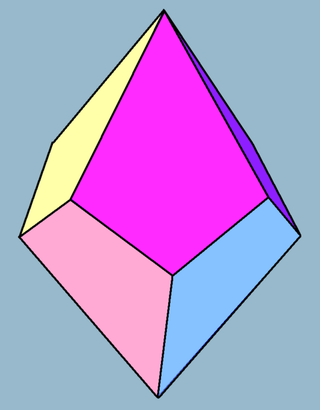
In geometry, a tetragonal trapezohedron, or deltohedron, is the second in an infinite series of trapezohedra, which are dual to the antiprisms. It has eight faces, which are congruent kites, and is dual to the square antiprism.

In geometry, a hexagonal trapezohedron or deltohedron is the fourth in an infinite series of trapezohedra which are dual polyhedra to the antiprisms. It has twelve faces which are congruent kites. It can be described by the Conway notation dA6.

In geometry, an n-gonaltruncated trapezohedron is a polyhedron formed by a n-gonal trapezohedron with n-gonal pyramids truncated from its two polar axis vertices.

In geometry, a tessellation of dimension 2 or higher, or a polytope of dimension 3 or higher, is isohedral or face-transitive if all its faces are the same. More specifically, all faces must be not merely congruent but must be transitive, i.e. must lie within the same symmetry orbit. In other words, for any two faces A and B, there must be a symmetry of the entire figure by translations, rotations, and/or reflections that maps A onto B. For this reason, convex isohedral polyhedra are the shapes that will make fair dice.

In geometry, a compound of two tetrahedra is constructed by two overlapping tetrahedra, usually implied as regular tetrahedra.

In geometry, a prismatic compound of antiprism is a category of uniform polyhedron compound. Each member of this infinite family of uniform polyhedron compounds is a symmetric arrangement of antiprisms sharing a common axis of rotational symmetry.

In geometry, a diminished trapezohedron is a polyhedron in an infinite set of polyhedra, constructed by removing one of the polar vertices of a trapezohedron and replacing it by a new face (diminishment). It has one regular n-gonal base face, n triangle faces around the base, and n kites meeting on top. The kites can also be replaced by rhombi with specific proportions.

In geometry, chamfering or edge-truncation is a topological operator that modifies one polyhedron into another. It is similar to expansion: it moves the faces apart (outward), and adds a new face between each two adjacent faces; but contrary to expansion, it maintains the original vertices. For a polyhedron, this operation adds a new hexagonal face in place of each original edge.