In geometry, a dodecahedron or duodecahedron is any polyhedron with twelve flat faces. The most familiar dodecahedron is the regular dodecahedron with regular pentagons as faces, which is a Platonic solid. There are also three regular star dodecahedra, which are constructed as stellations of the convex form. All of these have icosahedral symmetry, order 120.
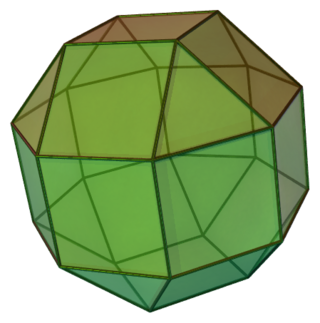
In geometry, a Johnson solid is a strictly convex polyhedron each face of which is a regular polygon. There is no requirement that each face must be the same polygon, or that the same polygons join around each vertex. An example of a Johnson solid is the square-based pyramid with equilateral sides ; it has 1 square face and 4 triangular faces. Some authors require that the solid not be uniform before they refer to it as a "Johnson solid".

In geometry, the elongated pentagonal rotunda is one of the Johnson solids (J21). As the name suggests, it can be constructed by elongating a pentagonal rotunda (J6) by attaching a decagonal prism to its base. It can also be seen as an elongated pentagonal orthobirotunda (J42) with one pentagonal rotunda removed.

In geometry, the gyroelongated pentagonal rotunda is one of the Johnson solids (J25). As the name suggests, it can be constructed by gyroelongating a pentagonal rotunda (J6) by attaching a decagonal antiprism to its base. It can also be seen as a gyroelongated pentagonal birotunda (J48) with one pentagonal rotunda removed.
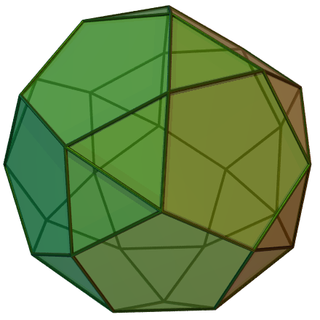
In geometry, the pentagonal orthobirotunda is one of the Johnson solids. It can be constructed by joining two pentagonal rotundae along their decagonal faces, matching like faces.

In geometry, the elongated pentagonal gyrobirotunda is one of the Johnson solids. As the name suggests, it can be constructed by elongating a "pentagonal gyrobirotunda," or icosidodecahedron, by inserting a decagonal prism between its congruent halves. Rotating one of the pentagonal rotundae through 36 degrees before inserting the prism yields an elongated pentagonal orthobirotunda.

In geometry, the triangular hebesphenorotunda is one of the Johnson solids.

In geometry, the elongated pentagonal cupola is one of the Johnson solids. As the name suggests, it can be constructed by elongating a pentagonal cupola by attaching a decagonal prism to its base. The solid can also be seen as an elongated pentagonal orthobicupola with its "lid" removed.

In geometry, the gyrobifastigium is the 26th Johnson solid. It can be constructed by joining two face-regular triangular prisms along corresponding square faces, giving a quarter-turn to one prism. It is the only Johnson solid that can tile three-dimensional space.

In geometry, the elongated pentagonal gyrocupolarotunda is one of the Johnson solids. As the name suggests, it can be constructed by elongating a pentagonal gyrocupolarotunda by inserting a decagonal prism between its halves. Rotating either the pentagonal cupola or the pentagonal rotunda through 36 degrees before inserting the prism yields an elongated pentagonal orthocupolarotunda.
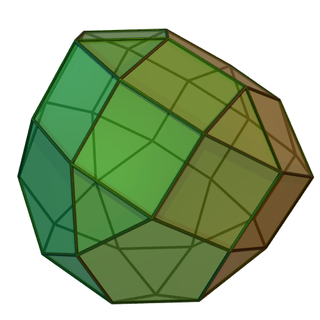
In geometry, the elongated pentagonal orthocupolarotunda is one of the Johnson solids. As the name suggests, it can be constructed by elongating a pentagonal orthocupolarotunda by inserting a decagonal prism between its halves. Rotating either the cupola or the rotunda through 36 degrees before inserting the prism yields an elongated pentagonal gyrocupolarotunda.
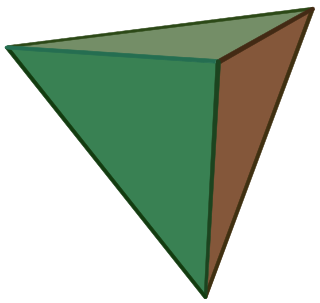
In geometry, a uniform polyhedron has regular polygons as faces and is vertex-transitive. It follows that all vertices are congruent.

In geometry, a bicupola is a solid formed by connecting two cupolae on their bases.

In geometry, a rotunda is any member of a family of dihedral-symmetric polyhedra. They are similar to a cupola but instead of alternating squares and triangles, it alternates pentagons and triangles around an axis. The pentagonal rotunda is a Johnson solid.

In geometry, the elongated pyramids are an infinite set of polyhedra, constructed by adjoining an n-gonal pyramid to an n-gonal prism. Along with the set of pyramids, these figures are topologically self-dual.

In geometry, the gyroelongated pyramids are an infinite set of polyhedra, constructed by adjoining an n-gonal pyramid to an n-gonal antiprism.

In geometry, the elongated cupolae are an infinite set of polyhedra, constructed by adjoining an n-gonal cupola to an 2n-gonal prism.

In geometry, the gyroelongated cupolae are an infinite set of polyhedra, constructed by adjoining an n-gonal cupola to an 2n-gonal antiprism.

In geometry, an icosahedron is a polyhedron with 20 faces. The name comes from Ancient Greek εἴκοσι (eíkosi) 'twenty', and ἕδρα (hédra) 'seat'. The plural can be either "icosahedra" or "icosahedrons".



























