Related Research Articles
In the mathematical field of geometric topology, the Poincaré conjecture is a theorem about the characterization of the 3-sphere, which is the hypersphere that bounds the unit ball in four-dimensional space.
In mathematics, Floer homology is a tool for studying symplectic geometry and low-dimensional topology. Floer homology is a novel invariant that arises as an infinite-dimensional analogue of finite-dimensional Morse homology. Andreas Floer introduced the first version of Floer homology, now called Lagrangian Floer homology, in his proof of the Arnold conjecture in symplectic geometry. Floer also developed a closely related theory for Lagrangian submanifolds of a symplectic manifold. A third construction, also due to Floer, associates homology groups to closed three-dimensional manifolds using the Yang–Mills functional. These constructions and their descendants play a fundamental role in current investigations into the topology of symplectic and contact manifolds as well as (smooth) three- and four-dimensional manifolds.
In topology, an area of mathematics, the virtually Haken conjecture states that every compact, orientable, irreducible three-dimensional manifold with infinite fundamental group is virtually Haken. That is, it has a finite cover that is a Haken manifold.
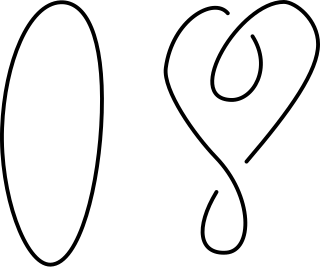
In mathematics, the unknotting problem is the problem of algorithmically recognizing the unknot, given some representation of a knot, e.g., a knot diagram. There are several types of unknotting algorithms. A major unresolved challenge is to determine if the problem admits a polynomial time algorithm; that is, whether the problem lies in the complexity class P.
Peter Steven Ozsváth is a professor of mathematics at Princeton University. He created, along with Zoltán Szabó, Heegaard Floer homology, a homology theory for 3-manifolds.
Clifford Henry Taubes is the William Petschek Professor of Mathematics at Harvard University and works in gauge field theory, differential geometry, and low-dimensional topology. His brother is the journalist Gary Taubes.
The Oswald Veblen Prize in Geometry is an award granted by the American Mathematical Society for notable research in geometry or topology. It was founded in 1961 in memory of Oswald Veblen. The Veblen Prize is now worth US$5000, and is awarded every three years.
Zoltán Szabó is a professor of mathematics at Princeton University known for his work on Heegaard Floer homology.
Leon Melvyn Simon, born in 1945, is a Leroy P. Steele Prize and Bôcher Prize-winning mathematician, known for deep contributions to the fields of geometric analysis, geometric measure theory, and partial differential equations. He is currently Professor Emeritus in the Mathematics Department at Stanford University.
Tomasz Mrowka is an American mathematician specializing in differential geometry and gauge theory. He is the Singer Professor of Mathematics and former head of the Department of Mathematics at the Massachusetts Institute of Technology.
Tobias Holck Colding is a Danish mathematician working on geometric analysis, and low-dimensional topology. He is the great grandchild of Ludwig August Colding.
In hyperbolic geometry, the ending lamination theorem, originally conjectured by William Thurston (1982), states that hyperbolic 3-manifolds with finitely generated fundamental groups are determined by their topology together with certain "end invariants", which are geodesic laminations on some surfaces in the boundary of the manifold.
Lawrence David Guth is a professor of mathematics at the Massachusetts Institute of Technology.
In mathematics, the Almgren–Pitts min-max theory is an analogue of Morse theory for hypersurfaces.
Jeremy Adam Kahn is an American mathematician. He works on hyperbolic geometry, Riemann surfaces and complex dynamics.
In mathematics, the curve complex is a simplicial complex C(S) associated to a finite-type surface S, which encodes the combinatorics of simple closed curves on S. The curve complex turned out to be a fundamental tool in the study of the geometry of the Teichmüller space, of mapping class groups and of Kleinian groups. It was introduced by W.J.Harvey in 1978.
Eleny-Nicoleta Ionel is a Romanian mathematician whose research concerns symplectic geometry, including the study of the Gromov–Witten invariants and Gopakumar–Vafa invariant. Among her most significant results are the construction of relative Gromov-Witten invariants of symplectic manifolds, and the proof of the vanishing in codimension at least g of the tautological ring of the moduli space of genus-g curves.
William Hamilton Meeks III is an American mathematician, specializing in differential geometry and minimal surfaces.
Albert Marden is an American mathematician, specializing in complex analysis and hyperbolic geometry.
