| 1983 European Athletics Indoor Championships | ||
|---|---|---|
 | ||
| Track events | ||
| 60 m | men | women |
| 200 m | men | women |
| 400 m | men | women |
| 800 m | men | women |
| 1500 m | men | women |
| 3000 m | men | women |
| 60 m hurdles | men | women |
| 5000 m walk | men | |
| Field events | ||
| High jump | men | women |
| Pole vault | men | |
| Long jump | men | women |
| Triple jump | men | |
| Shot put | men | women |
The women's 800 metres event at the 1983 European Athletics Indoor Championships was held on 5 and 6 March. [1]
| Gold | Silver | Bronze |
Svetlana Kitova  Soviet Union | Zuzana Moravčíková  Czechoslovakia | Olga Simakova Soviet Union |
First 2 from each heat (Q) and the next 2 fastest (q) qualified for the final.
| Rank | Heat | Name | Nationality | Time | Notes |
|---|---|---|---|---|---|
| 1 | 1 | Svetlana Kitova | Soviet Union | 2:02.93 | Q |
| 2 | 1 | Ines Vogelgesang |  East Germany | 2:03.25 | Q |
| 3 | 2 | Olga Simanova | Soviet Union | 2:03.31 | Q |
| 4 | 2 | Jane Finch |  Great Britain | 2:03.32 | Q |
| 5 | 2 | Zuzana Moravčíková | Czechoslovakia | 2:03.33 | q |
| 6 | 2 | Doina Melinte |  Romania | 2:03.33 | q |
| 7 | 1 | Teena Colebrook | Great Britain | 2:03.89 |
| Rank | Name | Nationality | Time | Notes |
|---|---|---|---|---|
 | Svetlana Kitova | Soviet Union | 2:01.28 | |
 | Zuzana Moravčíková | Czechoslovakia | 2:01.66 | |
 | Olga Simanova | Soviet Union | 2:02.25 | |
| 4 | Doina Melinte | Romania | 2:02.70 | |
| 5 | Ines Vogelgesang | East Germany | 2:03.11 | |
| 6 | Jane Finch | Great Britain | 2:03.21 |
In mathematics, a finite field or Galois field is a field that contains a finite number of elements. As with any field, a finite field is a set on which the operations of multiplication, addition, subtraction and division are defined and satisfy certain basic rules. The most common examples of finite fields are given by the integers mod p when p is a prime number.
Propositional calculus is a branch of logic. It is also called propositional logic, statement logic, sentential calculus, sentential logic, or sometimes zeroth-order logic. It deals with propositions and relations between propositions, including the construction of arguments based on them. Compound propositions are formed by connecting propositions by logical connectives. Propositions that contain no logical connectives are called atomic propositions.
Q, or q, is the seventeenth letter of the Latin alphabet, used in the modern English alphabet, the alphabets of other western European languages and others worldwide. Its name in English is pronounced, most commonly spelled cue, but also kew, kue and que.
In statistics, the standard deviation is a measure of the amount of variation or dispersion of a set of values. A low standard deviation indicates that the values tend to be close to the mean of the set, while a high standard deviation indicates that the values are spread out over a wider range.
An electric field is the physical field that surrounds electrically charged particles and exerts force on all other charged particles in the field, either attracting or repelling them. It also refers to the physical field for a system of charged particles. Electric fields originate from electric charges and time-varying electric currents. Electric fields and magnetic fields are both manifestations of the electromagnetic field, one of the four fundamental interactions of nature.
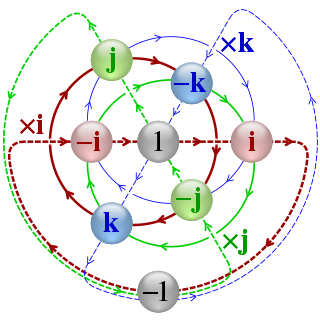
In mathematics, the quaternion number system extends the complex numbers. Quaternions were first described by the Irish mathematician William Rowan Hamilton in 1843 and applied to mechanics in three-dimensional space. Hamilton defined a quaternion as the quotient of two directed lines in a three-dimensional space, or, equivalently, as the quotient of two vectors. Multiplication of quaternions is noncommutative.
In mathematics, the Euclidean distance between two points in Euclidean space is the length of a line segment between the two points. It can be calculated from the Cartesian coordinates of the points using the Pythagorean theorem, therefore occasionally being called the Pythagorean distance. These names come from the ancient Greek mathematicians Euclid and Pythagoras, although Euclid did not represent distances as numbers, and the connection from the Pythagorean theorem to distance calculation was not made until the 18th century.
The ideal gas law, also called the general gas equation, is the equation of state of a hypothetical ideal gas. It is a good approximation of the behavior of many gases under many conditions, although it has several limitations. It was first stated by Benoît Paul Émile Clapeyron in 1834 as a combination of the empirical Boyle's law, Charles's law, Avogadro's law, and Gay-Lussac's law. The ideal gas law is often written in an empirical form:
2 (two) is a number, numeral and digit. It is the natural number following 1 and preceding 3. It is the smallest and only even prime number. Because it forms the basis of a duality, it has religious and spiritual significance in many cultures.
Exclusive or or exclusive disjunction or exclusive alternation, also known as non-equivalence which is the negation of equivalence, is a logical operation that is true if and only if its arguments differ.
Capacitance is the capability of a material object or device to store electric charge. It is measured by the charge in response to a difference in electric potential, expressed as the ratio of those quantities. Commonly recognized are two closely related notions of capacitance: self capacitance and mutual capacitance. An object that can be electrically charged exhibits self capacitance, for which the electric potential is measured between the object and ground. Mutual capacitance is measured between two components, and is particularly important in the operation of the capacitor, an elementary linear electronic component designed to add capacitance to an electric circuit.
Noether's theorem or Noether's first theorem states that every differentiable symmetry of the action of a physical system with conservative forces has a corresponding conservation law. The theorem was proven by mathematician Emmy Noether in 1915 and published in 1918. The action of a physical system is the integral over time of a Lagrangian function, from which the system's behavior can be determined by the principle of least action. This theorem only applies to continuous and smooth symmetries over physical space.
Hamiltonian mechanics emerged in 1833 as a reformulation of Lagrangian mechanics. Introduced by Sir William Rowan Hamilton, Hamiltonian mechanics replaces (generalized) velocities used in Lagrangian mechanics with (generalized) momenta. Both theories provide interpretations of classical mechanics and describe the same physical phenomena.
Electrostatics is a branch of physics that studies slow-moving or stationary electric charges.
In linear algebra, a rotation matrix is a transformation matrix that is used to perform a rotation in Euclidean space. For example, using the convention below, the matrix
In mathematical statistics, the Kullback–Leibler divergence, denoted , is a type of statistical distance: a measure of how one probability distribution P is different from a second, reference probability distribution Q. A simple interpretation of the KL divergence of P from Q is the expected excess surprise from using Q as a model when the actual distribution is P. While it is a distance, it is not a metric, the most familiar type of distance: it is not symmetric in the two distributions, and does not satisfy the triangle inequality. Instead, in terms of information geometry, it is a type of divergence, a generalization of squared distance, and for certain classes of distributions, it satisfies a generalized Pythagorean theorem.
A capacitor is a device that stores electrical energy in an electric field by accumulating electric charges on two closely spaced surfaces that are insulated from each other. It is a passive electronic component with two terminals.
In mathematics, a rational number is a number that can be expressed as the quotient or fraction of two integers, a numerator p and a non-zero denominator q. For example, is a rational number, as is every integer. The set of all rational numbers, also referred to as "the rationals", the field of rationals or the field of rational numbers is usually denoted by boldface Q, or blackboard bold
In physics, Lagrangian mechanics is a formulation of classical mechanics founded on the stationary-action principle. It was introduced by the Italian-French mathematician and astronomer Joseph-Louis Lagrange in his 1788 work, Mécanique analytique.
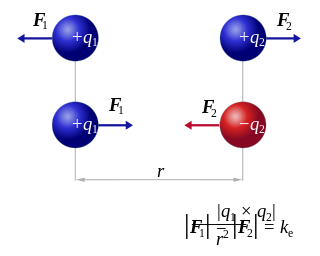
Coulomb's inverse-square law, or simply Coulomb's law, is an experimental law of physics that calculates the amount of force between two electrically charged particles at rest. This electric force is conventionally called electrostatic force or Coulomb force. Although the law was known earlier, it was first published in 1785 by French physicist Charles-Augustin de Coulomb, hence the name. Coulomb's law was essential to the development of the theory of electromagnetism and maybe even its starting point, as it allowed meaningful discussions of the amount of electric charge in a particle.