| 1987 European Athletics Indoor Championships | ||
|---|---|---|
 | ||
| Track events | ||
| 60 m | men | women |
| 200 m | men | women |
| 400 m | men | women |
| 800 m | men | women |
| 1500 m | men | women |
| 3000 m | men | women |
| 60 m hurdles | men | women |
| 3000 m walk | women | |
| 5000 m walk | men | |
| Field events | ||
| High jump | men | women |
| Pole vault | men | |
| Long jump | men | women |
| Triple jump | men | |
| Shot put | men | women |
The women's 200 metres event at the 1987 European Athletics Indoor Championships was held on 22 February. [1] [2]
| Gold | Silver | Bronze |
Kirsten Emmelmann  East Germany | Blanca Lacambra  Spain | Marie-Christine Cazier  France |
First 2 from each heat (Q) and the next 2 fastest (q) qualified for the final.
| Rank | Heat | Name | Nationality | Time | Notes |
|---|---|---|---|---|---|
| 1 | 1 | Kirsten Emmelmann | East Germany | 23.29 | Q |
| 2 | 1 | Blanca Lacambra | Spain | 23.33 | Q, NR |
| 3 | 1 | Martine Cassin | France | 24.27 | |
| 4 | 1 | Semra Aksu |  Turkey | 24.44 | |
| 5 | 1 | Marina Skourti |  Greece | 24.78 | |
| 1 | Gerda Haas |  Austria | DNS | ||
| 1 | 2 | Daniela Ferrian |  Italy | 23.67 | Q, NR |
| 2 | 2 | Marie-Christine Cazier | France | 23.75 | Q |
| 3 | 2 | Sisko Markkanen |  Finland | 24.26 | q |
| 6 | 2 | Maria Fernström |  Sweden | 23.85 | q |
| 8 | 2 | Odile Singa | France | 24.42 | |
| 2 | Ingrid Verbruggen |  Belgium | DQ | ||
| 1 | 3 | Maria Fernström | Sweden | 23.67 | Q |
| 3 | 3 | Martine Cassin | France | 23.95 |
| Rank | Name | Nationality | Time | Notes |
|---|---|---|---|---|
 | Kirsten Emmelmann | East Germany | 23.10 | |
 | Blanca Lacambra | Spain | 23.19 | |
 | Marie-Christine Cazier | France | 23.40 | |
| 4 | Daniela Ferrian | Italy | 23.57 | |
| 5 | Maria Fernström | Sweden | 24.50 | |
| 6 | Sisko Markkanen | Finland | 24.55 |
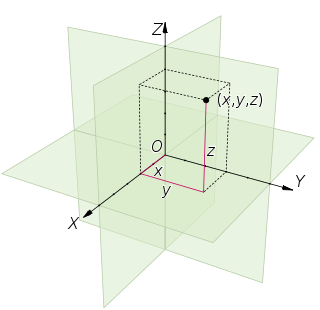
Euclidean space is the fundamental space of geometry, intended to represent physical space. Originally, in Euclid's Elements, it was the three-dimensional space of Euclidean geometry, but in modern mathematics there are Euclidean spaces of any positive integer dimension n, which are called Euclidean n-spaces when one wants to specify their dimension. For n equal to one or two, they are commonly called respectively Euclidean lines and Euclidean planes. The qualifier "Euclidean" is used to distinguish Euclidean spaces from other spaces that were later considered in physics and modern mathematics.
In geometry, a Platonic solid is a convex, regular polyhedron in three-dimensional Euclidean space. Being a regular polyhedron means that the faces are congruent regular polygons, and the same number of faces meet at each vertex. There are only five such polyhedra:
The modularity theorem states that elliptic curves over the field of rational numbers are related to modular forms in a particular way. Andrew Wiles and Richard Taylor proved the modularity theorem for semistable elliptic curves, which was enough to imply Fermat's Last Theorem. Later, a series of papers by Wiles's former students Brian Conrad, Fred Diamond and Richard Taylor, culminating in a joint paper with Christophe Breuil, extended Wiles's techniques to prove the full modularity theorem in 2001.
In differential geometry, a pseudo-Riemannian manifold, also called a semi-Riemannian manifold, is a differentiable manifold with a metric tensor that is everywhere nondegenerate. This is a generalization of a Riemannian manifold in which the requirement of positive-definiteness is relaxed.
In mathematics, a binary operation is commutative if changing the order of the operands does not change the result. It is a fundamental property of many binary operations, and many mathematical proofs depend on it. Perhaps most familiar as a property of arithmetic, e.g. "3 + 4 = 4 + 3" or "2 × 5 = 5 × 2", the property can also be used in more advanced settings. The name is needed because there are operations, such as division and subtraction, that do not have it ; such operations are not commutative, and so are referred to as noncommutative operations. The idea that simple operations, such as the multiplication and addition of numbers, are commutative was for many years implicitly assumed. Thus, this property was not named until the 19th century, when mathematics started to become formalized. A similar property exists for binary relations; a binary relation is said to be symmetric if the relation applies regardless of the order of its operands; for example, equality is symmetric as two equal mathematical objects are equal regardless of their order.
In mathematics, there are several equivalent ways of defining the real numbers. One of them is that they form a complete ordered field that does not contain any smaller complete ordered field. Such a definition does not prove that such a complete ordered field exists, and the existence proof consists of constructing a mathematical structure that satisfies the definition.
In geometry, a star polygon is a type of non-convex polygon. Regular star polygons have been studied in depth; while star polygons in general appear not to have been formally defined, certain notable ones can arise through truncation operations on regular simple or star polygons.
John Sherwood de Lancie, Jr. is an American actor. He is best known for his role as Q in various Star Trek series, beginning with Star Trek: The Next Generation in 1987 and leading up to the third season of Star Trek: Picard in 2023.
Paul Antony Young is an English musician, singer and songwriter. Formerly the frontman of the short-lived bands Kat Kool & the Kool Cats, Streetband and Q-Tips, he became a teen idol with his solo success in the 1980s. His hit singles include "Love of the Common People", "Wherever I Lay My Hat", "Come Back and Stay", "Every Time You Go Away" and "Everything Must Change", all reaching the top 10 of the UK Singles Chart. Released in 1983, his debut album, No Parlez, was the first of three UK number-one albums.
In economics, nominalvalue refers to value measured in terms of absolute money amounts, whereas real value is considered and measured against the actual goods or services for which it can be exchanged at a given time. Real value takes into account inflation and the value of an asset in relation to its purchasing power.
In linear algebra, a rotation matrix is a transformation matrix that is used to perform a rotation in Euclidean space. For example, using the convention below, the matrix
The UEFA European Under-17 Championship or simply UEFA Under-17 Championship is an annual football competition contested by the European men's under-17 national teams of the member associations of UEFA.
Xamtanga is a Central Cushitic language spoken in Ethiopia by the Xamir people.
In geometry, a polytope or a tiling is isotoxal or edge-transitive if its symmetries act transitively on its edges. Informally, this means that there is only one type of edge to the object: given two edges, there is a translation, rotation, and/or reflection that will move one edge to the other while leaving the region occupied by the object unchanged.
In mathematics, the Lehmer mean of a tuple of positive real numbers, named after Derrick Henry Lehmer, is defined as:
The men's 60 metres event at the 1987 European Athletics Indoor Championships was held on 21 February.
The men's 60 metres hurdles event at the 1987 European Athletics Indoor Championships was held on 22 February.
The women's 400 metres event at the 1987 European Athletics Indoor Championships was held on 21 and 22 February.
The men's 800 metres event at the 1987 European Athletics Indoor Championships was held on 21 and 22 February.
Results of India national football team from 1980 to 1989.
European Athletics Indoor Champions in women's 200 metres | |
|---|---|
|
