Duopyramid

Last updated February 28, 2023

In geometry of 4 dimensions or higher, a double pyramid or duopyramid or fusil is a polytope constructed by 2 orthogonal polytopes with edges connecting all pairs of vertices between the two. The term fusil is used by Norman Johnson as a rhombic-shape.^[1] The term duopyramid was used by George Olshevsky, as the dual of a duoprism.^[2]

Polygonal forms

Set of dual uniform p-q duopyramids
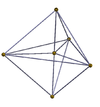
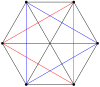
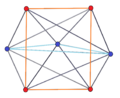
Example 4-4 duopyramid (16-cell) Orthogonal projection
Type	Uniform dual polychoron
Schläfli symbol	{p} + {q}^[3]
Coxeter diagram
Cells	pq digonal disphenoids
Faces	2pq triangles
Edges	pq+p+q
Vertices	p+q
Vertex figures	p-gonal bipyramid q-gonal bipyramid
Symmetry	[p,2,q], order 4pq
Dual	p-q duoprism
Properties	convex, facet-transitive

Set of dual uniform p-p duopyramids
Schläfli symbol	{p} + {p} = 2{p}
Coxeter diagram
Cells	p² tetragonal disphenoids
Faces	2p² triangles
Edges	p²+2p
Vertices	2p
Vertex figure	p-gonal bipyramid
Symmetry	[[p,2,p]] = [2p,2⁺,2p], order 8p²
Dual	p-p duoprism
Properties	convex, facet-transitive

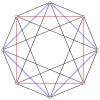
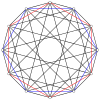
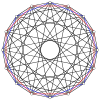
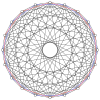
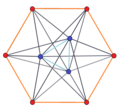
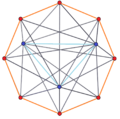
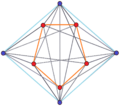
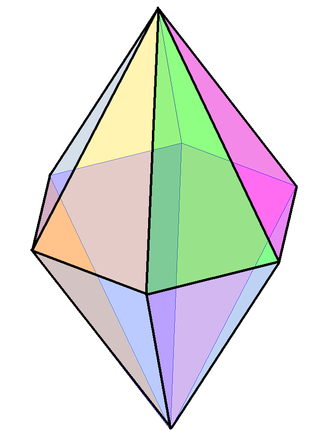
The lowest dimensional forms are 4 dimensional and connect two polygons. A p-q duopyramid or p-q fusil, represented by a composite Schläfli symbol {p} + {q}, and Coxeter-Dynkin diagram . The regular 16-cell can be seen as a 4-4 duopyramid or 4-4 fusil, , symmetry [[4,2,4]], order 128.

A p-q duopyramid or p-q fusil has Coxeter group symmetry [p,2,q], order 4pq. When p and q are identical, the symmetry in Coxeter notation is doubled as [[p,2,p]] or [2p,2⁺,2q], order 8p².

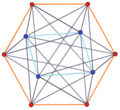
Edges exist on all pairs of vertices between the p-gon and q-gon. The 1-skeleton of a p-q duopyramid represents edges of each p and q polygon and pq complete bipartite graph between them.

Geometry

A p-q duopyramid can be seen as two regular planar polygons of p and q sides with the same center and orthogonal orientations in 4 dimensions. Along with the p and q edges of the two polygons, all permutations of vertices in one polygon to vertices in the other form edges. All faces are triangular, with one edge of one polygon connected to one vertex of the other polygon. The p and q sided polygons are hollow, passing through the polytope center and not defining faces. Cells are tetrahedra constructed as all permutations of edge pairs between each polygon.

It can be understood by analogy to the relation of the 3D prisms and their dual bipyramids with Schläfli symbol { } + {p}, and a rhombus in 2D as { } + { }. A bipyramid can be seen as a 3D degenerated duopyramid, by adding an edge across the digon { } on the inner axis, and adding intersecting interior triangles and tetrahedra connecting that new edge to p-gon vertices and edges.

Other nonuniform polychora can be called duopyramids by the same construction, as two orthogonal and co-centered polygons, connected with edges with all combinations of vertex pairs between the polygons. The symmetry will be the product of the symmetry of the two polygons. So a rectangle-rectangle duopyramid would be topologically identical to the uniform 4-4 duopyramid, but a lower symmetry [2,2,2], order 16, possibly doubled to 32 if the two rectangles are identical.

Coordinates

The coordinates of a p-q duopyramid (on a unit 3-sphere) can be given as:

(\cos {\frac {2\pi i}{p}},\sin {\frac {2\pi i}{p}},0,0),\quad i=1\dots p

(0,0,\cos {\frac {2\pi j}{q}},\sin {\frac {2\pi j}{q}}),\quad j=1\dots q

All pairs of vertices are connected by edges.

Perspective projections

3-3	3-4	4-4 (16-cell)

Orthogonal projections

The 2n vertices of a n-n duopyramid can be orthogonally projected into two regular n-gons with edges between all vertices of each n-gon.

The regular 16-cell can be seen as a 4-4 duopyramid, being dual to the 4-4 duoprism, which is the tesseract. As a 4-4 duopyramid, the 16-cell's symmetry is [4,2,4], order 64, and doubled to [[4,2,4]], order 128 with the 2 central squares interchangeable. The regular 16-cell has a higher symmetry [3,3,4], order 384.

p-p duopyramids
3-3	5-5	7-7	9-9	11-11	13-13	15-15	17-17	19-19
4-4 (16-cell)	6-6	8-8	10-10	12-12	14-14	16-16	18-18	20-20

p-q duopyramids
3-4	3-5	3-6	3-8
4-5	4-6

Example 6-4 duopyramid

	This vertex-centered stereographic projection of 6-4 duopyramid (blue) with its dual duoprism (in transparent red). In the last row, the duopyramid is projected by a direction perpendicular to the first one; so the two parameters (6,4) seem to be reversed. Indeed, asymmetry is due to the projection: the two parameters are symmetric in 4D.

Related Research Articles

In geometry, an $n$ -gonal antiprism or $n$ -antiprism is a polyhedron composed of two parallel direct copies of an $n$ -sided polygon, connected by an alternating band of $2 n$ triangles. They are represented by the Conway notation $A n$ .

A (symmetric) $n$ -gonal bipyramid or dipyramid is a polyhedron formed by joining an $n$ -gonal pyramid and its mirror image base-to-base. An $n$ -gonal bipyramid has $2 n$ triangle faces, $3 n$ edges, and $2 + n$ vertices.

In geometry, a polygon is a plane figure that is described by a finite number of straight line segments connected to form a closed polygonal chain. The bounded plane region, the bounding circuit, or the two together, may be called a polygon.

In geometry, a Platonic solid is a convex, regular polyhedron in three-dimensional Euclidean space. Being a regular polyhedron means that the faces are congruent regular polygons, and the same number of faces meet at each vertex. There are only five such polyhedra:

<span class="mw-page-title-main">Tetrahedron</span> Polyhedron with 4 faces

In geometry, a tetrahedron, also known as a triangular pyramid, is a polyhedron composed of four triangular faces, six straight edges, and four vertex corners. The tetrahedron is the simplest of all the ordinary convex polyhedra.

In geometry, a heptadecagon, septadecagon or 17-gon is a seventeen-sided polygon.

In geometry, a prism is a polyhedron comprising an $n$ -sided polygon base, a second base which is a translated copy of the first, and $n$ other faces, necessarily all parallelograms, joining corresponding sides of the two bases. All cross-sections parallel to the bases are translations of the bases. Prisms are named after their bases, e.g. a prism with a pentagonal base is called a pentagonal prism. Prisms are a subclass of prismatoids.

<span class="mw-page-title-main">Schläfli symbol</span> Notation that defines regular polytopes and tessellations

In geometry, the Schläfli symbol is a notation of the form $that defines regular polytopes and tessellations.$

In geometry, a dodecagon or 12-gon is any twelve-sided polygon.

<span class="mw-page-title-main">Cross-polytope</span> Regular polytope dual to the hypercube in any number of dimensions

In geometry, a cross-polytope, hyperoctahedron, orthoplex, or cocube is a regular, convex polytope that exists in n-dimensional Euclidean space. A 2-dimensional cross-polytope is a square, a 3-dimensional cross-polytope is a regular octahedron, and a 4-dimensional cross-polytope is a 16-cell. Its facets are simplexes of the previous dimension, while the cross-polytope's vertex figure is another cross-polytope from the previous dimension.

In geometry, a triacontagon or 30-gon is a thirty-sided polygon. The sum of any triacontagon's interior angles is 5040 degrees.

In geometry, a uniform 4-polytope is a 4-dimensional polytope which is vertex-transitive and whose cells are uniform polyhedra, and faces are regular polygons.

In geometry of 4 dimensions or higher, a double prism or duoprism is a polytope resulting from the Cartesian product of two polytopes, each of two dimensions or higher. The Cartesian product of an $n$ -polytope and an $m$ -polytope is an $(n + m)$ -polytope, where $n$ and $m$ are dimensions of 2 (polygon) or higher.

In geometry, a skew polygon is a polygon whose vertices are not all coplanar. Skew polygons must have at least four vertices. The interior surface of such a polygon is not uniquely defined.

In geometry, a complex polytope is a generalization of a polytope in real space to an analogous structure in a complex Hilbert space, where each real dimension is accompanied by an imaginary one.

<span class="mw-page-title-main">Regular 4-polytope</span> Four-dimensional analogues of the regular polyhedra in three dimensions

In mathematics, a regular 4-polytope is a regular four-dimensional polytope. They are the four-dimensional analogues of the regular polyhedra in three dimensions and the regular polygons in two dimensions.

In geometry, the regular skew polyhedra are generalizations to the set of regular polyhedra which include the possibility of nonplanar faces or vertex figures. Coxeter looked at skew vertex figures which created new 4-dimensional regular polyhedra, and much later Branko Grünbaum looked at regular skew faces.

In the geometry of 4 dimensions, the 3-3 duoprism or triangular duoprism is a four-dimensional convex polytope. It can be constructed as the Cartesian product of two triangles and is the simplest of an infinite family of four-dimensional polytopes constructed as Cartesian products of two polygons, the duoprisms.

In geometry, a regular complex polygon is a generalization of a regular polygon in real space to an analogous structure in a complex Hilbert space, where each real dimension is accompanied by an imaginary one. A regular polygon exists in 2 real dimensions, $, while a complex polygon exists in two complex dimensions,, which can be given real representations in 4 dimensions,, which then must be projected down to 2 or 3 real dimensions to be visualized. A complex polygon is generalized as a complex polytope in .$

References

↑ Norman W. Johnson, Geometries and Transformations (2018), p.167
↑ Olshevsky, George. "Duopyramid". Glossary for Hyperspace. Archived from the original on 4 February 2007.
↑ N.W. Johnson: Geometries and Transformations, (2018) ISBN 978-1-107-10340-5 Chapter 11: Finite symmetry groups, 11.5 Spherical Coxeter groups, p.251

This page is based on this Wikipedia article
Text is available under the CC BY-SA 4.0 license; additional terms may apply.
Images, videos and audio are available under their respective licenses.

[1] Norman W. Johnson, Geometries and Transformations (2018), p.167

[2] Olshevsky, George. "Duopyramid". Glossary for Hyperspace. Archived from the original on 4 February 2007.

[3] N.W. Johnson: Geometries and Transformations, (2018) ISBN 978-1-107-10340-5 Chapter 11: Finite symmetry groups, 11.5 Spherical Coxeter groups, p.251

[1]

[2]

[3]