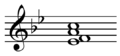In music theory, an interval is a difference in pitch between two sounds. An interval may be described as horizontal, linear, or melodic if it refers to successively sounding tones, such as two adjacent pitches in a melody, and vertical or harmonic if it pertains to simultaneously sounding tones, such as in a chord.
An altered chord is a chord that replaces one or more notes from the diatonic scale with a neighboring pitch from the chromatic scale. By the broadest definition, any chord with a non-diatonic chord tone is an altered chord. The simplest example of altered chords is the use of borrowed chords, chords borrowed from the parallel key, and the most common is the use of secondary dominants. As Alfred Blatter explains, "An altered chord occurs when one of the standard, functional chords is given another quality by the modification of one or more components of the chord."

A chord, in music, is any harmonic set of pitches/frequencies consisting of multiple notes that are heard as if sounding simultaneously. For many practical and theoretical purposes, arpeggios and other types of broken chords may also be considered as chords in the right musical context.

In music theory, the concept of root is the idea that a chord can be represented and named by one of its notes. It is linked to harmonic thinking—the idea that vertical aggregates of notes can form a single unit, a chord. It is in this sense that one speaks of a "C chord" or a "chord on C"—a chord built from C and of which the note C is the root. When a chord is referred to in Classical music or popular music without a reference to what type of chord it is, it is assumed a major triad, which for C contains the notes C, E and G. The root need not be the bass note, the lowest note of the chord: the concept of root is linked to that of the inversion of chords, which is derived from the notion of invertible counterpoint. In this concept, chords can be inverted while still retaining their root.
In music theory, an augmented sixth chord contains the interval of an augmented sixth, usually above its bass tone. This chord has its origins in the Renaissance, was further developed in the Baroque, and became a distinctive part of the musical style of the Classical and Romantic periods.

In music, extended chords are certain chords or triads with notes extended, or added, beyond the seventh. Ninth, eleventh, and thirteenth chords are extended chords. The thirteenth is the farthest extension diatonically possible as, by that point, all seven tonal degrees are represented within the chord. In practice however, extended chords do not typically use all the chord members; when it is not altered, the fifth is often omitted, as are notes between the seventh and the highest note, unless they are altered to give a special texture.
The term sixth chord refers to two different kinds of chord, the first in classical music and the second in modern popular music.

In music or music theory, a thirteenth is the note thirteen scale degrees from the root of a chord and also the interval between the root and the thirteenth. The thirteenth is most commonly major Play (help·info) or minor Play (help·info).
In music theory, a ninth chord is a chord that encompasses the interval of a ninth when arranged in close position with the root in the bass.
The ninth chord and its inversions exist today, or at least they can exist. The pupil will easily find examples in the literature [such as Schoenberg's Verklärte Nacht and Strauss's opera Salome]. It is not necessary to set up special laws for its treatment. If one wants to be careful, one will be able to use the laws that pertain to the seventh chords: that is, dissonances resolve by step downward, the root leaps a fourth upward.
The diminished seventh chord is a four-note chord composed of a root note, together with a minor third, a diminished fifth, and a diminished seventh above the root:. For example, the diminished seventh chord built on C, commonly written as Co7, has pitches C–E♭–G♭–B :

The root position of a chord is the voicing of a triad, seventh chord, or ninth chord in which the root of the chord is the bass note and the other chord factors are above it. In the root position, uninverted, of a C-major triad, the bass is C — the root of the triad — with the third and the fifth stacked above it, forming the intervals of a third and a fifth above the root of C, respectively.

In music, a guitar chord is a set of notes played on a guitar. A chord's notes are often played simultaneously, but they can be played sequentially in an arpeggio. The implementation of guitar chords depends on the guitar tuning. Most guitars used in popular music have six strings with the "standard" tuning of the Spanish classical guitar, namely E–A–D–G–B–E' ; in standard tuning, the intervals present among adjacent strings are perfect fourths except for the major third (G,B). Standard tuning requires four chord-shapes for the major triads.
Jazz chords are chords, chord voicings and chord symbols that jazz musicians commonly use in composition, improvisation, and harmony. In jazz chords and theory, most triads that appear in lead sheets or fake books can have sevenths added to them, using the performer's discretion and ear. For example, if a tune is in the key of C, if there is a G chord, the chord-playing performer usually voices this chord as G7. While the notes of a G7 chord are G–B–D–F, jazz often omits the fifth of the chord—and even the root if playing in a group. However, not all jazz pianists leave out the root when they play voicings: Bud Powell, one of the best-known of the bebop pianists, and Horace Silver, whose quintet included many of jazz's biggest names from the 1950s to the 1970s, included the root note in their voicings.

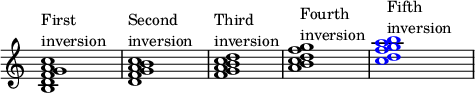
The second inversion of a chord is the voicing of a triad, seventh chord, or ninth chord in which the fifth of the chord is the bass note. In this inversion, the bass note and the root of the chord are a fourth apart which traditionally qualifies as a dissonance. There is therefore a tendency for movement and resolution. In notation form, it is referred to with a c following the chord position. In figured bass, a second-inversion triad is a 6
4 chord, while a second-inversion seventh chord is a 4
3 chord.
Inversions are not restricted to the same number of tones as the original chord, nor to any fixed order of tones except with regard to the interval between the root, or its octave, and the bass note, hence, great variety results.

The first inversion of a chord is the voicing of a triad, seventh chord, or ninth chord in which the third of the chord is the bass note and the root a sixth above it. In the first inversion of a C-major triad, the bass is E — the third of the triad — with the fifth and the root stacked above it, forming the intervals of a minor third and a minor sixth above the inverted bass of E, respectively.
In music theory, an inversion is a type of change to intervals, chords, voices, and melodies. In each of these cases, "inversion" has a distinct but related meaning. The concept of inversion also plays an important role in musical set theory.

In music, the seventh factor of a chord is the note or pitch seven scale degrees above the root or tonal center. When the seventh is the bass note, or lowest note, of the expressed chord, the chord is in third inversion Play (help·info).

In music, a factor or chord factor is a member or component of a chord. These are named root, third, fifth, sixth, seventh, ninth, eleventh, thirteenth, and so on, for their generic interval above the root. In harmony, the consonance and dissonance of a chord factor and a nonchord tone are distinguished, respectively.
Musicians use various kinds of chord names and symbols in different contexts to represent musical chords. In most genres of popular music, including jazz, pop, and rock, a chord name and its corresponding symbol typically indicate one or more of the following:
- the root note,
- the chord quality,
- whether the chord is a triad, seventh chord, or an extended chord,
- any altered notes,
- any added tones, and
- the bass note if it is not the root.

The third inversion of a seventh chord is the voicing in which the seventh of the chord is the bass note and the root a major second above it. In the third inversion of a G-dominant seventh chord, the bass is F — the seventh of the chord — with the root, third, and fifth stacked above it, forming the intervals of a second, a fourth, and a sixth above the inverted bass of F, respectively. In figured bass, it is referred to as a 4
2 chord.