Related Research Articles
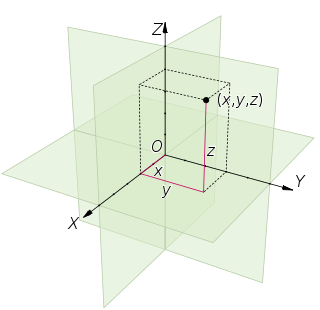
Euclidean space is the fundamental space of geometry, intended to represent physical space. Originally, that is, in Euclid's Elements, it was the three-dimensional space of Euclidean geometry, but in modern mathematics there are Euclidean spaces of any positive integer dimension n, which are called Euclidean n-spaces when one wants to specify their dimension. For n equal to one or two, they are commonly called respectively Euclidean lines and Euclidean planes. The qualifier "Euclidean" is used to distinguish Euclidean spaces from other spaces that were later considered in physics and modern mathematics.

In mathematics, a metric space is a set together with a notion of distance between its elements, usually called points. The distance is measured by a function called a metric or distance function. Metric spaces are the most general setting for studying many of the concepts of mathematical analysis and geometry.

In group theory, the symmetry group of a geometric object is the group of all transformations under which the object is invariant, endowed with the group operation of composition. Such a transformation is an invertible mapping of the ambient space which takes the object to itself, and which preserves all the relevant structure of the object. A frequent notation for the symmetry group of an object X is G = Sym(X).

In mathematics, hyperbolic geometry is a non-Euclidean geometry. The parallel postulate of Euclidean geometry is replaced with:
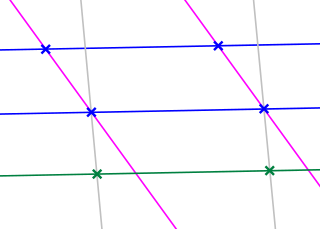
In mathematics, affine geometry is what remains of Euclidean geometry when ignoring the metric notions of distance and angle.

In mathematics, the open unit disk around P, is the set of points whose distance from P is less than 1:
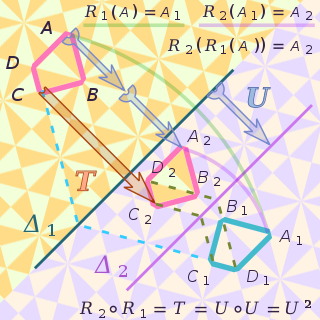
In mathematics, an isometry is a distance-preserving transformation between metric spaces, usually assumed to be bijective. The word isometry is derived from the Ancient Greek: ἴσος isos meaning "equal", and μέτρον metron meaning "measure".
In mathematics, conformal geometry is the study of the set of angle-preserving (conformal) transformations on a space.

In mathematics, hyperbolic space of dimension n is the unique simply connected, n-dimensional Riemannian manifold of constant sectional curvature equal to -1. It is homogeneous, and satisfies the stronger property of being a symmetric space. There are many ways to construct it as an open subset of with an explicitly written Riemannian metric; such constructions are referred to as models. Hyperbolic 2-space, H2, which was the first instance studied, is also called the hyperbolic plane.

A tessellation or tiling is the covering of a surface, often a plane, using one or more geometric shapes, called tiles, with no overlaps and no gaps. In mathematics, tessellation can be generalized to higher dimensions and a variety of geometries.

In mathematics, the conformal group of an inner product space is the group of transformations from the space to itself that preserve angles. More formally, it is the group of transformations that preserve the conformal geometry of the space.

In mathematics, a topological group G is called a discrete group if there is no limit point in it. Equivalently, the group G is discrete if and only if its identity is isolated.

In mathematics, low-dimensional topology is the branch of topology that studies manifolds, or more generally topological spaces, of four or fewer dimensions. Representative topics are the structure theory of 3-manifolds and 4-manifolds, knot theory, and braid groups. This can be regarded as a part of geometric topology. It may also be used to refer to the study of topological spaces of dimension 1, though this is more typically considered part of continuum theory.

In mathematics, a Euclidean group is the group of (Euclidean) isometries of a Euclidean space ; that is, the transformations of that space that preserve the Euclidean distance between any two points (also called Euclidean transformations). The group depends only on the dimension n of the space, and is commonly denoted E(n) or ISO(n).
In geometry, hyperbolic motions are isometric automorphisms of a hyperbolic space. Under composition of mappings, the hyperbolic motions form a continuous group. This group is said to characterize the hyperbolic space. Such an approach to geometry was cultivated by Felix Klein in his Erlangen program. The idea of reducing geometry to its characteristic group was developed particularly by Mario Pieri in his reduction of the primitive notions of geometry to merely point and motion.

In geometry, the hyperboloid model, also known as the Minkowski model after Hermann Minkowski, is a model of n-dimensional hyperbolic geometry in which points are represented by points on the forward sheet S+ of a two-sheeted hyperboloid in (n+1)-dimensional Minkowski space or by the displacement vectors from the origin to those points, and m-planes are represented by the intersections of (m+1)-planes passing through the origin in Minkowski space with S+ or by wedge products of m vectors. Hyperbolic space is embedded isometrically in Minkowski space; that is, the hyperbolic distance function is inherited from Minkowski space, analogous to the way spherical distance is inherited from Euclidean distance when the n-sphere is embedded in (n+1)-dimensional Euclidean space.
In geometry, the Beckman–Quarles theorem states that if a transformation of the Euclidean plane or a higher-dimensional Euclidean space preserves unit distances, then it preserves all Euclidean distances. Equivalently, every homomorphism from the unit distance graph of the plane to itself must be an isometry of the plane. The theorem is named after Frank S. Beckman and Donald A. Quarles Jr., who published this result in 1953; it was later rediscovered by other authors and re-proved in multiple ways. Analogous theorems for rational subsets of Euclidean spaces, or for non-Euclidean geometry, are also known.
In mathematics—specifically, in differential geometry—a geodesic map (or geodesic mapping or geodesic diffeomorphism) is a function that "preserves geodesics". More precisely, given two (pseudo-)Riemannian manifolds (M, g) and (N, h), a function φ : M → N is said to be a geodesic map if

Geometry is a branch of mathematics concerned with properties of space such as the distance, shape, size, and relative position of figures. Geometry is, along with arithmetic, one of the oldest branches of mathematics. A mathematician who works in the field of geometry is called a geometer. Until the 19th century, geometry was almost exclusively devoted to Euclidean geometry, which includes the notions of point, line, plane, distance, angle, surface, and curve, as fundamental concepts.
In geometry, an object has symmetry if there is an operation or transformation that maps the figure/object onto itself. Thus, a symmetry can be thought of as an immunity to change. For instance, a circle rotated about its center will have the same shape and size as the original circle, as all points before and after the transform would be indistinguishable. A circle is thus said to be symmetric under rotation or to have rotational symmetry. If the isometry is the reflection of a plane figure about a line, then the figure is said to have reflectional symmetry or line symmetry; it is also possible for a figure/object to have more than one line of symmetry.
References
- ↑ Roman, Steven (2008), Advanced Linear Algebra, Graduate Texts in Mathematics (Third ed.), Springer, p. 271, ISBN 978-0-387-72828-5 .
- ↑ Burago, Dmitri; Burago, Yuri; Ivanov, Sergei (2001), A course in metric geometry, Graduate Studies in Mathematics, vol. 33, Providence, RI: American Mathematical Society, p. 75, ISBN 0-8218-2129-6, MR 1835418 .
- ↑ Berger, Marcel (1987), Geometry. II, Universitext, Berlin: Springer-Verlag, p. 281, doi:10.1007/978-3-540-93816-3, ISBN 3-540-17015-4, MR 0882916 .
- ↑ Olver, Peter J. (1999), Classical invariant theory, London Mathematical Society Student Texts, vol. 44, Cambridge: Cambridge University Press, p. 53, doi:10.1017/CBO9780511623660, ISBN 0-521-55821-2, MR 1694364 .
- ↑ Müller-Kirsten, Harald J. W.; Wiedemann, Armin (2010), Introduction to supersymmetry, World Scientific Lecture Notes in Physics, vol. 80 (2nd ed.), Hackensack, NJ: World Scientific Publishing Co. Pte. Ltd., p. 22, doi:10.1142/7594, ISBN 978-981-4293-42-6, MR 2681020 .